Statement 1 : A bullet is fired and it hits a target. An observer in the same plane heard two sounds: the crack of the rifle and the thud of the bullet striking the target at the same instant. Then the locus of the observer is a hyperbola where the velocity of sound is smaller than the velocity of the bullet. Statement 2 : If the difference of distances of a point P from two fixed points is constant and less than the distance between the fixed points, then the locus of P is a hyperbola.
To solve the given problem, we need to analyze both statements and derive the necessary conclusions step by step.
2. Defining Variables:
- Let P be the point where the gun is fired.
- Let Q be the target where the bullet hits.
- Let R be the position of the observer.
- Let u be the velocity of sound.
- Let v be the velocity of the bullet.
3. Time Analysis:
- Let t1 be the time taken for the observer to hear the sound of the gunshot.
- Let t2 be the time taken for the bullet to reach the target.
- Let t3 be the time taken for the sound of the bullet hitting the target to reach the observer.
- According to the problem, t1=t2+t3.
4. Distance Relationships:
- The distance from the observer to the gunshot is PR.
- The distance from the bullet to the target is PQ.
- The distance from the target to the observer is QR.
5. Using the Speed Formula:
- The distance can be expressed as:
PR=u⋅t1
PQ=v⋅t2
QR=u⋅t3
6. Substituting Time Relationships:
- From t1=t2+t3, we can derive:
PR=u(t2+t3)=u⋅t2+u⋅t3
- Thus, we can write:
PR=u⋅t2+QR
7. Rearranging the Equation:
- Rearranging gives:
PR−QR=u⋅t2
- This implies the difference in distances is proportional to the time taken and the velocities involved.
8. Conclusion for Statement 1:
- Since the velocity of the bullet v is greater than the velocity of sound u, the locus of the observer R forms a hyperbola.
Statement 2:
1. Understanding the Definition of Hyperbola:
- A hyperbola is defined as the locus of points P such that the absolute difference of the distances from two fixed points (foci) is constant.
2. Given Condition:
- If the difference of distances from point P to two fixed points F1 and F2 is constant and less than the distance between F1 and F2, then the locus of P is indeed a hyperbola.
3. Conclusion for Statement 2:
- This statement is true as it directly follows the definition of a hyperbola.
Final Conclusion:
Both statements are true. The first statement describes a scenario leading to a hyperbola based on the relationship between the velocities of the bullet and sound, while the second statement is a direct application of the definition of a hyperbola.
- Let P be the point where the gun is fired.
- Let Q be the target where the bullet hits.
- Let R be the position of the observer.
- Let u be the velocity of sound.
- Let v be the velocity of the bullet.
- Let t1 be the time taken for the observer to hear the sound of the gunshot.
- Let t2 be the time taken for the bullet to reach the target.
- Let t3 be the time taken for the sound of the bullet hitting the target to reach the observer.
- According to the problem, t1=t2+t3.
- The distance from the observer to the gunshot is PR.
- The distance from the bullet to the target is PQ.
- The distance from the target to the observer is QR.
- The distance can be expressed as:
PR=u⋅t1
PQ=v⋅t2
QR=u⋅t3
- From t1=t2+t3, we can derive:
PR=u(t2+t3)=u⋅t2+u⋅t3
- Thus, we can write:
PR=u⋅t2+QR
- Rearranging gives:
PR−QR=u⋅t2
- This implies the difference in distances is proportional to the time taken and the velocities involved.
- Since the velocity of the bullet v is greater than the velocity of sound u, the locus of the observer R forms a hyperbola.
1. Understanding the Definition of Hyperbola:
- A hyperbola is defined as the locus of points P such that the absolute difference of the distances from two fixed points (foci) is constant.
- If the difference of distances from point P to two fixed points F1 and F2 is constant and less than the distance between F1 and F2, then the locus of P is indeed a hyperbola.
- This statement is true as it directly follows the definition of a hyperbola.
Both statements are true. The first statement describes a scenario leading to a hyperbola based on the relationship between the velocities of the bullet and sound, while the second statement is a direct application of the definition of a hyperbola.
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions886videos
COMPLEX NUMBERS AND QUADRATIC EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
Similar Questions
A large number of bullets are fired in all directions with the same speed v. Find the maximum area on the ground on which these bullets will spread.
View SolutionA bulley with muzzle velocity 100ms−1 is to be shot at a target 30m away in the same horizontal line. How high above the target must the rifle be aimed so that the bullet will hit the target ?
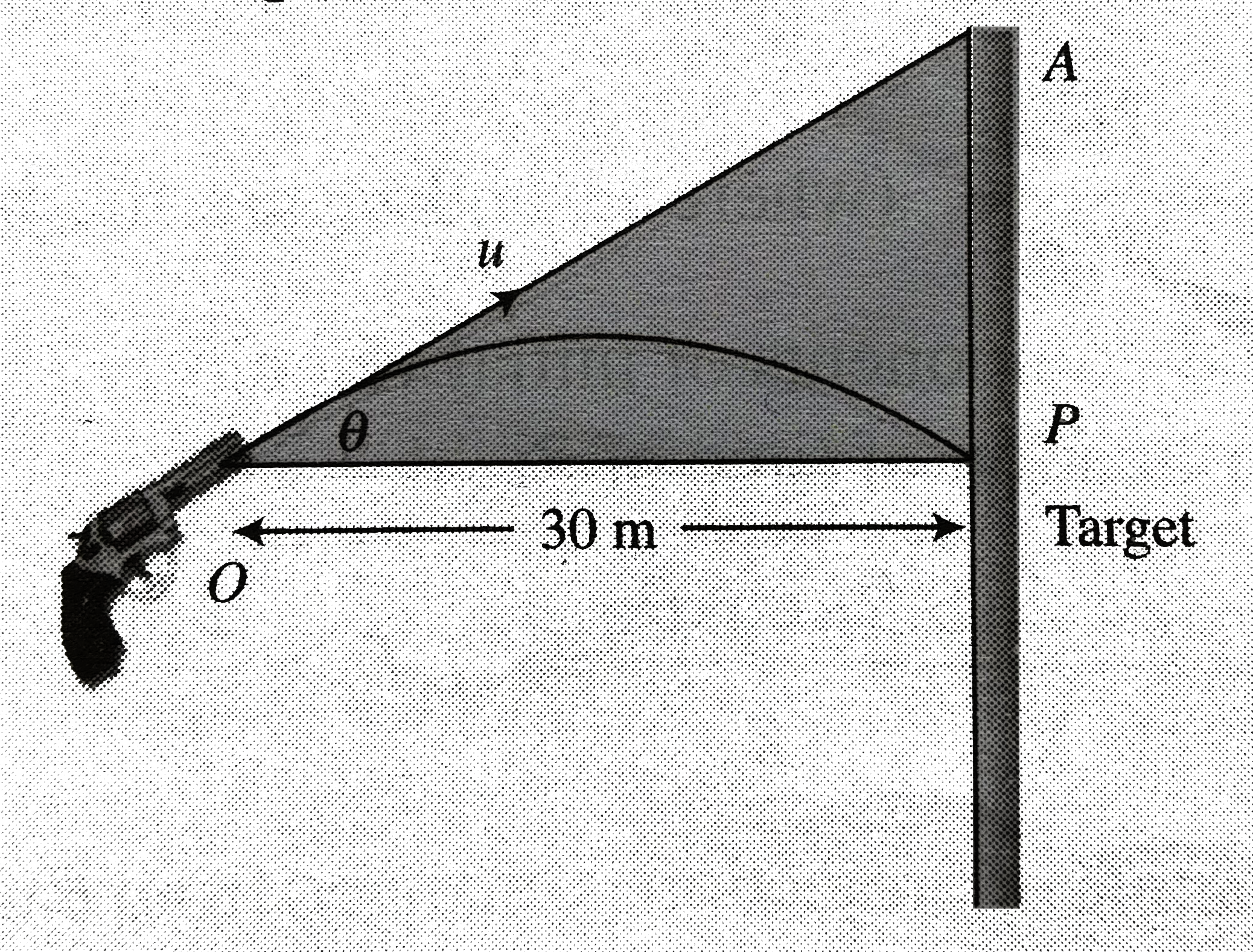 .View Solution
.View Solution
Knowledge Check
- Question 1 - Select One
Assertion : The sound emitted by the source travels in all directions.
Reason : The relative velocity of sound with respect to the observer is the sum of velocity of sound and velocity of observer.AIf both assertion and reason are true and reason is the correct explanation of assertion.BIf both assertion and reason are true but reason is not the correct explanation of assertion.CIf assertion is true but reason is false.DIf both assertion and reason are false. - Question 2 - Select One
A large number of bullets are fired in all directions with the 'same speed v∘. The maximum area on the ground on which these bullets will spread is
Aπv2gBπv4g2Cπ2v2g2Dπ2v4g2
A bullet is fired horizontally aiming at an object which starts falling at the instant the bullet is fired. Show that the bullet will hit the object.
View SolutionWhen a bullet is fired from a gun
View SolutionWhen a bullet is fired from a gun
View SolutionAssertion : A bullet is fired from a rifle. If the rifle recoils freely, the kinetic energy of rifle is more than that of the bullet.
Reason : In the case of rifle bullet system the law of conservation of momentum violates.View SolutionA bullet of mass m hits a target of mass M hanging by a string and gets embedded in it. If the block rises to a height h as a result of this collision, the velocity of the bullet before collision is
View SolutionA bullet of mass m is fired into a block of wood of mass M which hangs on the end of pendulum and gets embedded into it. When the bullet strikes with maximum rise R. Then, the velocity of the bullet is given by
View Solution

