Two circles with equal radii are intersecting at the points (0, 1) and (0,-1). The tangent at the point (0,1) to one of the circles passes through the centre of the other circle. Then the distance between the centres of these circles is.
1
√2
2√2
2
The correct Answer is:D
To solve the problem, we need to find the distance between the centers of two intersecting circles that share the same radius and intersect at the points (0, 1) and (0, -1).
1. Define the Circles:
Let the centers of the two circles be (a,b) and (c,d) with equal radius r. The equations of the circles can be written as:
(x−a)2+(y−b)2=r2(Circle 1)
(x−c)2+(y−d)2=r2(Circle 2)
2. Substituting Intersection Points:
Since both circles intersect at the points (0, 1) and (0, -1), we can substitute these points into the equations of both circles.
For Circle 1 at (0, 1):
(0−a)2+(1−b)2=r2(1)
For Circle 1 at (0, -1):
(0−a)2+(−1−b)2=r2(2)
For Circle 2 at (0, 1):
(0−c)2+(1−d)2=r2(3)
For Circle 2 at (0, -1):
(0−c)2+(−1−d)2=r2(4)
3. Equating the Radius Expressions:
From equations (1) and (2):
a2+(1−b)2=a2+(−1−b)2
Simplifying gives:
(1−b)2=(−1−b)2
Expanding both sides:
1−2b+b2=1+2b+b2
Canceling b2 and 1 from both sides:
−2b=2b⟹4b=0⟹b=0
4. Finding the Center of Circle 1:
Now substituting b=0 back into the equation for Circle 1:
(0−a)2+(1−0)2=r2⟹a2+1=r2(5)
For Circle 1 at (0, -1):
(0−a)2+(−1−0)2=r2⟹a2+1=r2(same as 5)
5. Finding the Center of Circle 2:
Similarly, substituting d=0 into Circle 2:
(0−c)2+(1−0)2=r2⟹c2+1=r2(6)
For Circle 2 at (0, -1):
(0−c)2+(−1−0)2=r2⟹c2+1=r2(same as 6)
6. Relating the Centers:
Since the tangent at (0, 1) of Circle 1 passes through the center of Circle 2, we can find the relationship between a and c. The tangent slope at (0, 1) is given by:
slope=a−00−1=−a
The equation of the tangent line at (0, 1) is:
y−1=−a(x−0)⟹y=−ax+1
This line passes through the center of Circle 2, (c,0):
0=−ac+1⟹ac=1(7)
7. Finding the Distance Between Centers:
From equations (5) and (6), we have:
r2=a2+1=c2+1⟹a2=c2
This gives us two cases:
a=cora=−c
Since the circles are distinct, we take a=−c.
The distance d between the centers (a,0) and (c,0) is:
d=|a−c|=|a−(−a)|=|2a|
8. Finding the Value of a:
From ac=1 and a=−c:
a(−a)=1⟹−a2=1⟹a2=1⟹a=1 or −1
9. Final Distance Calculation:
Thus, the distance between the centers is:
d=|2a|=|2⋅1|=2or|2⋅(−1)|=2
Final Answer:
The distance between the centers of the two circles is 2.
Let the centers of the two circles be (a,b) and (c,d) with equal radius r. The equations of the circles can be written as:
(x−a)2+(y−b)2=r2(Circle 1)
(x−c)2+(y−d)2=r2(Circle 2)
Since both circles intersect at the points (0, 1) and (0, -1), we can substitute these points into the equations of both circles.
(0−a)2+(1−b)2=r2(1)
(0−a)2+(−1−b)2=r2(2)
(0−c)2+(1−d)2=r2(3)
(0−c)2+(−1−d)2=r2(4)
From equations (1) and (2):
a2+(1−b)2=a2+(−1−b)2
Simplifying gives:
(1−b)2=(−1−b)2
Expanding both sides:
1−2b+b2=1+2b+b2
Canceling b2 and 1 from both sides:
−2b=2b⟹4b=0⟹b=0
Now substituting b=0 back into the equation for Circle 1:
(0−a)2+(1−0)2=r2⟹a2+1=r2(5)
For Circle 1 at (0, -1):
(0−a)2+(−1−0)2=r2⟹a2+1=r2(same as 5)
Similarly, substituting d=0 into Circle 2:
(0−c)2+(1−0)2=r2⟹c2+1=r2(6)
For Circle 2 at (0, -1):
(0−c)2+(−1−0)2=r2⟹c2+1=r2(same as 6)
Since the tangent at (0, 1) of Circle 1 passes through the center of Circle 2, we can find the relationship between a and c. The tangent slope at (0, 1) is given by:
slope=a−00−1=−a
The equation of the tangent line at (0, 1) is:
y−1=−a(x−0)⟹y=−ax+1
This line passes through the center of Circle 2, (c,0):
0=−ac+1⟹ac=1(7)
From equations (5) and (6), we have:
r2=a2+1=c2+1⟹a2=c2
This gives us two cases:
a=cora=−c
Since the circles are distinct, we take a=−c.
d=|a−c|=|a−(−a)|=|2a|
From ac=1 and a=−c:
a(−a)=1⟹−a2=1⟹a2=1⟹a=1 or −1
Thus, the distance between the centers is:
d=|2a|=|2⋅1|=2or|2⋅(−1)|=2
The distance between the centers of the two circles is 2.
Topper's Solved these Questions
- JEE 2019BOOK - CENGAGE ENGLISHCHAPTER - JEE 2019EXERCISE - Chapter 5 (Parabola)
8 Videos
- JEE 2019BOOK - CENGAGE ENGLISHCHAPTER - JEE 2019EXERCISE - Chapter 6 (Ellipse)
3 Videos
- JEE 2019BOOK - CENGAGE ENGLISHCHAPTER - JEE 2019EXERCISE - Chapter 2 (Straight Lines)
8 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - Archives (Numerical Value type)
2 Videos
- LIMITSBOOK - CENGAGE ENGLISHCHAPTER - LIMITSEXERCISE - Comprehension Type
4 Videos
Similar Questions
A circle passes through (0,0) and (1,0) and touches the circle x2+y2=9 then the centre of circle is -
View SolutionIf the line x+3y=0 is tangent at (0,0) to the circle of radius 1, then the centre of one such circle is
View SolutionProve that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
View SolutionProve that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
View SolutionIf a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
View SolutionIf a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
View SolutionThe line x = y touches a circle at the point (1, 1). If the circle also passes through the point (1, -3). Then its radius is 0
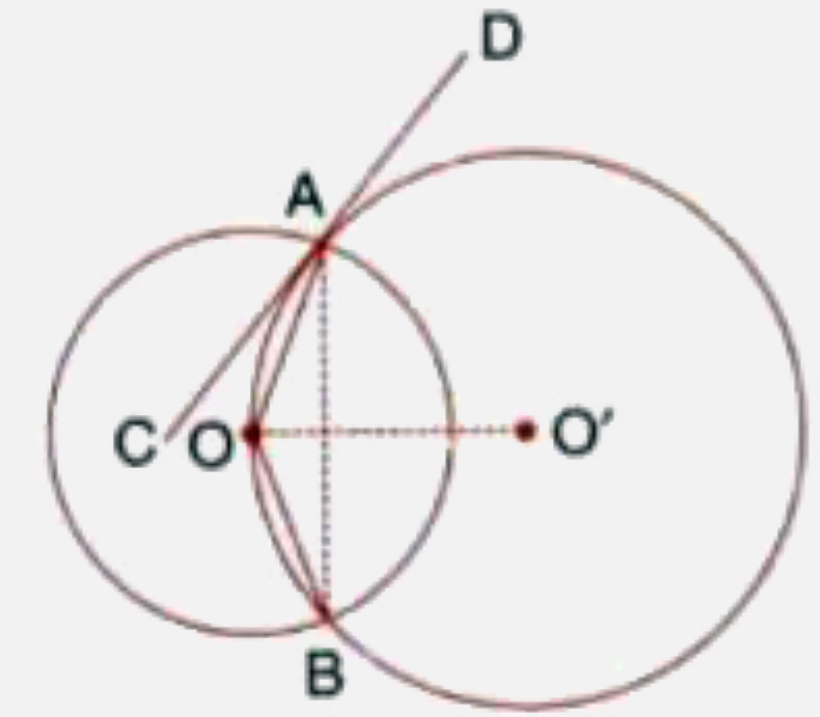
View SolutionTwo circles with centres O and O'are drawn to intersect each other at points A and B. Centre O of one circle lies on the circumference of the other circle and CD is drawn tangent to the circle with centre O' at A. prove that OA bisects angle BAC.
View SolutionIf a circle passes through the point (1, 1) and cuts the circle x2+y2=1 orthogonally, then the locus of its centre is
View SolutionThe line 2x−y+1=0 is tangent to the circle at the point (2, 5) and the centre of the circle lies on x−2y=4. The square of the radius of the circle is
View Solution
