Question

Plot $y=\sin x\text{and}y=\sin \left(\frac{x}{2}\right)$

Explanation

We have `y= " sin "(x)/(2).`
Since the period of `y= " sin "(x)/(2)" is "(2pi)/(1//2)=4pi,` the graph completes its one cycle in the interval of length `4pi.`
In any interval of length `4pi, " sin " (x)/(2)` takes all its values, which are `[-1,1].`
So graph of `y= " sin " (x)/(2)" for "x in [0,4pi]` is as follows.

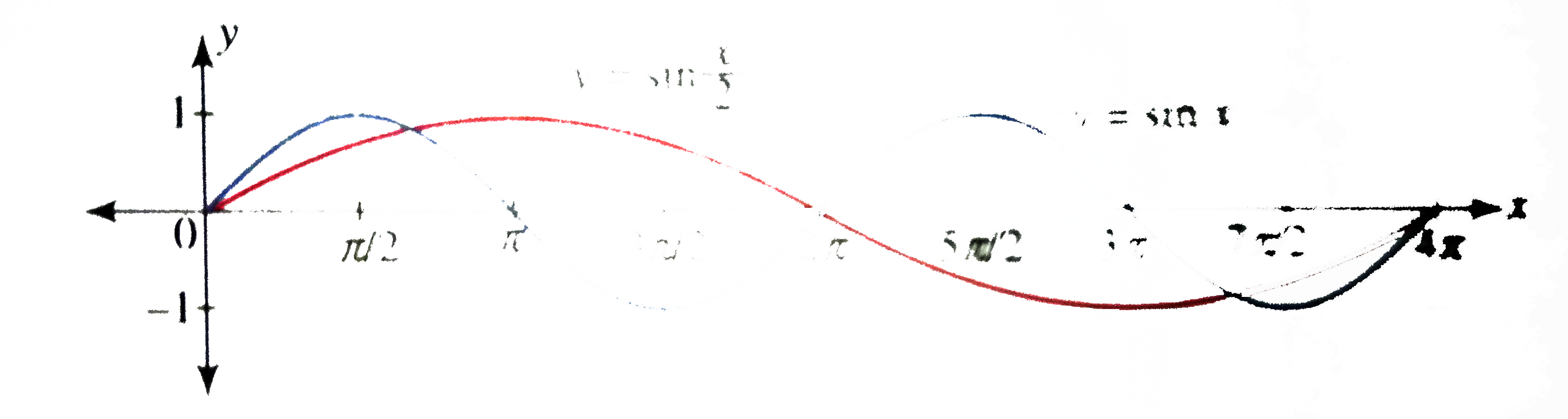
Thus, the graph of `y=sinx` is stretched by 2 units horizontally. In `[0,4pi],y=sinx` completes its two cycles.