Question

Let $f:R\to R$ be a function defined by $f\left(x\right)=max.\{x,x^3\}$. The set of all points where $f\left(x\right)$ is NOT differenctiable is
(a) $\{-1,1\}$
(b) $\{-1,0\}$
(c ) $\{0,1\}$
(d) $\{-1,0,1\}$

Explanation

We have `f(x) = max. {x, x^(3)}`
Draw the graphs of `y=x and y= x^(3)`

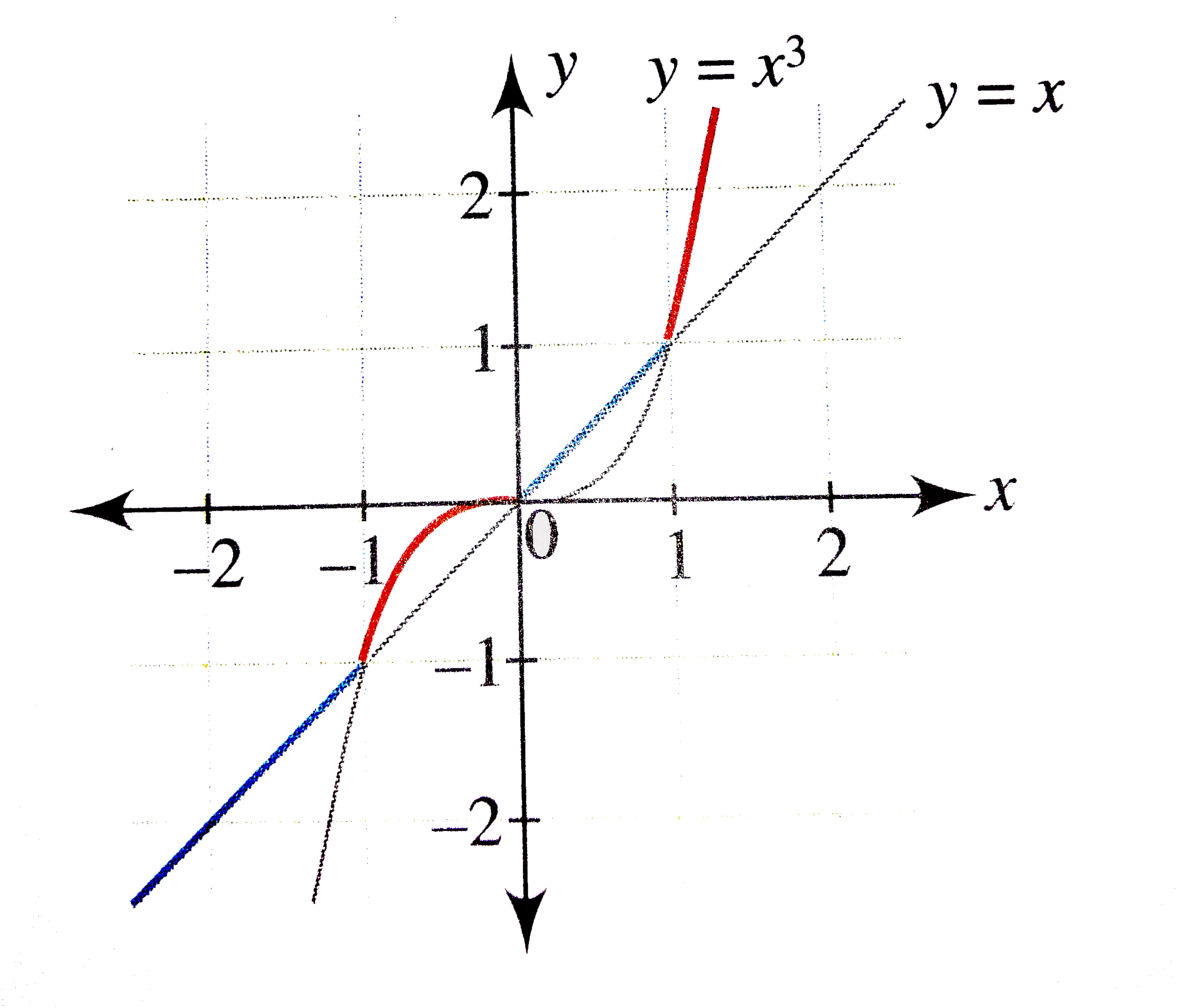
Graph of `y = f(x)` is combination of the combination of the blue and red-lined graphs.
From the graph, `y= f(x) ` is non-differentiable at `x=-1, x=0 and x=1`.