Question

The line joining two points A(2,0) and B(3,1) is rotated about A in anticlockwise direction through an angle of ${15}^{\circ }$. find the equation of line in the new position. If b goes to c in the new position what will be the coordinates of C.

Explanation

The slope of the line AB is
`m = (1-0)/(3-2) = 1 = tan45^(@)`
So, inclination of line AB with x-axis is `45^(@)`.

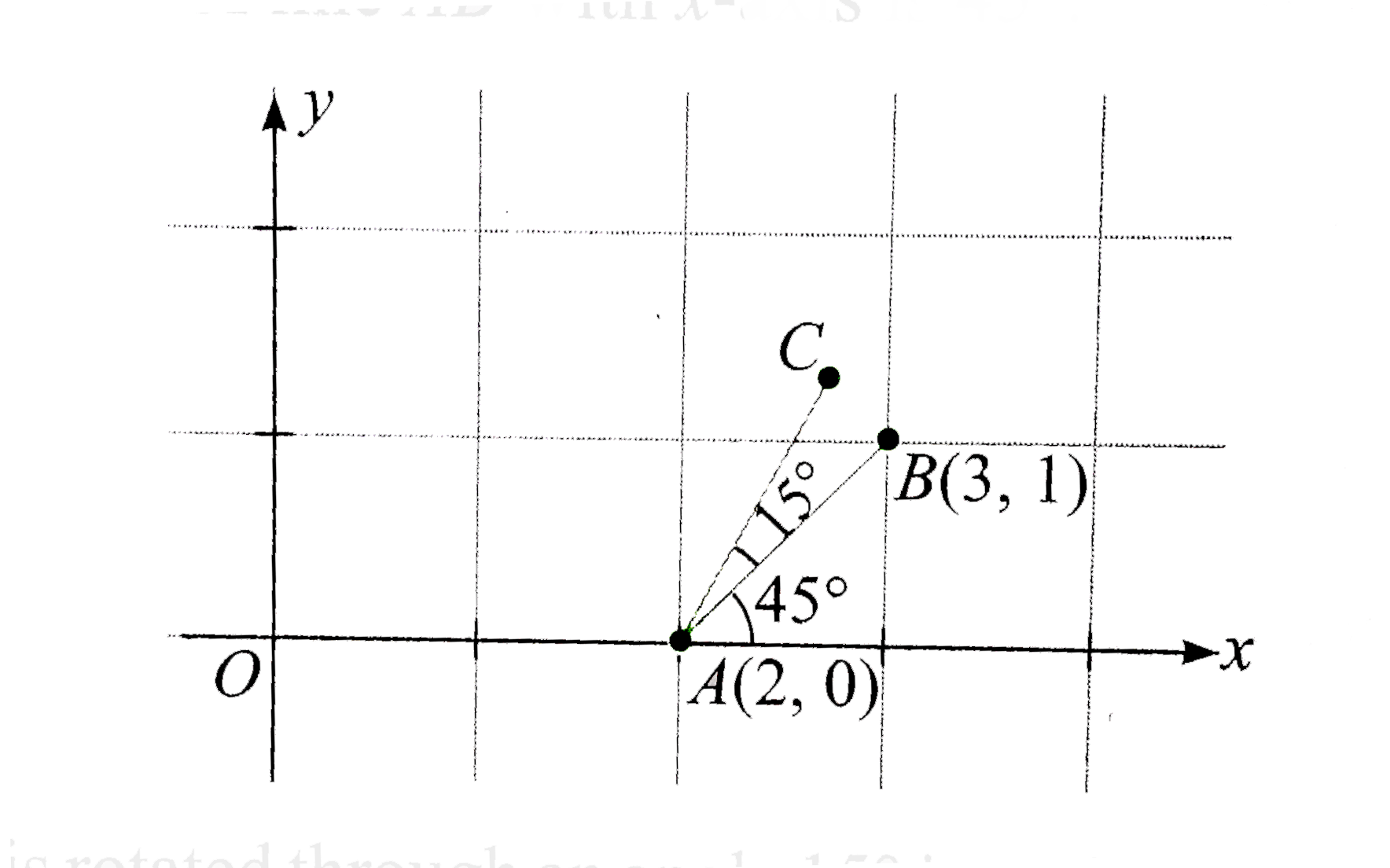
Now, AB is rotated through an angle `15^(@)` in anticlockwise direction about A and point B goes to C.
So, inclination of line AC is `60^(@)`.
Hence, equation of line AC is
`(x-2)/("cos" 60^(@)) = (y-0)/("sin" 60^(@))`
` or (x-2)/(1//2) = (y-0)/(sqrt(3)//2) = r ("say")`
` AC =AB = sqrt((3-2)^(2) + (1+0)^(2)) = sqrt(2)`
`"So, for point C, r" = sqrt(2).`
`therefore (x-2)/(1//2) = (y-0)/(sqrt(3)//2) = sqrt(2)`
`rArr x = 2+(1)/(2)sqrt(2) = 2 + (1)/(sqrt(2)) and y = (sqrt(3))/(2) * sqrt(2) = (sqrt(6))/(2)`
`"Therefore, C" -= (2+(1)/(sqrt(2)), (sqrt(6))/(2))`