If point P(3,2) divides the line segment AB internally in the ratio of 3:2 and point Q(−2,3) divides AB externally in the ratio 4:3 then find the coordinates of points A and B.
The correct Answer is:A=(6617,3117) and B=(4117,3617)
To find the coordinates of points A and B given the points P(3,2) and Q(-2,3) that divide the line segment AB in specific ratios, we can use the section formula. Let's break down the solution step by step.
Step 1: Use the Section Formula for Internal Division
Point P(3, 2) divides the line segment AB internally in the ratio 3:2. According to the section formula, if a point divides a line segment internally in the ratio m:n, the coordinates (x, y) of the point can be calculated as follows:
x=mx2+nx1m+n
y=my2+ny1m+n
Here, let A be (x1, y1) and B be (x2, y2). For point P:
- m = 3
- n = 2
- P(3, 2) = (x, y)
Substituting the values, we get:
3=3x2+2x13+2(1)
2=3y2+2y13+2(2)
Step 2: Simplify the Equations
From equation (1):
3(3+2)=3x2+2x1
15=3x2+2x1(Equation 1)
From equation (2):
2(3+2)=3y2+2y1
10=3y2+2y1(Equation 2)
Step 3: Use the Section Formula for External Division
Point Q(-2, 3) divides the line segment AB externally in the ratio 4:3. For external division, the formula is slightly different:
x=mx2−nx1m−n
y=my2−ny1m−n
For point Q:
- m = 4
- n = 3
- Q(-2, 3) = (x, y)
Substituting the values, we get:
−2=4x2−3x14−3(3)
3=4y2−3y14−3(4)
Step 4: Simplify the External Division Equations
From equation (3):
−2=4x2−3x1
4x2−3x1=−2(Equation 3)
From equation (4):
3=4y2−3y1
4y2−3y1=3(Equation 4)
Step 5: Solve the System of Equations
Now we have four equations:
1. 3x2+2x1=15 (Equation 1)
2. 3y2+2y1=10 (Equation 2)
3. 4x2−3x1=−2 (Equation 3)
4. 4y2−3y1=3 (Equation 4)
Solving for x-coordinates:
From Equation 1:
2x1=15−3x2⇒x1=15−3x22
Substituting x1 into Equation 3:
4x2−3(15−3x22)=−2
Multiply through by 2 to eliminate the fraction:
8x2−3(15−3x2)=−4
8x2−45+9x2=−4
17x2=41⇒x2=4117
Now substitute x2 back to find x1:
x1=15−3(4117)2=15−123172=255−123172=132172=6617
Solving for y-coordinates:
From Equation 2:
2y1=10−3y2⇒y1=10−3y22
Substituting y1 into Equation 4:
4y2−3(10−3y22)=3
Multiply through by 2:
8y2−3(10−3y2)=6
8y2−30+9y2=6
17y2=36⇒y2=3617
Now substitute y2 back to find y1:
y1=10−3(3617)2=10−108172=170−108172=62172=3117
Final Coordinates
Thus, the coordinates of points A and B are:
- A = (6617,3117)
- B = (4117,3617)
Point P(3, 2) divides the line segment AB internally in the ratio 3:2. According to the section formula, if a point divides a line segment internally in the ratio m:n, the coordinates (x, y) of the point can be calculated as follows:
y=my2+ny1m+n
- m = 3
- n = 2
- P(3, 2) = (x, y)
3=3x2+2x13+2(1)
2=3y2+2y13+2(2)
From equation (1):
3(3+2)=3x2+2x1
15=3x2+2x1(Equation 1)
2(3+2)=3y2+2y1
10=3y2+2y1(Equation 2)
Point Q(-2, 3) divides the line segment AB externally in the ratio 4:3. For external division, the formula is slightly different:
x=mx2−nx1m−n
y=my2−ny1m−n
- m = 4
- n = 3
- Q(-2, 3) = (x, y)
−2=4x2−3x14−3(3)
3=4y2−3y14−3(4)
From equation (3):
−2=4x2−3x1
4x2−3x1=−2(Equation 3)
3=4y2−3y1
4y2−3y1=3(Equation 4)
Now we have four equations:
1. 3x2+2x1=15 (Equation 1)
2. 3y2+2y1=10 (Equation 2)
3. 4x2−3x1=−2 (Equation 3)
4. 4y2−3y1=3 (Equation 4)
From Equation 1:
2x1=15−3x2⇒x1=15−3x22
4x2−3(15−3x22)=−2
Multiply through by 2 to eliminate the fraction:
8x2−3(15−3x2)=−4
8x2−45+9x2=−4
17x2=41⇒x2=4117
x1=15−3(4117)2=15−123172=255−123172=132172=6617
From Equation 2:
2y1=10−3y2⇒y1=10−3y22
4y2−3(10−3y22)=3
Multiply through by 2:
8y2−3(10−3y2)=6
8y2−30+9y2=6
17y2=36⇒y2=3617
y1=10−3(3617)2=10−108172=170−108172=62172=3117
Thus, the coordinates of points A and B are:
- A = (6617,3117)
- B = (4117,3617)
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.48videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.55videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.28videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE ENGLISHCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
→a,→b,→c are the position vectors of the three points A,B,C respectiveluy. The point P divides the ilne segment AB internally in the ratio 2:1 and the point Q divides the lines segment BC externally in the ratio 3:2 show that 3−−→PQ=−→a−8→b+9→c.
View SolutionDetermine a point which divides a line segment 7 cm long, internally in the ratio 2:3
View Solution
Knowledge Check
- Question 1 - Select One
If the line joining A(1,3,4) and B is divided by the point (-2,3,5) in the ratio 1:3 then the coordinates of B is
A(−11,3,8)B(−11,8,3)C(11,−8,3)D(−11,−3,8) - Question 2 - Select One
If point C(−4,1) divides the line segment joining the point A(2,−2) and B in the ratio 3:5, then the coordinates of B are
A(a) (−14,6)B(b) (6,−14)C(c) (−14,−6)D(d) (−6,−14)
In the figure given below , the line segment AB meets X-axis at A and Y-axis at B. The point P (-3, 4) on AB divides it in the ratio 2:3. Find the coordinates of A and B.
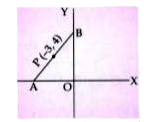 View Solution
View SolutionIf the point P (−1,2) divides the line segment joining A (2,5) and B in the ratio 3:4 , find the co-ordinate of B .
View SolutionFind the point which divides the line segment joining
(-1,2), (4,-5) in the ratio 3 : 2View SolutionThe point which divides the line segment joining the points A(0, 5) and B(5, 0) internally in the ratio 2:3 is __________
View SolutionThe xy-plane divides the line segment joining (1,2,3) and (-3,4,-5) (A) internally in the ratio 3:4 (B) externally in the ratio 5:3 (C) internally in the ratio 3:5 (D) none of these
View SolutionIf the point C(−1,2) divides internally the line segment joining A(2,5) and B in ratio 3:4, find the coordinates of B.
View Solution

