Question

A particle $p$
moves from the point $A(0,4)$
to the point $10,-4)$
. The particle $P$
can travel the upper-half plane $\{(x,y)\mid y\ge \}$
at the speed of $1m/s$
and the lower-half plane $\{(x,y)\mid y\le 0\}$
at the speed of 2 m/s. The coordinates of a point on the x-axis, if the
sum of the squares of the travel times of the upper- and lower-half planes is
minimum, are
(1, 0) (b) (2, 0) (c)
(4, 0) (d) (5, 0)

Options

A. (1,0)
B. (2,0)
C. (4,0)
D. (5,0)

Answer

B

Explanation

Let the point on the the axis be (c,0). Sum of the square of travel times is

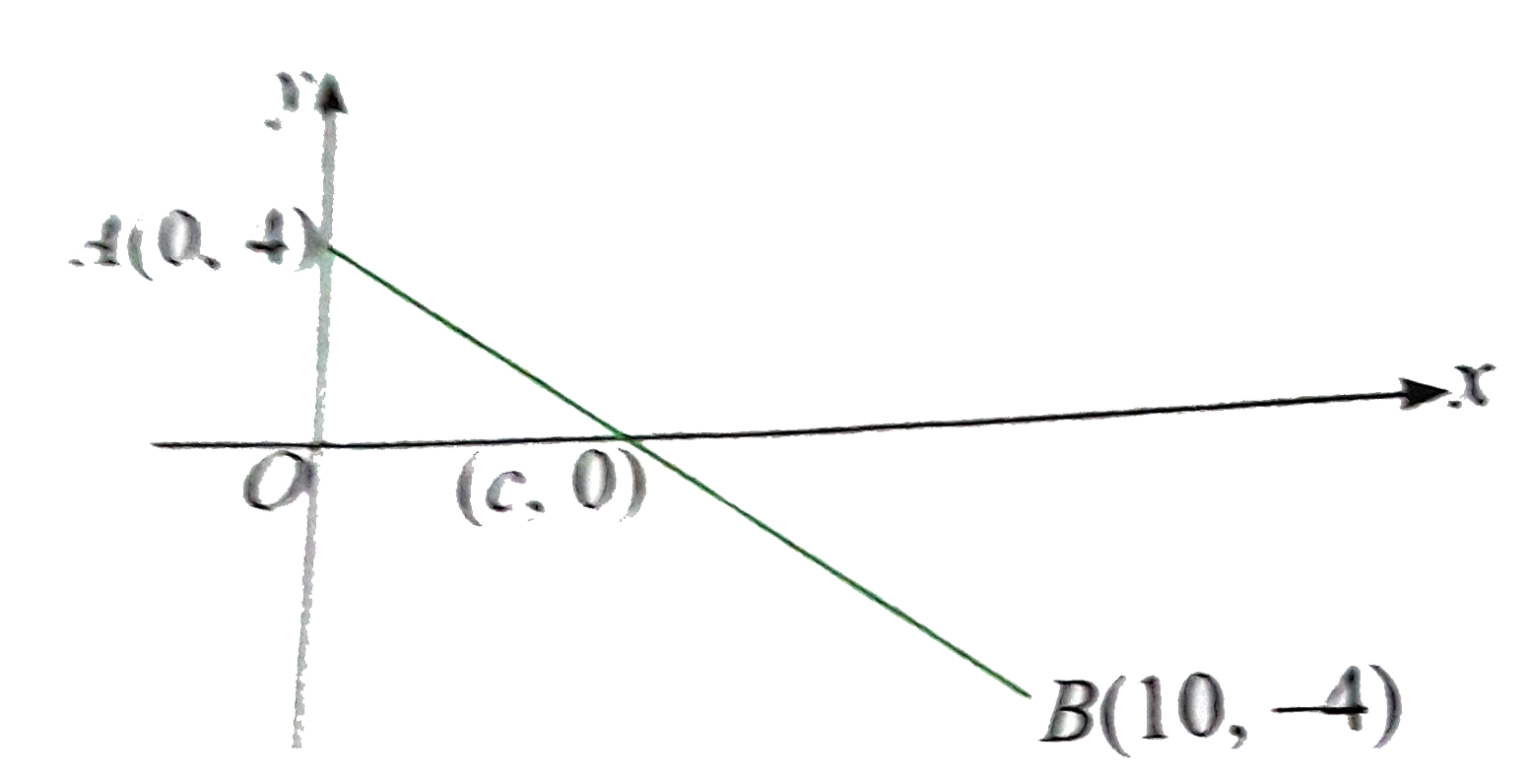
`T=((sqrt(c^2+16))/(1))^2+(sqrt((10-c)^2+16)/(2))^2`
`=c^2+16+(116+c^2-20c)/(4)`
`=(5)/(4)c^2-5c+45=(5)/(4)(c^2-4c+36)`
Therefore, T is minimum at `c=2`.