In the ΔABC, the coordinates of B are (0,0),AB=2,∠ABC=π3 and the middle point of BC has the coordinates (2,0). The centroid of the triangle is
(1/2,√3/2)
(5/3,1/√3)
(4+√3/3,1/3)
none of these
The correct Answer is:B
To find the centroid of triangle ABC given the coordinates of point B, the length of AB, and the angle ABC, we can follow these steps:
Step 1: Identify the coordinates of point B
Given that the coordinates of point B are (0, 0), we can denote:
- B(0,0)
Step 2: Determine the coordinates of point C
We know that the midpoint of BC is (2, 0). Let the coordinates of point C be C(xC,yC). Since the midpoint M of segment BC is given by:
M=(xB+xC2,yB+yC2)
Substituting the known values:
(2,0)=(0+xC2,0+yC2)
From the x-coordinate:
2=xC2⟹xC=4
From the y-coordinate:
0=yC2⟹yC=0
Thus, the coordinates of point C are:
- C(4,0)
Step 3: Determine the coordinates of point A
We are given that AB=2 and ∠ABC=π3 (which is 60 degrees). The coordinates of point A can be found using polar coordinates:
A(xA,yA)=(rcosθ,rsinθ)
where r=2 and θ=π3:
xA=2cos(π3)=2⋅12=1
yA=2sin(π3)=2⋅√32=√3
Thus, the coordinates of point A are:
- A(1,√3)
Step 4: Calculate the centroid of triangle ABC
The centroid G of triangle ABC is given by the formula:
G(xA+xB+xC3,yA+yB+yC3)
Substituting the coordinates of points A, B, and C:
G(1+0+43,√3+0+03)=G(53,√33)
Final Answer
The coordinates of the centroid G are:
G(53,√33)
Given that the coordinates of point B are (0, 0), we can denote:
- B(0,0)
We know that the midpoint of BC is (2, 0). Let the coordinates of point C be C(xC,yC). Since the midpoint M of segment BC is given by:
M=(xB+xC2,yB+yC2)
Substituting the known values:
(2,0)=(0+xC2,0+yC2)
From the x-coordinate:
2=xC2⟹xC=4
From the y-coordinate:
0=yC2⟹yC=0
Thus, the coordinates of point C are:
- C(4,0)
We are given that AB=2 and ∠ABC=π3 (which is 60 degrees). The coordinates of point A can be found using polar coordinates:
A(xA,yA)=(rcosθ,rsinθ)
where r=2 and θ=π3:
xA=2cos(π3)=2⋅12=1
yA=2sin(π3)=2⋅√32=√3
Thus, the coordinates of point A are:
- A(1,√3)
The centroid G of triangle ABC is given by the formula:
G(xA+xB+xC3,yA+yB+yC3)
Substituting the coordinates of points A, B, and C:
G(1+0+43,√3+0+03)=G(53,√33)
The coordinates of the centroid G are:
G(53,√33)
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Multiple correct13videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Linked10videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSYEM EXERCISE - Concept applications 1.69videos
COORDINATE SYSYEM
- BOOK - CENGAGE ENGLISHCHAPTER - COORDINATE SYSTEMEXERCISE - Multiple Correct Answers Type2videos
COORDINATE SYSTEM
- BOOK - CENGAGE ENGLISHCHAPTER - CROSS PRODUCTS EXERCISE - DPP 2.213videos
CROSS PRODUCTS
Similar Questions
In ABC, the coordinates of B are (0,0),AB=2,∠ABC=π3, and the middle point of BC has coordinates (2,0). The centroid o the triangle is (12,√32) (b) (53,1√3) (4+√33,13) (d) none of these
View SolutionIn ΔPQR, Q =(0,0), PQ = 2, ∠PQR=π3 and the mid point of QR has co-ordinates (2,0) then find the centroid of triangle
View SolutionA (4,8), B(-2,6) are two vertices of a triangle ABC. Find the coordinates of C if the centroid of the triangle is (2,7).
View SolutionA(-1, 3), B(4, 2) and C(3, -2) are the vertices of a triangle.
Find the coordinates of the centroid G of the triangle.View SolutionThe mid point of the sides of a triangle are (2,3,4),(1,5,−1) and (0,4,−2). Find the coordinates of the centroid of the triangle.
View SolutionIf (32,0),(32,6) and (−1,6) are mid-points of the sides of a triangle, then find
Centroid of the triangleView SolutionPoint O has coordinates (0, 0), point P lies on the graph of y=6−x2, and point B has coordinates (2√3,0). If OP=BP, the area of triangle OPB is
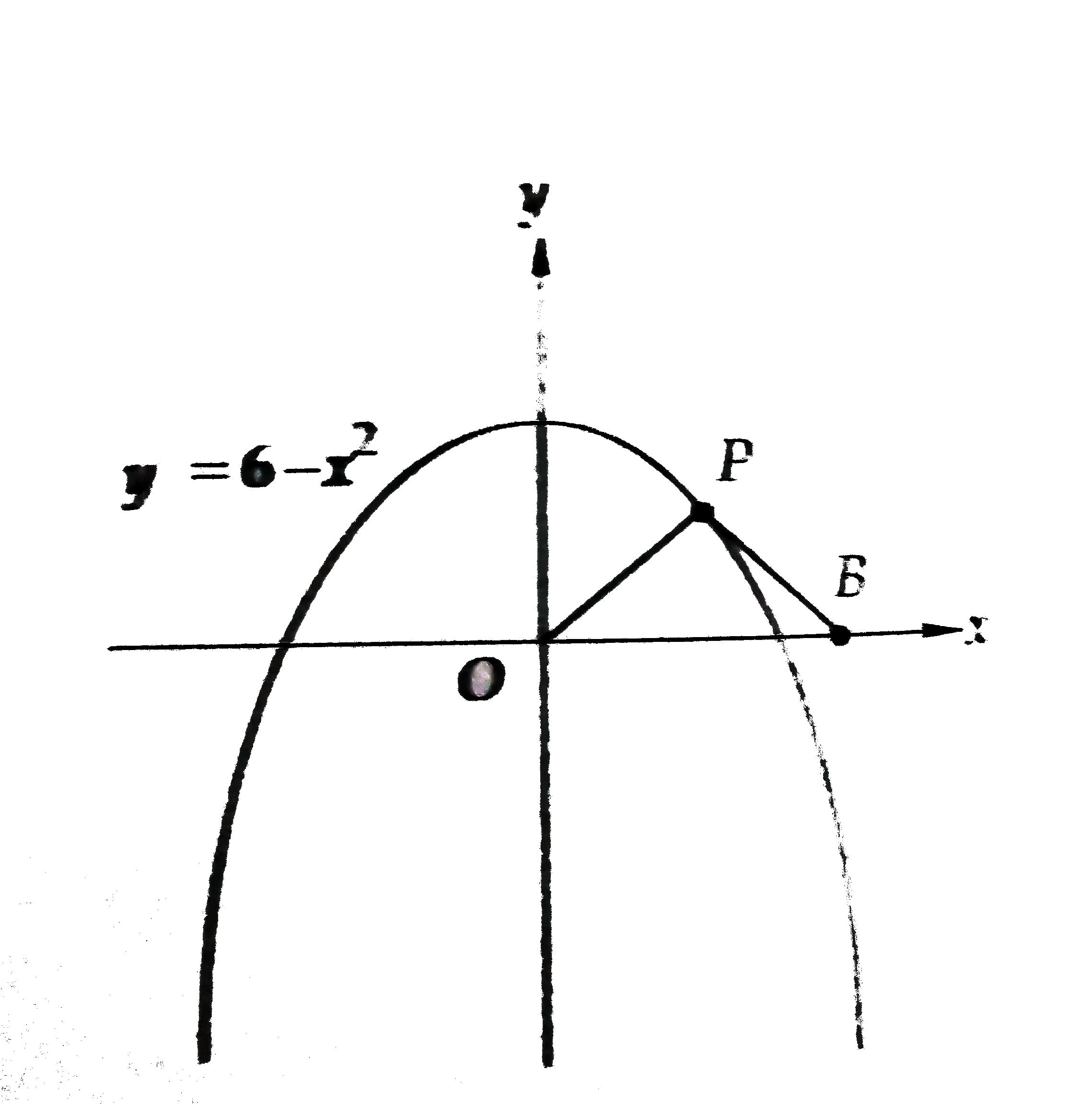 View Solution
View SolutionIf the coordinates of the points A,B,C be (−1,5),(0,0) and (2,2) respectively, and D be the middle point of BC, then the equation of the perpendicular drawn from B to the line AD is
View SolutionIf (1,2),(0,−1)and(2,−1) are the middle points of the sides of a triangle, find the coordinates of its centroid.
View SolutionIf a plane meets the coordinates axes at A, Band C, in such a way that the centroid of ΔABC is at the point (1,2,3), the equation of the plane is
View Solution

