If the positive integers are written in a triangular array as shown below,

then the row in which the number 2010 will be, is
65
61
63
65
The correct Answer is:C
(c) Let 2010 be in kth row
⇒kth term of series 1,2,4,7,……≤2010 (series formed by 1st term of each group)
and (k+1)th term of series 1,2,4,7,…>2010
Sn=1+2+4+7+...+Tn
Sn=1+2+4+...+Tn−1+Tn
∴¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯0=1+(1+2+3+...(n−1)terms−Tn)
⇒Tn=n2−n+22
⇒k2−k+22≤2010 and k2+k+22>2010
⇒k2−k−4018≤0 and k2+j−4018>0
⇒(k−12)2≤160734) and (k+12)2>160734
k−12≤63.3 and k+12>63.3
⇒k=63
Topper's Solved these Questions
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - EXERCIESE ( MULTIPLE CORRECT ANSWER TYPE )
66 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - EXERCIESE ( MATRIX MATCH TYPE )
3 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE )
8 Videos
- PROBABILITY IIBOOK - CENGAGE ENGLISHCHAPTER - PROBABILITY IIEXERCISE - MULTIPLE CORRECT ANSWER TYPE
6 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)
3 Videos
Similar Questions
Knowledge Check
The positive numbers are written in a triangular array as shown.
12345678910
The row in which number 2005 will be isA58B61C63D65The odd positive integers are arranged in a traingle as follows :

Find the sum of the number in the nth row of this arrangement :Aa. n2+nBb. 2n2−2+nCc. n3Dd. none of theseThe natural numbers are written as follows :
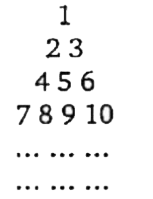
The sum of numbers in the nth row is :Aa. n(n+1)2Bb. n2+12Cc. n(n2+1)2Dd. none of these
The numbers 2 3 4 5 6 7 8 are to be placed one per square in the diagram as shown below such that the sum of the four numbers in the horizontal row as well as vertical row is 21 then the number of ways in which this can be done is ?
View SolutionIf we divide a positive integer by another positive integer, what is the resulting number?
View SolutionTwo positions of a dice are shown below. When number 'one' is on the top, what number will be at the bottom?
View SolutionIt is possible to arrange eight of the nine numbers 2, 3, 4, 5, 7, 10, 11, 12, 13 in the vacant squares of the 3 by 4 array shown below so that the arithmetic average of the numbers in each row and column is the same integer.
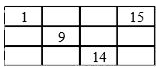
The arithmetic average is
View SolutionPositive integers
View Solution