Let be a function defined by f(x) = a sin ( x +) + b cosx + c. If f is both one-one and onto, then find the set X and Y
Let
where
For to be one-one, we must have Thus,
domain is and range is
Topper's Solved these Questions
- RELATIONS AND FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - CONCEPT APPLICATION EXERCISE 1.1
15 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - CONCEPT APPLICATION EXERCISE 1.2
5 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Archives(Matrix Match Type)
1 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Archives (Numerical Value Type)
3 Videos
- SCALER TRIPLE PRODUCTS BOOK - CENGAGE ENGLISHCHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.3
11 Videos
Similar Questions
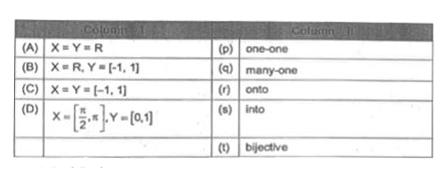
Let f:X→Y be a function defined by f(x)=sinx.
View SolutionLet f:R→R be a function defined by f(x)=x3+x2+3x+sinx. Then f is
View SolutionLet f:R→R be a function defined by f(x)=x3+x2+3x+sinx. Then f is
View SolutionLet f:R→B, be a function defined f(x)=tan−1.2x√3(1+x2), then f is both one - one and onto when B, is the interval
View SolutionLet f:A→B be a function defined by f(x)=√3sinx+cosx+4. If f is invertible, then
View SolutionLet the function f:R→R be defined by f(x)=cosx,∀x∈R. Show that f is neither one-one nor onto.
View SolutionLet the function f:R→R be defined by f(x)=cosx,∀x∈R. Show that f is neither one-one nor onto.
View SolutionLet f: R→R be a function defined as f (x) = 3x+ 7 , x∈R . Show that f is an onto functions.
View SolutionLet the function f:R→R be defined by f(x)=cosx,∀x∈R. Show that f is neither one-one nor onto
View SolutionLet A = R - {2}, B = R - {1}. If f : A→B is a function defined by f(x) = x−1x−2, show that f is one-one and onto.
View Solution
