A line x=k intersects the graph of y=log4x and y=log4(x+4). The distance between the points of intersection is 0.5, then the value of k is
1
2
3
4
The correct Answer is:D
To solve the problem step by step, we can follow these procedures:
Step 1: Identify the Points of Intersection
The line x=k intersects the graphs of y=log4x and y=log4(x+4) at two points:
- Point A: (k,log4k)
- Point B: (k,log4(k+4))
Step 2: Calculate the Distance Between Points A and B
The distance d between points A and B can be calculated using the distance formula. Since both points have the same x-coordinate (k), the distance is given by the difference in their y-coordinates:
d=|y2−y1|=|log4(k+4)−log4k|
Step 3: Use the Logarithmic Property
Using the property of logarithms, we can simplify the expression for distance:
d=|log4(k+4)−log4k|=|log4(k+4k)|
Step 4: Set the Distance Equal to 0.5
According to the problem, the distance is given as 0.5:
|log4(k+4k)|=0.5
Step 5: Remove the Absolute Value
Since logarithms can be positive or negative, we can write two equations:
1. log4(k+4k)=0.5
2. log4(k+4k)=−0.5
Step 6: Solve the First Equation
Starting with the first equation:
log4(k+4k)=0.5
This implies:
k+4k=40.5=2
Cross-multiplying gives:
k+4=2k
Rearranging leads to:
k=4
Step 7: Solve the Second Equation
Now, solving the second equation:
log4(k+4k)=−0.5
This implies:
k+4k=4−0.5=12
Cross-multiplying gives:
k+4=k2
Rearranging leads to:
2(k+4)=k⟹2k+8=k⟹k=−8
Step 8: Conclusion
The value of k must be positive since it represents a point on the graph of a logarithm. Therefore, the only valid solution is:
4
---
The line x=k intersects the graphs of y=log4x and y=log4(x+4) at two points:
- Point A: (k,log4k)
- Point B: (k,log4(k+4))
The distance d between points A and B can be calculated using the distance formula. Since both points have the same x-coordinate (k), the distance is given by the difference in their y-coordinates:
Using the property of logarithms, we can simplify the expression for distance:
According to the problem, the distance is given as 0.5:
Since logarithms can be positive or negative, we can write two equations:
2. log4(k+4k)=−0.5
Starting with the first equation:
Now, solving the second equation:
The value of k must be positive since it represents a point on the graph of a logarithm. Therefore, the only valid solution is:
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - LOGARITHM AND ITS APPLICATIONS EXERCISE - Subjective Type9videos
LOGARITHM AND ITS APPLICATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - LINEAR COMBINATION OF VECTORS, DEPENDENT AND INDEPENDENT VECTORSEXERCISE - DPP 1.210videos
LINEAR COMBINATION OF VECTORS, DEPENDENT AND INDEPENDENT VECTORS
- BOOK - CENGAGE ENGLISHCHAPTER - LOGARITHM AND ITS PROPERTIESEXERCISE - JEE ADVANCED1videos
LOGARITHM AND ITS PROPERTIES
Similar Questions
Knowledge Check
- Question 1 - Select One
The graph of y=log5xandy=ln 0.5x intersect at a point where x equals
A6.24B5.44C1.69D1.14 - Question 2 - Select One
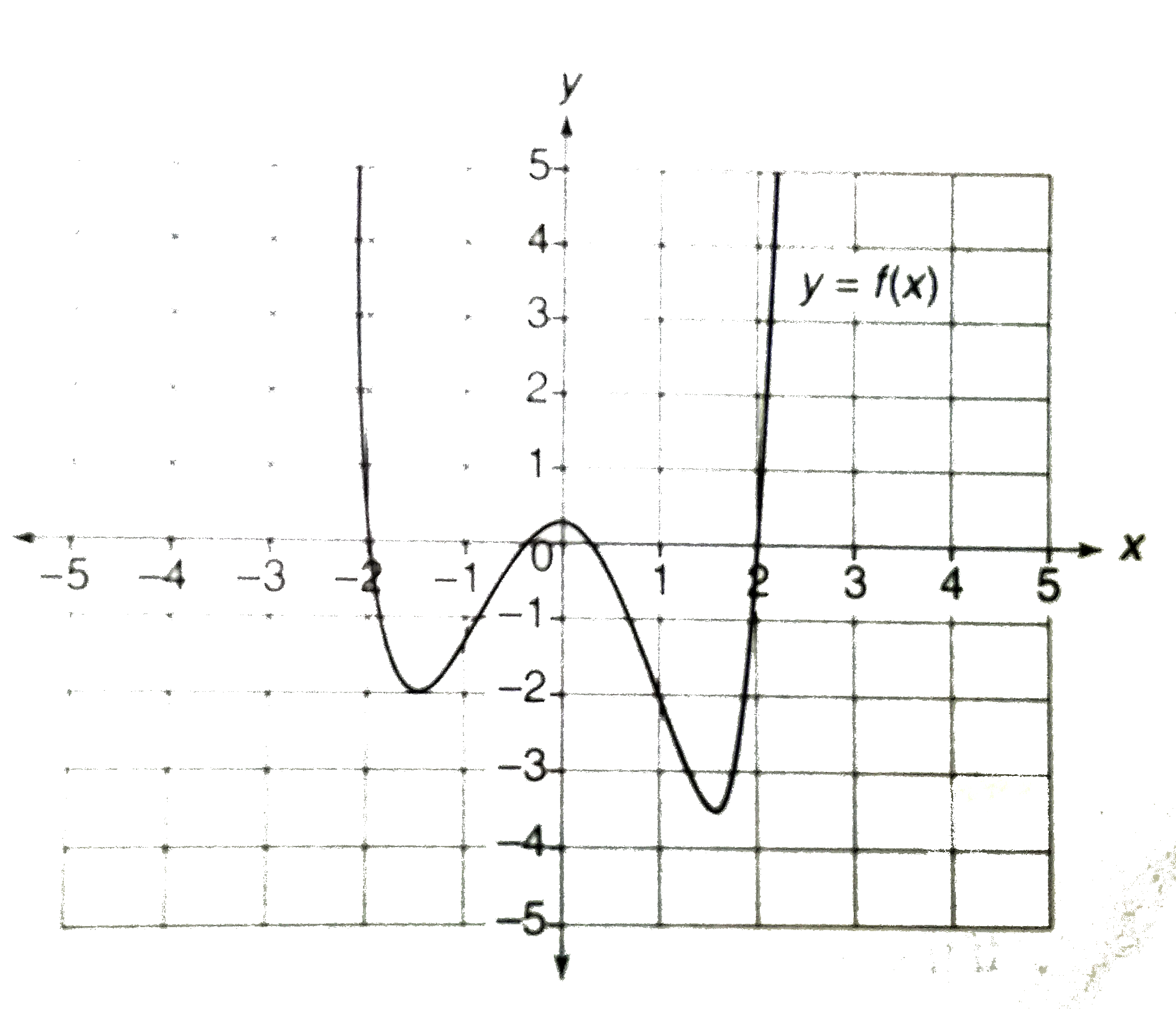
If g(x)=−2 intersects the graph of y=f(x)+k at one point, which of these choices could be the value of k?A−1.5B−0.5C0D1.5
The line 4x−7y+10=0 intersects the parabola y2=4x at the points P and Q. The coordinates of the point of intersection of the tangents drawn at the points P and Q are
View SolutionFind the point of intersection of the lines 2x−3y+8=0 and 4x+5y=6
View SolutionThe distance of the point of intersection of the lines 2x−3y+5=0 and 3x+4y=0 from the line 5x−2y=0 is
View SolutionThe distance of the point of intersection of the lines 2x−3y+5=0 and 3x+4y=0 from the line 5x−2y=0 is
View SolutionCosine of the acute angle between the curve y=3x−1logex and y=xx−1, at the point of intersection (1,0) is
View SolutionFind the point of intersection of the line, x3−y4=0andx2+y3=1
View Solution

