The lateral edge of a regular rectangular pyramid is acmlong. The lateral edge makes an angle α with the plane of the base. Find the value of α for which the volume of the pyramid is greatest.
The correct Answer is:α=tan−1(1)√2
h= a sin αandx=acosα
x2+h2=a2
∴V=132h=132x2h
∴v(α)=23a2cos2αasinα
=23a3sinαcos2α
Now v(α)=0 then n tan α=1√2
∴Vmax=4√3a327

Topper's Solved these Questions
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Exercise
93 Videos
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Multiple correct answers type
48 Videos
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Concept Application Exercise 6.6
9 Videos
- METHODS OF DIFFERENTIATIONBOOK - CENGAGE ENGLISHCHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - Single Correct Answer Type
46 Videos
- MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSEXERCISE - Comprehension Type
6 Videos
Similar Questions
Comprehesion-I
Let k be the length of any edge of a regular tetrahedron (all edges are equal in length). The angle between a line and a plane is equal to the complement of the angle between the line and the normal to the plane whereas the angle between two plane is equal to the angle between the normals. Let O be the origin and A,B and C vertices with position vectors →a,→b and →c respectively of the regular tetrahedron.
The value of [→a→b→c]2 is
View SolutionThe pyramid in Figure composed of a square base of area 16 and four isoceles triangles, in which each base angle measures 60∘. What is the volume of the pyramid ?
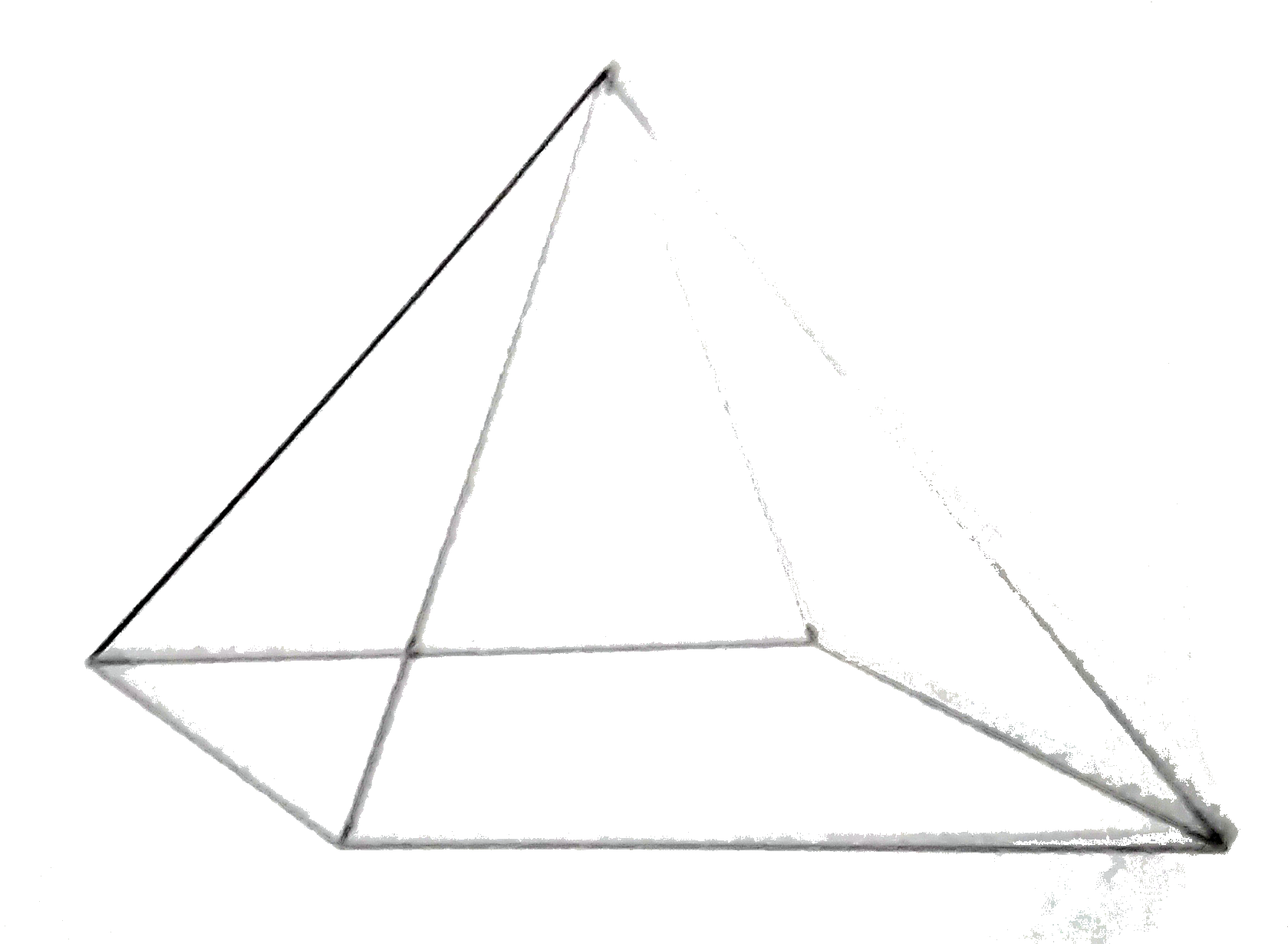
View Solution
Knowledge Check
If the plane 2x−3y+6z=11 makes an angle sin−1(α) with the x-axis, then the value of α is
A√32B2√3C17D27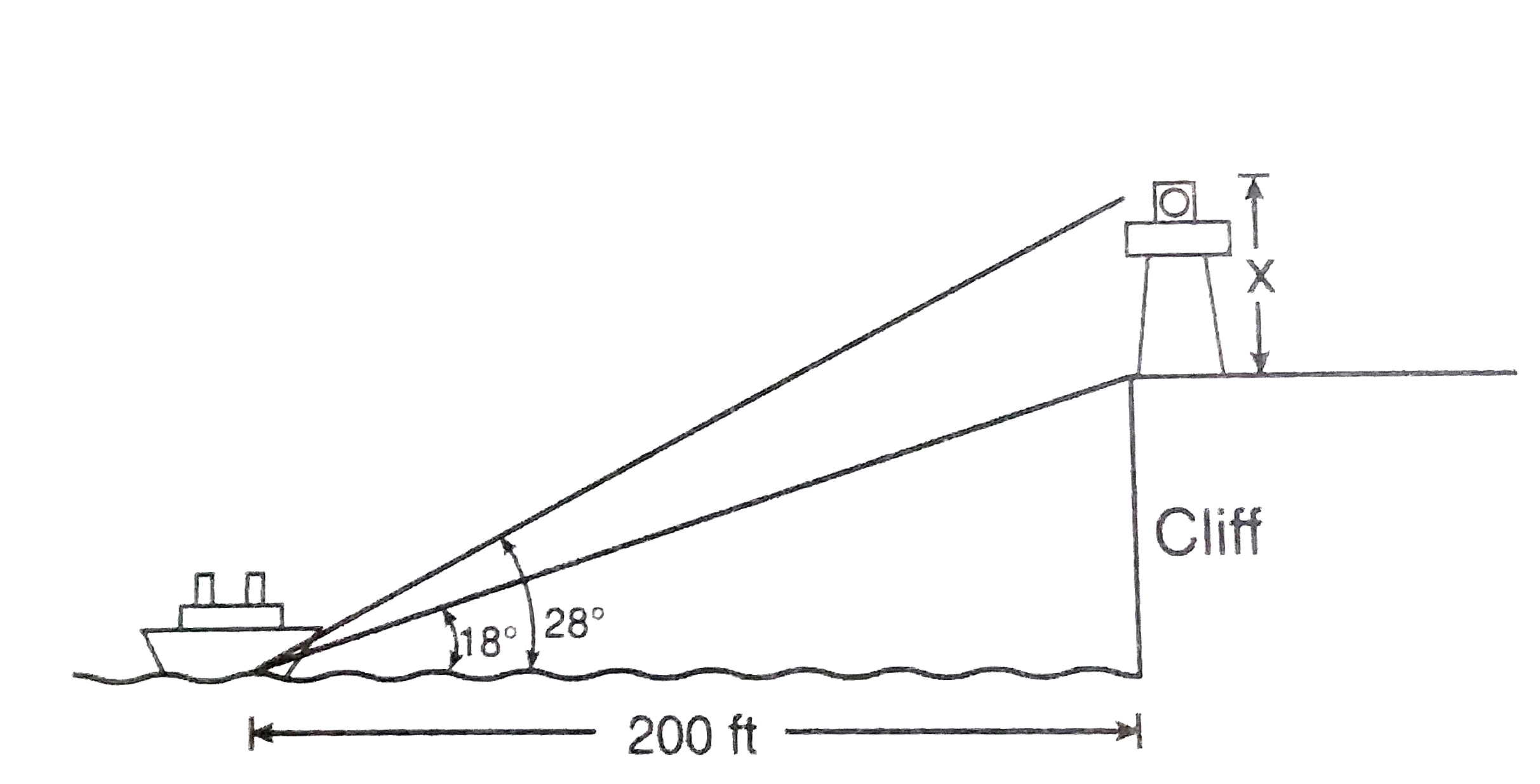
A lighthouse is built on the edge of a cliff near the ocean, as shown in the diagram above. From a boat located 200 feet from the base of the cliff, the angle of elevation to the top of the cliff is 18∘ and the angle of elevation to the top of the lighthouse is 28∘. Which of the following equations could be used to find the height of the lighthouse, x, in feet?Ax=200tan10∘Bx=200(tan28∘−tan18∘)Cx=200tan28∘−tan18∘Dx=200(tan18∘tan28∘)
At t=0, a force F = kt is applied on a block of mass m making an angle α with the horizontal . Suppose surfaces to be smooth. Find the velocity of the body at the time of breaking off the plane.

View SolutionThe base of an isosceles triangle measures 4 units base angle is equal to 45∘. A straight line cuts the extension of the base at a point M at the angle θ and bisects the lateral side of the triangle which is nearest to M.
The possible range of values in which area of quadrilateral which straight line cuts off from the given triangle lie in (a) (5/2, 7/2) (b) (4,3) (c) (4,5) (d) (3,4)
View SolutionA large , heavy box is sliding without friction down a smooth plane of inclination θ . From a point P on the bottom of the box , a particle is projected inside the box . The initial speed of the particle with respect to the box is u , and the direction of projection makes an angle α with the bottom as shown in Figure .
Find the distance along the bottom of the box between the point of projection p and the point Q where the particle lands . ( Assume that the particle does not hit any other surface of the box . Neglect air resistance .

View SolutionIf a line makes angle alpha,beta\ and\ gamma with the coordinate axes, find the value of cos2alpha+cos2beta+cos2gamma.
View SolutionA ball is projected form a point A on a smooth inclined plane which makes an angle a to the horizontal. The velocity of projection makes an angle θ with the plane upwards. If on the second bounce the ball is moving perpendicular to the plane, find e in terms of α and θ. Here e is the coefficient of restitution between the ball and the plane.
View SolutionA uniform cube of side 'a' and mass m rests on a rough horizontal table. A horizontal force F is applied normal to one of the faces at a point directly below the centre of the face, at a height a/4 above the base.
a. What is the minimum value of F for which the cube begins to tip about an edge?
b. What is the minimum value of its so that toppling occurs?
c. If μ=μmin find minimum force for topping.
d. Find minimum μs so that Fmin can cause toppling.
View Solution
