consider function f(x) =x4−14x2+24x−3. Now match the following lists:

Text Solution
The correct Answer is:a→r;b→s;c→q;d→p
f(x)=4x3−28x+24
=4(x3−7x+6)
=4(x3−x2+x−x−6x+6
=(x−1)(x2+x−6)
=4(x−1)(x+3)(x−2)

Now nature of roots f(X)+p=0 can be obtained shifiting the graph of y=f(x) by p units upward or downward depending on whether p is positive or negative
|
Topper's Solved these Questions
- MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONSEXERCISE - Linked comprehension type
50 Videos
- METHODS OF DIFFERENTIATIONBOOK - CENGAGE ENGLISHCHAPTER - METHODS OF DIFFERENTIATIONEXERCISE - Single Correct Answer Type
46 Videos
- MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - MONOTONOCITY AND NAXINA-MINIMA OF FUNCTIONSEXERCISE - Comprehension Type
6 Videos
Similar Questions
Knowledge Check
If f(x)=x3−9x2+24x, then f
Ahas maximum value =36Bhas minimum value =16Chas minima at x=2Dhas maxima at x=4If f(x)=x3−9x2+24x, then f
Ahas maximum value =16Bhas minimum value =10Chas minima at x=2Dhas maxima at x=2Consider equation x4−6x3+8x2+4ax−4a2=0,a∈R. Then match the following lists:
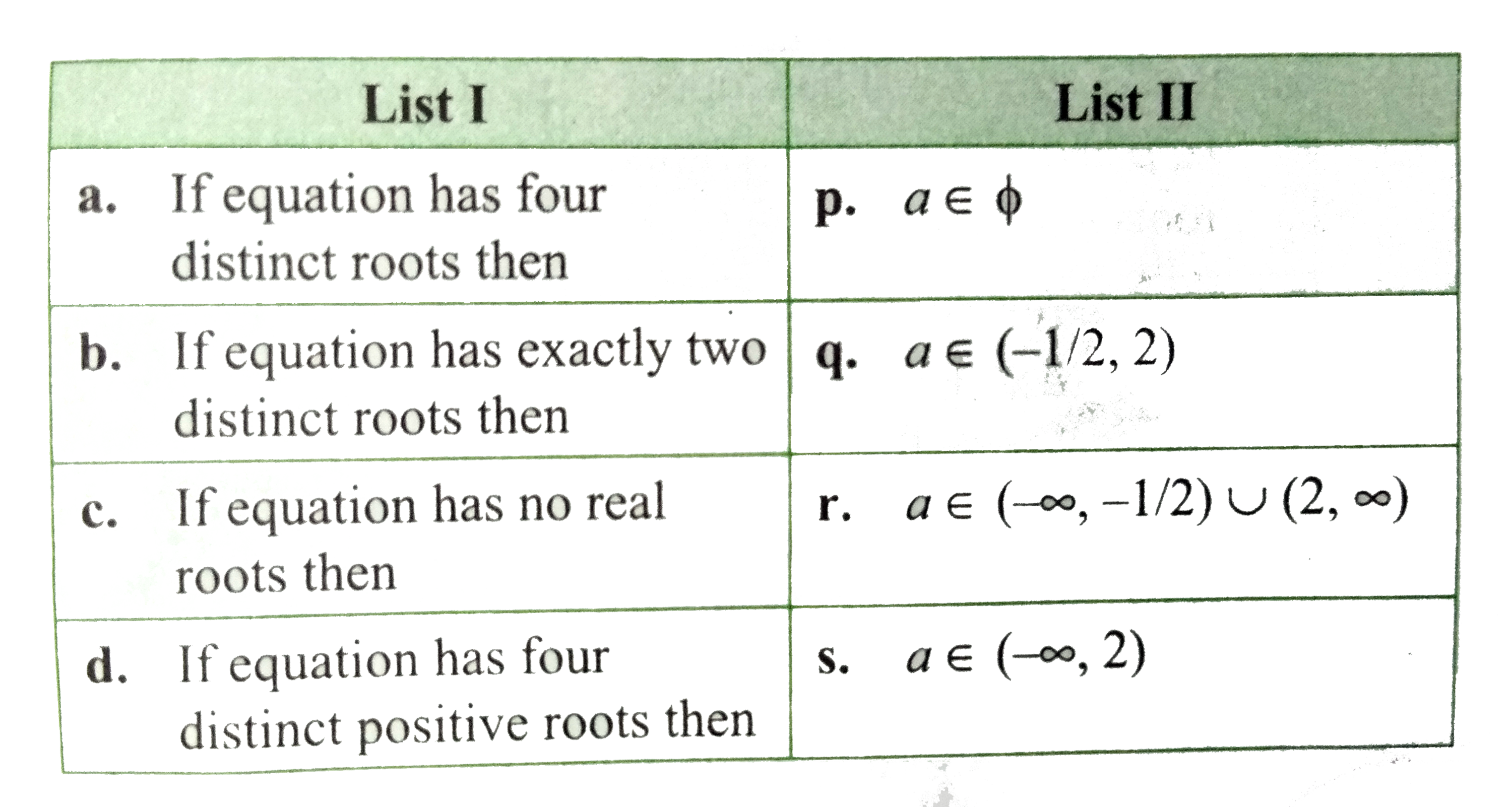 Aabcd(1)qssrBabcd(2)rsqpCabcd(3)qsrpDabcd(4)qrpp
Aabcd(1)qssrBabcd(2)rsqpCabcd(3)qsrpDabcd(4)qrpp
Consider limx→∞(x3+x2+x+sinxx2+2cosx−asinx−bx+c)=4. Now, match the following lists and then choose the correct code.
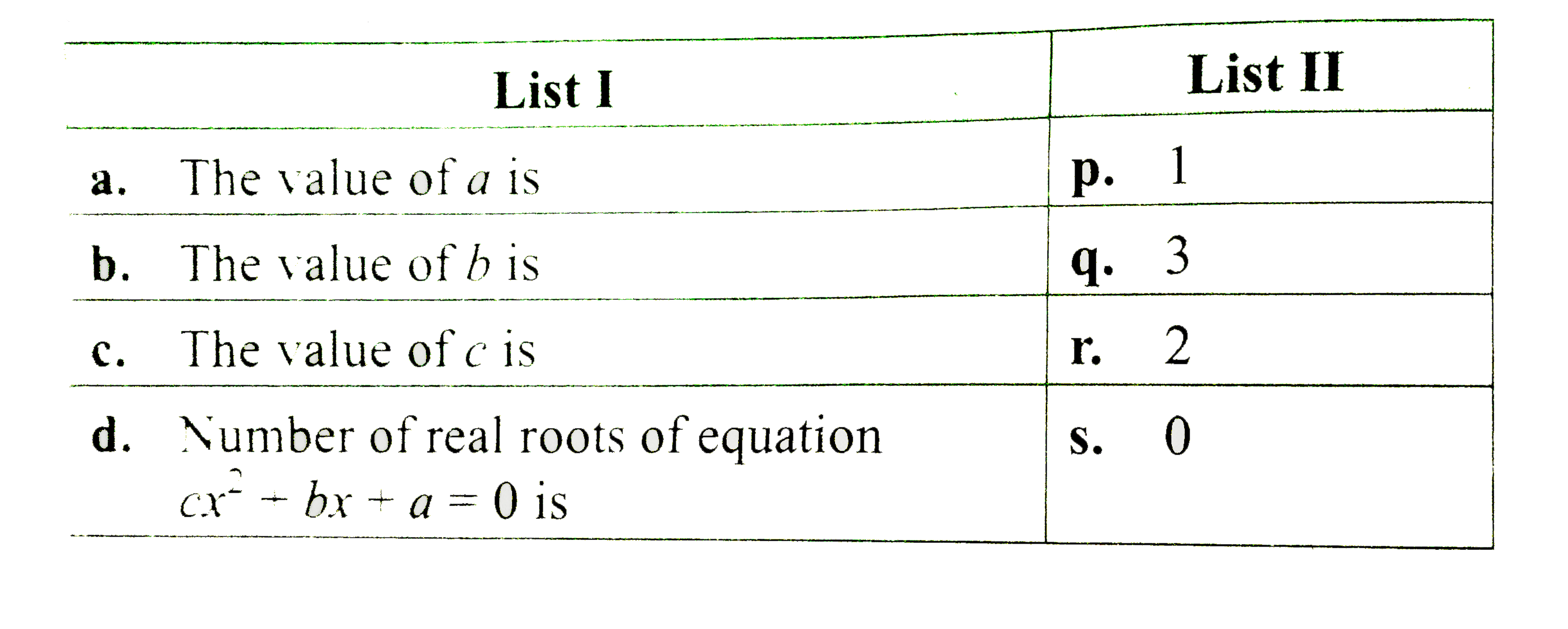
View SolutionConsider limx→∞(x3+x2+x+sinxx2+2cosx−asinx−bx+c)=4. Now, match the following lists and then choose the correct code.

View SolutionIf the function f(x)=x3−24x2+6kx−8 is maximum at x = 2 then find value of k.
View SolutionIf the function f(x)=x3−24x2+6kx−8 is maximum at x = 2. Then, find value of k.
View Solutionf(x)=4x3−12x2+14x−3, g(x)=2x−1
View Solution
