Determine p such that the length of the such-tangent and sub-normal is equal for the curve y=epx+px at the point (0,1).
To solve the problem of finding the value of p such that the lengths of the sub-tangent and sub-normal are equal for the curve y=epx+px at the point (0,1), we can follow these steps:
Step 1: Find the derivative of the function
Given the function:
y=epx+px
We need to find the derivative dydx:
dydx=ddx(epx)+ddx(px)=pepx+p
Step 2: Evaluate the derivative at the point (0, 1)
Now, we evaluate the derivative at x=0:
dydx∣∣∣x=0=pep⋅0+p=p+p=2p
Step 3: Calculate the lengths of the sub-tangent and sub-normal
The length of the sub-tangent LT is given by:
LT=y1m=12p
where y1=1 and m=2p.
The length of the sub-normal LN is given by:
LN=y1⋅m=1⋅(2p)=2p
Step 4: Set the lengths equal
To find p, we set the lengths equal:
12p=2p
Step 5: Solve for p
Cross-multiplying gives:
1=4p2
Thus,
4p2=1⟹p2=14⟹p=±12
Final Answer
The values of p are:
p=12orp=−12
Given the function:
y=epx+px
We need to find the derivative dydx:
dydx=ddx(epx)+ddx(px)=pepx+p
Now, we evaluate the derivative at x=0:
dydx∣∣∣x=0=pep⋅0+p=p+p=2p
The length of the sub-tangent LT is given by:
LT=y1m=12p
where y1=1 and m=2p.
LN=y1⋅m=1⋅(2p)=2p
To find p, we set the lengths equal:
12p=2p
Cross-multiplying gives:
1=4p2
Thus,
4p2=1⟹p2=14⟹p=±12
The values of p are:
p=12orp=−12
Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - SOLVED EXAMPLES
15 Videos
- APPLICATION OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - MATRIX MATCH TYPE
3 Videos
- APPLICATION OF DERIVATIVESBOOK - CENGAGE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - JEE PREVIOUS YEAR
10 Videos
- 3D COORDINATION SYSTEM BOOK - CENGAGE ENGLISHCHAPTER - 3D COORDINATION SYSTEM EXERCISE - DPP 3.1
11 Videos
- APPLICATION OF INTEGRALSBOOK - CENGAGE ENGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - All Questions
142 Videos
Similar Questions
Determine p such that the length of the sub-tangent and sub-normal is equal for the curve y=epx+px at the point (0,1).
View SolutionLet P (h,K) be any point on curve y=f(x). Let tangent drawn at point P meets x-axis at T & normal at point P meets x-axis at N (as shown in figure) and m =dydx](h,k)) = shope of tangent.

(i) Length of tangent =PT =|K|√1+1m2
(ii) Length of Normal =PN +|K|√1+m2
(iii) Length subtangent = Projection of segment PT on x-axis =TM=∣∣∣km∣∣∣
(iv) Length of subnormal =Projection of line segment PN on x-axis =MN =|Km|
Determine 'p' such that the length of the subtangent and subnormal is equal for the curve y=epx+px at the point (0,1)
View SolutionFind the length of sub-tangent to the curve y=ex/a
View SolutionThe curve y=f(x) in the first quadrant is such that the y - intercept of the tangent drawn at any point P is equal to twice the ordinate of P If y=f(x) passes through Q(2,3), then the equation of the curve is
View SolutionFind the length of the tangent for the curve y=x3+3x2+4x−1 at point x=0.
View SolutionFind the length of the tangent for the curve y=x3+3x2+4x−1 at point x=0.
View SolutionLet P (h,K) be any point on curve y=f(x). Let tangent drawn at point P meets x-axis at T & normal at point P meets x-axis at N (as shown in figure) and m =dydx](h,k)) = shope of tangent.
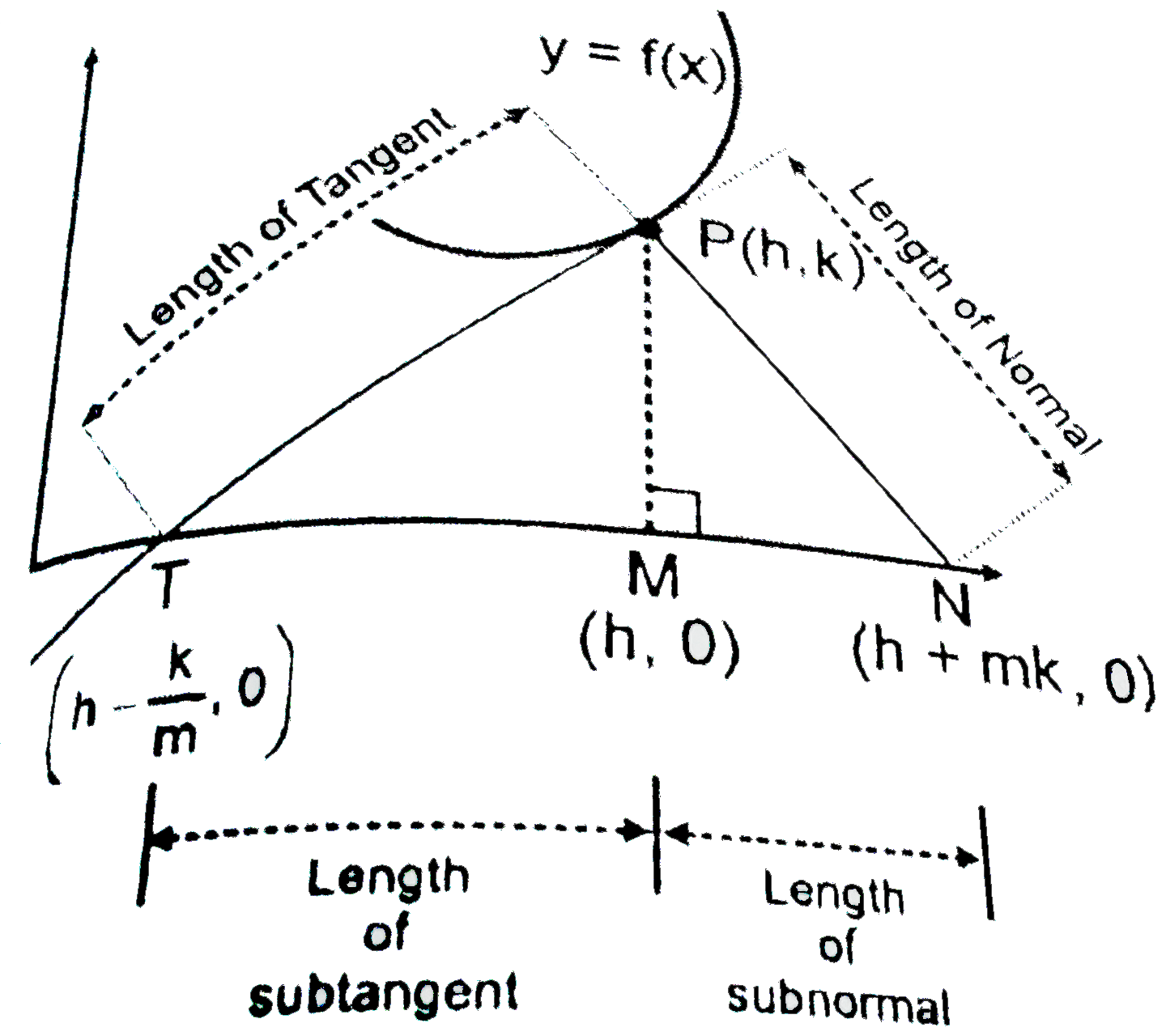
(i) Length of tangent =PT =|K|√1+1m2
(ii) Length of Normal =PN +|K|√1+m2
(iii) Length subtangent = Projection of segment PT on x-axis =TM=∣∣∣km∣∣∣
(iv) Length of subnormal =Projection of line segment PN on x-axis =MN =|Km|
Find the product of length of tangent and length of normal for the curve y=x3+3x2+4x−1 at point x=0
View SolutionThe length of the normal to the curve y=a(e−x/a+ex/a2) at any point varies as the
View SolutionTangent and normal at any point P of the parabola y2=4ax(a>0) meet the x-axis at T and N respectively. If the lengths of sub-tangent and sub-normal at this point are equal, then the area of ΔPTN is given by
View SolutionIf a point P is moving such that the lengths
of the tangents drawn form P to the circles
x2+y2+8x+12y+15=0and
x2+y2−4x−6y−12=0 are equal
then find the equation of the locus of P
View Solution
