Any contingent of 616 members is to march behind an army band of 32 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march?
To find the maximum number of columns in which both the contingent of 616 members and the army band of 32 members can march, we need to calculate the Highest Common Factor (HCF) of the two numbers. We will use the Euclidean division method to find the HCF.
1. Identify the two numbers: We have 616 (members of the contingent) and 32 (members of the army band).
2. Apply the Euclidean algorithm:
- We start by dividing the larger number (616) by the smaller number (32).
- Perform the division:
616÷32=19(quotient)
32×19=608
Remainder=616−608=8
- So, we can express this as:
616=32×19+8
3. Repeat the process: Now, we take the divisor (32) and the remainder (8) and apply the division again.
- Divide 32 by 8:
32÷8=4(quotient)
8×4=32
Remainder=32−32=0
- This can be expressed as:
32=8×4+0
4. Conclusion: Since the remainder is now 0, we stop here. The last non-zero remainder is 8, which means:
HCF(616,32)=8
5. Final Answer: The maximum number of columns in which both groups can march is 8.
- We start by dividing the larger number (616) by the smaller number (32).
- Perform the division:
616÷32=19(quotient)
32×19=608
Remainder=616−608=8
- So, we can express this as:
616=32×19+8
- Divide 32 by 8:
32÷8=4(quotient)
8×4=32
Remainder=32−32=0
- This can be expressed as:
32=8×4+0
HCF(616,32)=8
Topper's Solved these Questions
Similar Questions
An army contingent of 616 members is to march behind an army band of 32 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march?
View SolutionAn army contingent of 616 members is to march behind an army band of 32 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march ?
View SolutionAn army contingent of 616 members is to march behind an army band of 32 members in a parade. The two groups are to march in the same number of columns. What is the maximum number of columns in which they can march?
View SolutionCadets are marching in a parade. There are 5 cadets in a row. What is the rule which gives the number of cadets, given the number of rows? (Use n for the number of rows.)
View SolutionRead the bar graph given in Figure and answer the following questions: What is the information given by the bar graph? What is the number of families having 6 members? How many member per family are there in the maximum number of families? Also tell the number of such families. What are the number of members per family for which the number of families are equal? Also, tell the number of such families?
View SolutionA group of 2n students consisting of n boys and n girls are to be arranged in a row such that adjacent members are of opposite sex. The number of ways in which this can be done is
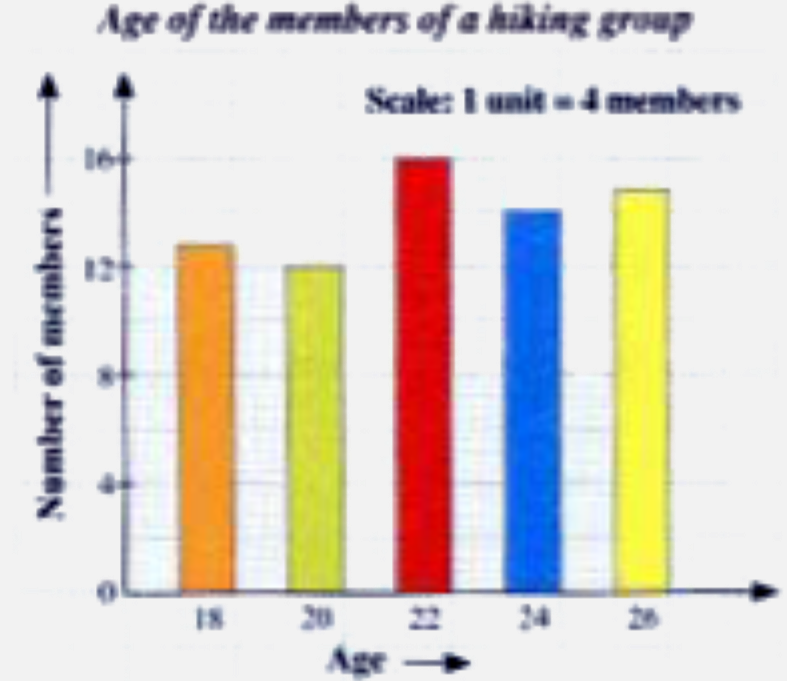
View SolutionThe given graph represents the age and number of members of a hiking group who are planning an excursion. Study the graph and answer the following question.
Which age group has the maximum members in the hiking gruoup?
 View Solution
View SolutionTo each element of the set S = {1, 2, 3.....1000}, a colour is assigned. Suppose that for any two elements a, b of S, if 15 divides a + b they are both assigned the same colour. What is the maximum possible number of distinct colours used
View SolutionWhich of the following statements related to the modern periodic table is incorrect?
a.The p-block has 6 columns, because a maximum of 6 electrons can occupy all the orbitals in a p-shell.
b.The d-blocks has 8 columns, because a maximum of 8 electrons can occupy all the orbitals in a d-subshell.
c.Each block contains a number of columns equal to the number of electrons that can occupy that subshell.
d.The block indicates value of azimuthal quantum number (l) for the last subshell that received electrons in building up the electronic configuration.View SolutionTo each element of the set S = {1, 2, 3.....1000}, a colour is assigned. Suppose that for any two elements a, b of S, if 15 divides a + b they are both assigned the same colour. What is the maximum possible number of distinct colours used
View Solution

