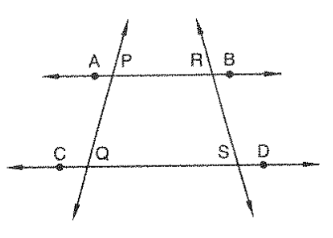
In Figure , name the following Figure Five line segments (ii) Five rays Four collinear points Two pairs of non-intersecting line segments.
Step-by-Step Solution
1. Identify Five Line Segments:
- In the given figure, we need to identify five line segments.
- The line segments present are:
- AB
- CD
- AC
- PQ
- DS
- Therefore, the five line segments are: AB,CD,AC,PQ,DS.
2. Identify Five Rays:
- Next, we need to identify five rays from the figure.
- The rays present are:
- PA
- PR
- RB
- DC
- QS
- Therefore, the five rays are: PA,PR,RB,DC,QS.
3. Identify Four Collinear Points:
- We need to find four collinear points in the figure.
- Upon examining the figure, we can see that the points C,D,Q, and S are collinear.
- Therefore, the four collinear points are: C,D,Q,S.
4. Identify Two Pairs of Non-Intersecting Line Segments:
- Finally, we need to identify two pairs of non-intersecting line segments.
- The first pair of non-intersecting line segments is AB and CD.
- The second pair of non-intersecting line segments is PB and LS.
- Therefore, the two pairs of non-intersecting line segments are: (AB,CD) and (PB,LS).
Summary of Answers:
- Five line segments: AB,CD,AC,PQ,DS
- Five rays: PA,PR,RB,DC,QS
- Four collinear points: C,D,Q,S
- Two pairs of non-intersecting line segments: (AB,CD) and (PB,LS)
- In the given figure, we need to identify five line segments.
- The line segments present are:
- AB
- CD
- AC
- PQ
- DS
- Therefore, the five line segments are: AB,CD,AC,PQ,DS.
- Next, we need to identify five rays from the figure.
- The rays present are:
- PA
- PR
- RB
- DC
- QS
- Therefore, the five rays are: PA,PR,RB,DC,QS.
- We need to find four collinear points in the figure.
- Upon examining the figure, we can see that the points C,D,Q, and S are collinear.
- Therefore, the four collinear points are: C,D,Q,S.
- Finally, we need to identify two pairs of non-intersecting line segments.
- The first pair of non-intersecting line segments is AB and CD.
- The second pair of non-intersecting line segments is PB and LS.
- Therefore, the two pairs of non-intersecting line segments are: (AB,CD) and (PB,LS).
- Five line segments: AB,CD,AC,PQ,DS
- Five rays: PA,PR,RB,DC,QS
- Four collinear points: C,D,Q,S
- Two pairs of non-intersecting line segments: (AB,CD) and (PB,LS)
Topper's Solved these Questions
Similar Questions
In Fig., name: Five line segments. (ii) Five rays (iii) Non-intersection line segments
View SolutionDefine the following terms: Line segment
View SolutionIn the adjoining figure, name :
(i) four points
(ii) four line segments
(iii) four rays

View SolutionConsider the following figure and name:
(a) A pair of parallel lines
(b) Concurrent lines and point of concurrency
( c ) Three sets of collinear points
(d) Six pairs of intersecting lines.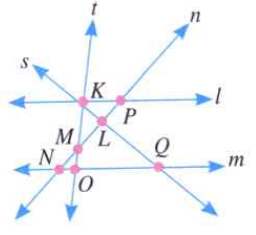
View SolutionUse the figure to name :(a) Line containing point E.(b) Line passing through A.(c) Line on which O lies(d) Two pairs of intersecting lines
View SolutionState which of the following pairs of line segments or rays appear to be parallel :
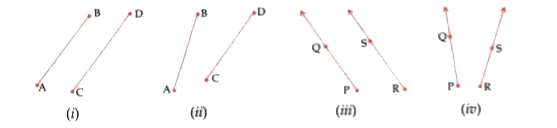
View SolutionIdentify rays and line segments form the following figures:
View SolutionIdentify rays and line segments form the following figures: Figure (ii) Figure (iii) Figure (iv) Figure
View SolutionWrite the truth value (T/F) of each of the following statements: Two lines intersect in a point. Two lines may intersect in two points. A segment has no length. Two distinct points always determine a line. Every ray has a finite length. A ray has one end-point only. A segment has one end-point only. The ray AB is same as ray BA Only a single line may pass through a given point. Two lines are coincident if they have only one point in common.
View SolutionName the four lines segments in the given figure. Identify the line segments which have a common end point. Name the common end points

View Solution
