If the mode of the data: 64, 60, 48, x, 43, 48, 43, 34 is 43, then x+3= (a) 44 (b) 45 (c) 46 (d) 48
To solve the problem step by step, we need to find the value of x such that the mode of the given data set is 43. The data set is:
64,60,48,x,43,48,43,34
Step 1: Understand the concept of mode
The mode of a data set is the number that appears most frequently. In this case, we are given that the mode is 43.
Step 2: Count the occurrences of each number
Let's list the numbers and their occurrences, excluding x:
- 64: 1 time
- 60: 1 time
- 48: 2 times
- 43: 2 times
- 34: 1 time
Step 3: Analyze the current mode
Currently, the numbers 43 and 48 both appear 2 times. For 43 to remain the mode, it must appear more frequently than any other number.
Step 4: Determine the value of x
To ensure that 43 is the mode, x must be equal to 43. If x=43, the occurrences would be:
- 64: 1 time
- 60: 1 time
- 48: 2 times
- 43: 3 times (because we now have an additional 43 from x)
- 34: 1 time
Now, 43 appears 3 times, which is more than any other number.
Step 5: Calculate x+3
Since we found that x=43, we can now calculate:
x+3=43+3=46
Conclusion
Thus, the value of x+3 is:
46
Answer: (c) 46
---
The mode of a data set is the number that appears most frequently. In this case, we are given that the mode is 43.
Let's list the numbers and their occurrences, excluding x:
- 60: 1 time
- 48: 2 times
- 43: 2 times
- 34: 1 time
Currently, the numbers 43 and 48 both appear 2 times. For 43 to remain the mode, it must appear more frequently than any other number.
To ensure that 43 is the mode, x must be equal to 43. If x=43, the occurrences would be:
- 60: 1 time
- 48: 2 times
- 43: 3 times (because we now have an additional 43 from x)
- 34: 1 time
Since we found that x=43, we can now calculate:
Thus, the value of x+3 is:
Topper's Solved these Questions
Similar Questions
Find the mean deviation from the mean for the following data:
38, 70, 48, 40, 42, 55, 63, 46, 54, 44
View SolutionIn Fig.72, if AB∣∣CD and AE∣∣BD, then the value of x
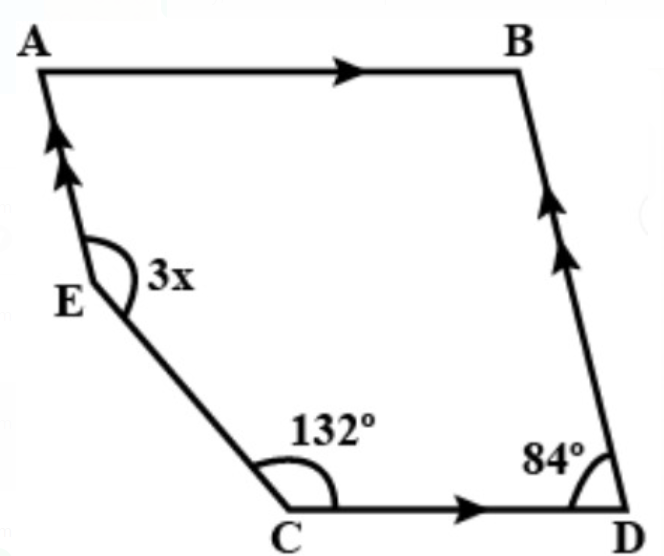 is
(a) 38 (b) 48
(b) 58 (d) 68
is
(a) 38 (b) 48
(b) 58 (d) 68
View SolutionIf the mean of observations is then 43 (b) 42 44 (d) 45
View SolutionIn Figure, for which value of x is l1l2 ? 37 (b) 43 (c) 45 (d) 47
View Solution2+310+4100= ? (a) 2.304 (b) 0.403 (c) 2.34 (d) 2.43
View SolutionIn the given figure, the value of x is
(a) 720
(b) 500
(c) 580
(d) 480
View SolutionThe ratio of two numbers is 3:4 and their HCF is 4. Their LCM is
(a) 12
(b) 16
(c) 24
(d) 48
View SolutionThe weights (in kg.) of 15 students of a class are:38, 42, 35, 37, 45, 50, 32, 43, 43, 40, 36, 38, 43, 38, 47(i) Find the mode and median of this data.(ii) Is there more than one mode?
View SolutionThe value of 0.23+ 0.22 is (a) 0.45 (b) 0.43 (c) 0.47 (d) 0.41
View SolutionFind the median of the following observations: 46, 64, 87, 41, 58, 77, 35, 90, 55, 92, 33. If 92 is replaced by 99 and 41 by 43 in the above data, find the new median?
View Solution
