In figure, ABCD is a parallelogram, AE perpendicular to DC and CF perpendicular to AD. If AB = 16cm, AE = 8cm and CF = 10cm, Find AD.
To find the length of side AD in the parallelogram ABCD, we can follow these steps:
1. Identify the Given Information:
- AB = 16 cm (one side of the parallelogram)
- AE = 8 cm (height from A to DC)
- CF = 10 cm (height from C to AD)
2. Use the Property of Parallelograms:
- In a parallelogram, opposite sides are equal. Therefore, CD = AB = 16 cm.
3. Calculate the Area of the Parallelogram:
- The area of a parallelogram can be calculated using the formula:
Area=Base×Height
- Using CD as the base and AE as the height:
Area=CD×AE=16cm×8cm=128cm2
4. Express the Area Using a Different Base and Height:
- Now, we can also express the area using AD as the base and CF as the height:
Area=AD×CF
- Substituting the known height:
128cm2=AD×10cm
5. Solve for AD:
- Rearranging the equation to find AD:
AD=128cm210cm=12.8cm
Final Answer:
- The length of side AD is 12.8 cm.
- AB = 16 cm (one side of the parallelogram)
- AE = 8 cm (height from A to DC)
- CF = 10 cm (height from C to AD)
- In a parallelogram, opposite sides are equal. Therefore, CD = AB = 16 cm.
- The area of a parallelogram can be calculated using the formula:
Area=Base×Height
- Using CD as the base and AE as the height:
Area=CD×AE=16cm×8cm=128cm2
- Now, we can also express the area using AD as the base and CF as the height:
Area=AD×CF
- Substituting the known height:
128cm2=AD×10cm
- Rearranging the equation to find AD:
AD=128cm210cm=12.8cm
- The length of side AD is 12.8 cm.
Topper's Solved these Questions
Similar Questions
ABCD is a parallelogram where DE is perpendicular to AB. If BD= 17 cm, AE = 15 cm, and area of ΔADE=60sq. cm, find the perimeter of ABCD.

View SolutionIn the given figure, AB and DE are perpendiculars to BC. If AB = 9 cm, DE = 3 cm and AC = 24 cm, calculate AD.

View SolutionIn a pentagon ABCDE , DP is drawn perpendicular to AB and is perpendicular to CE also at point Q . If AP = BP = 12 cm EQ = CQ = 8 cm . DE = DC = 10 cm and DP = 18 cm . Find the area of the pentagon ABCDE .
View SolutionABCD is a parallologram. From C perpendiculars CS and CT are drpopped upon AB and AD, respectively. Given AB=16cm,CS=9cm,andCT=8cm, find BC.
View SolutionABC is a right angled triangle with ∠ABC=90∘, D is any point on AB and DE is perpendicular to AC.
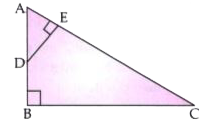
(ii) If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.
View SolutionIn the figure, ABCDE is a pentagon with BE∣∣CD and BC∣∣DE. BC is perpendicular to CD. AB= 5cm, AE=5cm, BE= 7cm, BC= x-y and CD= x+y. If the perimeter of ABCDE is 27cm. find the value of x and y, given x,y,≠0
View SolutionABC is a right angled triangle with ABC=90∘. D is any point on AB and DE is perpendicular to AC. Prove that:

If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.
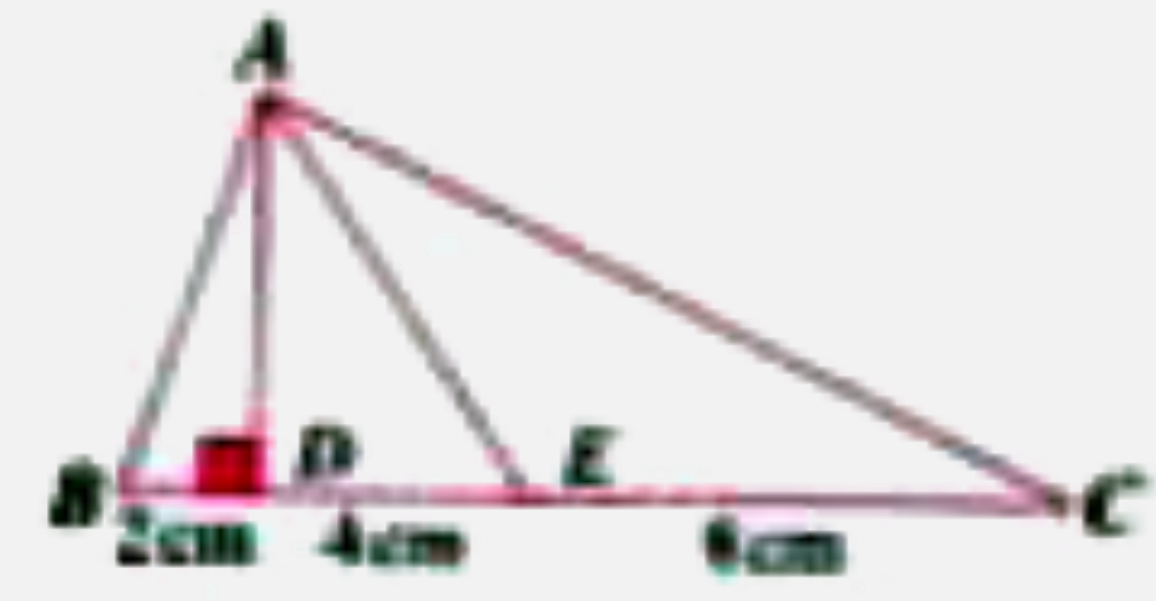
View SolutionA triangle ABC is such that AD is perpendicular to BC and E is a point on DC. Also. Given that BD=2cm,DE=4cm,andEC=8cm. Find the ratio of the areas of ΔABC , ΔADEandΔAEC.
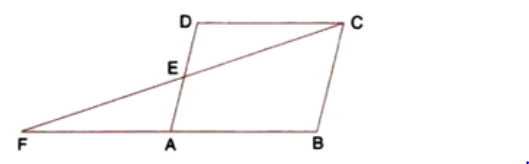
View SolutionThe given figure shows a parallelogram ABCD. E is a point in AD and CE produced meets BA produced at point F. If AE = 4 cm, AF = 8 cm and AB = 12 cm, find the perimeter of the parallelogram ABCD.
View SolutionIn ΔABC,AD and CE are perpendiculars from A and C on the opposite sides. If AD=6cm.BC=14cm,AB=10cm,find CE.
View Solution