If h,c,V are respectively the height, the curved surface and the volume of a cone, prove that 3πVh3−C2h2+9V2=0.
To prove that 3πVh3−C2h2+9V2=0, where h is the height, C is the curved surface area, and V is the volume of a cone, we will follow these steps:
Step 1: Write the formulas for the curved surface area and volume of a cone.
The formulas for the curved surface area C and volume V of a cone are given by:
C=πrl
V=13πr2h
where r is the radius and l is the slant height of the cone.
Step 2: Express C and V in terms of r and h.
From the formulas above, we have:
C=πrl
V=13πr2h
Step 3: Substitute l using the Pythagorean theorem.
The slant height l can be expressed in terms of r and h using the Pythagorean theorem:
l=√r2+h2
Thus, we can rewrite C as:
C=πr√r2+h2
Step 4: Substitute C and V into the equation 3πVh3−C2h2+9V2.
Now, substituting V and C into the left-hand side of the equation:
3πVh3=3π(13πr2h)h3=π2r2h4
C2=(πr√r2+h2)2=π2r2(r2+h2)
9V2=9(13πr2h)2=13π2r4h2
Step 5: Substitute these into the equation.
Now substituting these into the equation:
π2r2h4−π2r2(r2+h2)h2+13π2r4h2=0
Step 6: Simplify the equation.
Distributing and simplifying:
π2r2h4−π2r2h2(r2+h2)+13π2r4h2=0
π2r2h4−π2r2h2r2−π2r2h4+13π2r4h2=0
The h4 terms cancel out:
−π2r2h2r2+13π2r4h2=0
Factoring out π2h2:
π2h2(−r2+13r4)=0
Step 7: Set the factor equal to zero.
Thus, we have:
−r2+13r4=0
This simplifies to:
r4−3r2=0
Factoring gives:
r2(r2−3)=0
Thus, r2=0 or r2=3.
Conclusion
Since r2=0 is not a valid solution for a cone, we conclude that the equation holds true, proving:
3πVh3−C2h2+9V2=0
The formulas for the curved surface area C and volume V of a cone are given by:
C=πrl
V=13πr2h
where r is the radius and l is the slant height of the cone.
From the formulas above, we have:
C=πrl
V=13πr2h
The slant height l can be expressed in terms of r and h using the Pythagorean theorem:
l=√r2+h2
Thus, we can rewrite C as:
C=πr√r2+h2
Now, substituting V and C into the left-hand side of the equation:
3πVh3=3π(13πr2h)h3=π2r2h4
C2=(πr√r2+h2)2=π2r2(r2+h2)
9V2=9(13πr2h)2=13π2r4h2
Now substituting these into the equation:
π2r2h4−π2r2(r2+h2)h2+13π2r4h2=0
Distributing and simplifying:
π2r2h4−π2r2h2(r2+h2)+13π2r4h2=0
π2r2h4−π2r2h2r2−π2r2h4+13π2r4h2=0
The h4 terms cancel out:
−π2r2h2r2+13π2r4h2=0
Factoring out π2h2:
π2h2(−r2+13r4)=0
Thus, we have:
−r2+13r4=0
This simplifies to:
r4−3r2=0
Factoring gives:
r2(r2−3)=0
Thus, r2=0 or r2=3.
Since r2=0 is not a valid solution for a cone, we conclude that the equation holds true, proving:
3πVh3−C2h2+9V2=0
Topper's Solved these Questions
- SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDERBOOK - RD SHARMA ENGLISHCHAPTER - SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDEREXERCISE - All Questions
133 Videos
- SURFACE AREA AND VOLUME OF A SPHERE BOOK - RD SHARMA ENGLISHCHAPTER - SURFACE AREA AND VOLUME OF A SPHERE EXERCISE - All Questions
140 Videos
Similar Questions
Knowledge Check
V=13π(d2)2h
A circle of rubber with a constant diameter d is placed on a table, its perimeter is anchored to the table and a string is attached to its centre. When the string is pulled upwards, a cone is formed with height h and volume V. The relationship between d, h, and V is represented above. Which of the following statements must be true ?
I. As the volume of the cone decreases, the height also decreases.
II. If the diameter of the base of the cone is 6 centimeters, the height can be determined by dividing the volume by 3π.
III. If the height of the conc triples, the volume must also triple.AI onlyBI and II onlyCII and III onlyDI, II, and III
Show that the height of the cylinder of maximum volume that can be inscribed in a cone of height h is 13h
View SolutionA solid consists of a circular cylinder with an exact fitting right circular cone placed at the top. The height of the cone is h . If the total volume of the solid is 3 times the volume of the cone, then the height of the circular cylinder is (a) 2h (b) 2h3 (c) 3h2 (d) 4h
View SolutionA solid consists of a circular cylinder with an exact fitting right circular cone placed at the top. The height of the cone is h . If the total volume of the solid is 3 times the volume of the cone, then the height of the circular cylinder is 2h (b) 2h3 (c) 3h2 (d) 4h
View SolutionIf the height and radius of a cone of volume V are doubled, then the volume of the cone, is (a) 3 V (b) 4 V (c) 6 V (d) 8 V
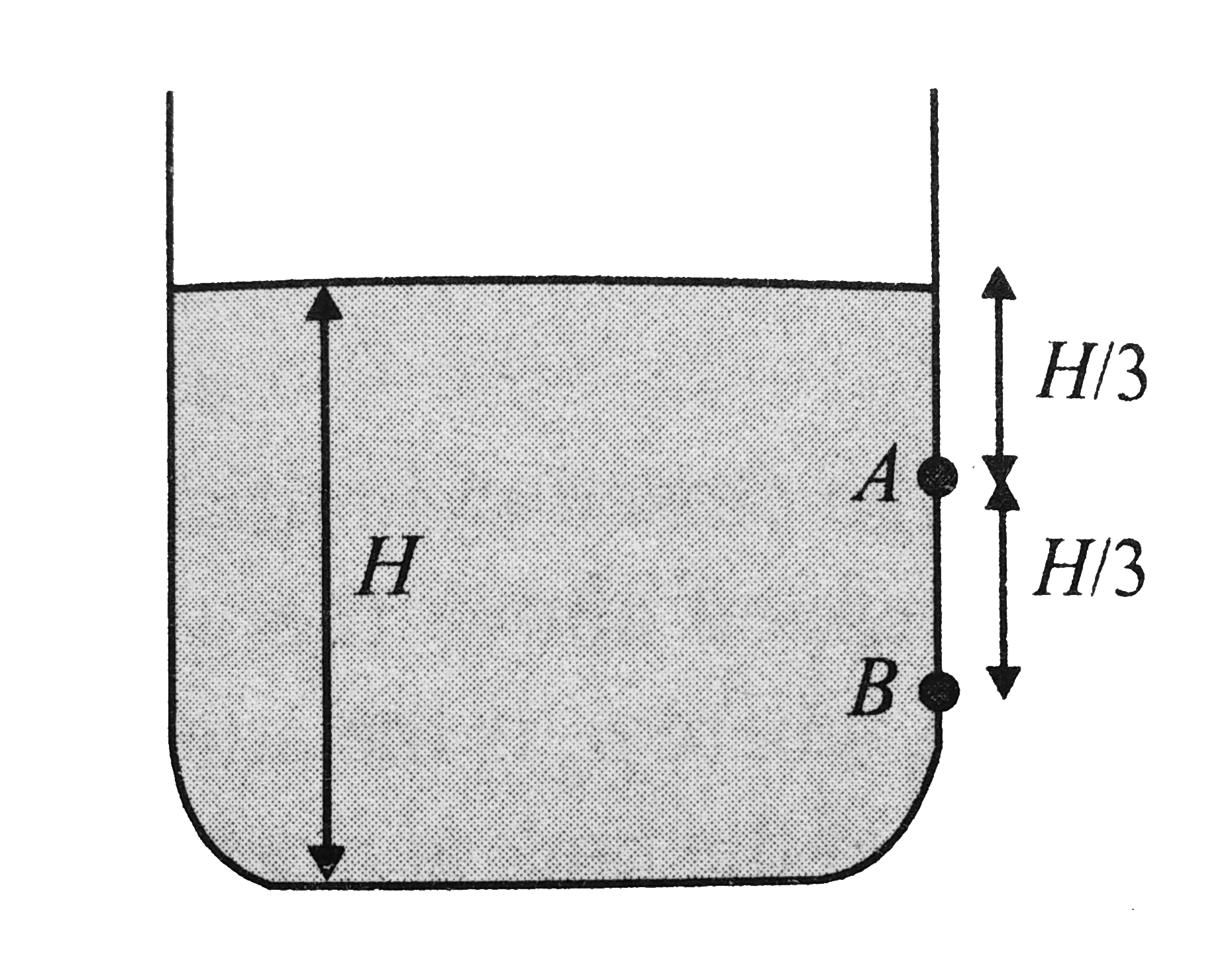
View SolutionThe area of two holes A and B are 2a and a, respectively, The holes are at height (H/3)and(2H/3) from the surface of water. Find the correct option(s):
View SolutionFrom a given solid cone of height H, another inverted cone is carved whose height is h such that its volume is maximum. Then the ratio Hh is
View SolutionThe difference between the value of Cp of H2 and Cv of He will be
View Solution
