A hemispherical bowl is made of steel 0.5 cm thick. The inside radius of the bowl is 4cm.
To solve the problem of finding the volume of steel used in making a hemispherical bowl, we will follow these steps:
Step 1: Identify the given values
- Inside radius of the bowl (r) = 4 cm
- Thickness of the bowl = 0.5 cm
Step 2: Calculate the external radius
The external radius (R) of the bowl can be calculated by adding the thickness to the inside radius:
R=r+thickness=4cm+0.5cm=4.5cm
Step 3: Use the formula for the volume of a hemispherical shell
The volume of the steel used in making the bowl is equal to the volume of the outer hemisphere minus the volume of the inner hemisphere. The formula for the volume of a hemisphere is:
V=23πr3
Thus, the volume of the steel used (V_steel) can be expressed as:
Vsteel=Vouter−Vinner=23πR3−23πr3
This can be simplified to:
Vsteel=23π(R3−r3)
Step 4: Substitute the values into the formula
Now, substituting the values of R and r into the formula:
Vsteel=23π(4.53−43)
Step 5: Calculate R3 and r3
Calculate 4.53 and 43:
4.53=91.125and43=64
Step 6: Calculate the difference
Now, calculate the difference:
R3−r3=91.125−64=27.125
Step 7: Substitute back into the volume formula
Substituting this difference back into the volume formula:
Vsteel=23π(27.125)
Step 8: Use π≈227 for calculation
Now, substituting π with 227:
Vsteel=23×227×27.125
Step 9: Calculate the final volume
Calculating this gives:
Vsteel=4421×27.125≈56.83cm3
Final Answer
The volume of steel used in making the bowl is approximately 56.83 cm³.
---
- Inside radius of the bowl (r) = 4 cm
- Thickness of the bowl = 0.5 cm
The external radius (R) of the bowl can be calculated by adding the thickness to the inside radius:
R=r+thickness=4cm+0.5cm=4.5cm
The volume of the steel used in making the bowl is equal to the volume of the outer hemisphere minus the volume of the inner hemisphere. The formula for the volume of a hemisphere is:
V=23πr3
Thus, the volume of the steel used (V_steel) can be expressed as:
Vsteel=Vouter−Vinner=23πR3−23πr3
This can be simplified to:
Vsteel=23π(R3−r3)
Now, substituting the values of R and r into the formula:
Vsteel=23π(4.53−43)
Calculate 4.53 and 43:
4.53=91.125and43=64
Now, calculate the difference:
R3−r3=91.125−64=27.125
Substituting this difference back into the volume formula:
Vsteel=23π(27.125)
Now, substituting π with 227:
Vsteel=23×227×27.125
Calculating this gives:
Vsteel=4421×27.125≈56.83cm3
The volume of steel used in making the bowl is approximately 56.83 cm³.
Topper's Solved these Questions
- SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDER CONEBOOK - RD SHARMA ENGLISHCHAPTER - SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDER CONEEXERCISE - All Questions
129 Videos
- TABULAR REPRESENTATION OF STATISTICAL DATA BOOK - RD SHARMA ENGLISHCHAPTER - TABULAR REPRESENTATION OF STATISTICAL DATA EXERCISE - All Questions
70 Videos
Similar Questions
A hemispherical bowl is made of steel, 0.5 cm thick. The inside radius of the bowl is 4 cm. Find the volume of steel used in making the bowl.
View SolutionA hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
View SolutionA hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
View SolutionA hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
View SolutionA hemispherical tank is made up of an iron sheet 1 cm thick. If the inner radius is 1 m,then find the volume of the iron used to make the tank
View SolutionA hemispherical tank is made up of an iron sheet 1 cm thick. If the inner radius is 1 m, then find the volume of the iron used to make the tank
View SolutionA hemispherical tank is made up of an iron sheet 1 cm thick. If the inner radius is 1 m, then find the volume of the iron used to make the tank
View SolutionA particle is projected horizontally as shown from the rim of a large hemispherical bowl. The displacement of the particle when it strikes the bowl the first time is R. Find the velocity of the particle at that instant and the time taken.

View SolutionA hemispherical bowl made of brass has inner diameter 10.5 cm. Find the cost of tin-plating it on the inside at the rate of Rs 16 per100cm2.
View Solution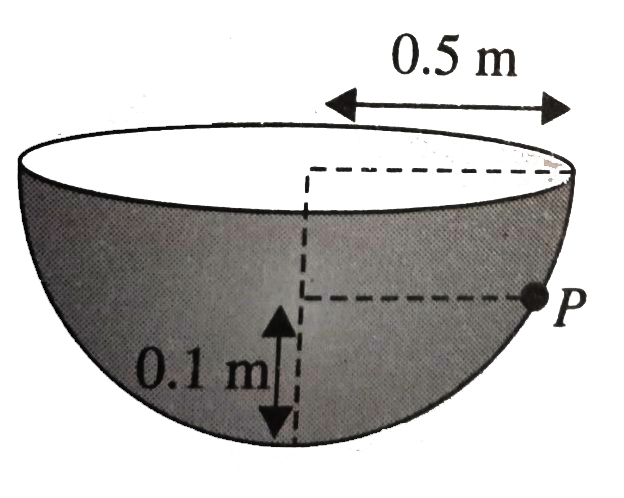
A small mass of 10 g lies in a hemispherical bowl of radius 0.5 m at a height of 0.1 m from the bottom of the bowl. The mass will be in equilibrius if the bowl rotates at an
View Solution
