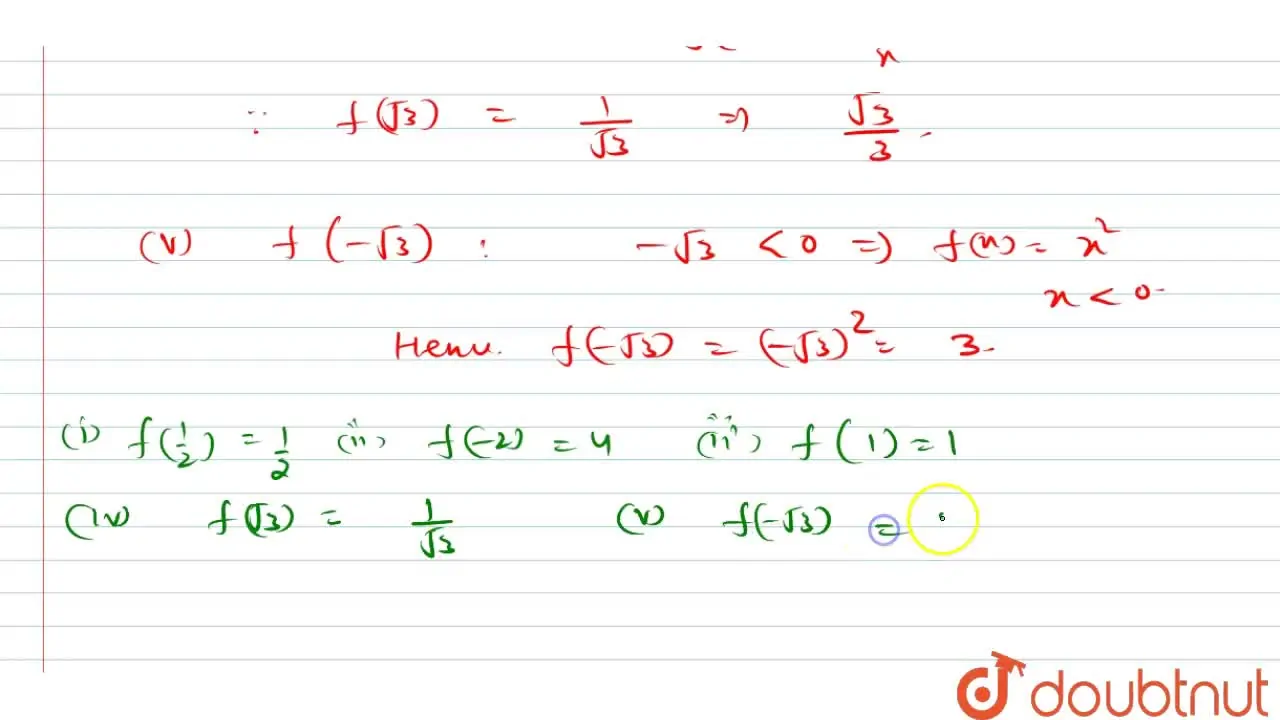
If f(x)=⎧⎪ ⎪⎨⎪ ⎪⎩x2whenx<0x when0≤x<11x whenx>1 Find : (i). f(1/2) (ii). f(−2) (iii). f(1) (iv). f(√3) (v). f(−√3)
Step by step video & image solution for If f(x)= {(x^2,"when" ,xlt0),( x ," when", 0lexlt1),( 1/x ," when", x gt1):} F i n d : (i). f(1//2) (ii). f(-2) (iii). f(1) (iv). f(sqrt(3)) (v). f(-sqrt(3)) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
If f(x)={3x−1 when x≤0x+1 when x>0, find f(-1) and f(0).
View SolutionIf f(x)=2x,thenf(0),f(1),f(2)...are in
View SolutionLet f(x)={(1+sinx),when 0≤x<π21, when x<0 . Find Rf'(0)
View SolutionIf f(x)={2x−1when x≤0x2when x>0,then find f(12)andf(−12)
View SolutionIf f(x)=⎧⎪⎨⎪⎩x, x<01, x=0x2, x>0, then find limx→0f(x) if exists.
View SolutionIf f(x)=√x2−2x+1, then f' (x) ?
View SolutionIf f:R→R is defined as:
f(x)=⎧⎪⎨⎪⎩2x+5,x>9x2−1,−9<x<9x−4,x<−9
then evaluate (i) f(2),(ii)f(10),(iii)f(−12)and(iv)f[f(3)].View SolutionIf f:R→R is defined as:
f(x)=⎧⎪⎨⎪⎩2x+1ifx>2x2−1if−2<x<22xifx<−2
then evaluate the following:
(i) f(1)
(ii) f(5)
(iii) f(−3)View SolutionIf f(x)=2x-5 , then evaluate the following:
(i) f(0)
(ii) f(7)
(iii) f(−3)View SolutionIf f:R→R defined by f(x)=⎧⎪⎨⎪⎩4x−1 for x>4x2−2 for −2≤x<43x+4 for x<−2
find f(5)+f(0)+f(−5)View Solution