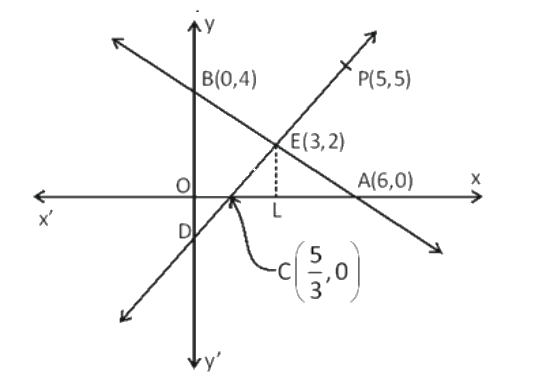
The line meets the x-axis at A and y-axis at B. The line through (5,5) perpendicular to AB meets the x-axis and the line AB at C and E respectively. If O is the origin of coordinates, find the area of figure OCEB.
To solve the problem step by step, we will follow these instructions:
Step 1: Find the coordinates of points A and B
The line given is 2x+3y=12.
Finding point A (where the line meets the x-axis):
To find the x-intercept (point A), set y=0:
2x+3(0)=12⟹2x=12⟹x=6
Thus, the coordinates of point A are A(6,0).
Finding point B (where the line meets the y-axis):
To find the y-intercept (point B), set x=0:
2(0)+3y=12⟹3y=12⟹y=4
Thus, the coordinates of point B are B(0,4).
Step 2: Determine the slope of line AB
The slope m of line AB can be calculated using the coordinates of points A and B:
m=y2−y1x2−x1=4−00−6=4−6=−23
Step 3: Find the slope of the line perpendicular to AB
The slope of the line perpendicular to AB is the negative reciprocal of the slope of AB:
m_{\perpendicular} = \frac{3}{2}
Step 4: Write the equation of the line through point (5, 5) with the perpendicular slope
Using point-slope form y−y1=m(x−x1):
y−5=32(x−5)
Expanding this:
y−5=32x−152
Rearranging gives:
3x−2y−5=0
Step 5: Find the intersection point E of the two lines
To find point E, we need to solve the equations of the two lines:
1. 2x+3y=12
2. 3x−2y−5=0
From the second equation, express y:
2y=3x−5⟹y=32x−52
Substituting this into the first equation:
2x+3(32x−52)=12
Simplifying:
2x+92x−152=12
Multiplying through by 2 to eliminate the fraction:
4x+9x−15=24⟹13x=39⟹x=3
Substituting x=3 back to find y:
y=32(3)−52=92−52=2
Thus, the coordinates of point E are E(3,2).
Step 6: Find the coordinates of point C (where the line meets the x-axis)
To find point C, set y=0 in the equation of the line 3x−2y−5=0:
3x−2(0)−5=0⟹3x=5⟹x=53
Thus, the coordinates of point C are C(53,0).
Step 7: Calculate the area of quadrilateral OCEB
The area of quadrilateral OCEB can be calculated as the sum of the areas of triangles OBE and OCE.
Area of triangle OBE:
Base OB=4 (from O(0,0) to B(0,4)) and height from E to OB (horizontal distance from E to y-axis):
Height=3 (x-coordinate of E)
Area of triangle OBE:
AreaOBE=12×Base×Height=12×4×3=6
Area of triangle OCE:
Base OC=53 and height from E to OC (vertical distance from E to x-axis):
Height=2 (y-coordinate of E)
Area of triangle OCE:
AreaOCE=12×Base×Height=12×53×2=53
Step 8: Total area of quadrilateral OCEB
Total Area=AreaOBE+AreaOCE=6+53=183+53=233
Thus, the area of the figure OCEB is 233 square units.
The line given is 2x+3y=12.
To find the x-intercept (point A), set y=0:
2x+3(0)=12⟹2x=12⟹x=6
Thus, the coordinates of point A are A(6,0).
To find the y-intercept (point B), set x=0:
2(0)+3y=12⟹3y=12⟹y=4
Thus, the coordinates of point B are B(0,4).
The slope m of line AB can be calculated using the coordinates of points A and B:
m=y2−y1x2−x1=4−00−6=4−6=−23
The slope of the line perpendicular to AB is the negative reciprocal of the slope of AB:
m_{\perpendicular} = \frac{3}{2}
Using point-slope form y−y1=m(x−x1):
y−5=32(x−5)
Expanding this:
y−5=32x−152
Rearranging gives:
3x−2y−5=0
To find point E, we need to solve the equations of the two lines:
1. 2x+3y=12
2. 3x−2y−5=0
2y=3x−5⟹y=32x−52
Substituting this into the first equation:
2x+3(32x−52)=12
Simplifying:
2x+92x−152=12
Multiplying through by 2 to eliminate the fraction:
4x+9x−15=24⟹13x=39⟹x=3
Substituting x=3 back to find y:
y=32(3)−52=92−52=2
Thus, the coordinates of point E are E(3,2).
To find point C, set y=0 in the equation of the line 3x−2y−5=0:
3x−2(0)−5=0⟹3x=5⟹x=53
Thus, the coordinates of point C are C(53,0).
The area of quadrilateral OCEB can be calculated as the sum of the areas of triangles OBE and OCE.
Base OB=4 (from O(0,0) to B(0,4)) and height from E to OB (horizontal distance from E to y-axis):
Height=3 (x-coordinate of E)
Area of triangle OBE:
AreaOBE=12×Base×Height=12×4×3=6
Base OC=53 and height from E to OC (vertical distance from E to x-axis):
Height=2 (y-coordinate of E)
Area of triangle OCE:
AreaOCE=12×Base×Height=12×53×2=53
Total Area=AreaOBE+AreaOCE=6+53=183+53=233
Topper's Solved these Questions
Similar Questions
The line 2x+3y=12 meets the coordinates axes at A and B respectively. The line through (5, 5) perpendicular to AB meets the coordinate axes and the line AB at C, D and E respectively. If O is the origin, then the area (in sq. units) of the figure OCEB is equal to
View SolutionThe line xa+yb=1 meets the axis of and y at A and B respectively and C is middle point of AB then
View Solution
Knowledge Check
The line xa−yb=1 cuts the x-axis at P. The equation of the line through P and perpendicular to the given line is
A(a) x+y=abB(b) x+y=a+bC(c) ax+by=a2D(d) bx+ay=b2
A line intersects the y-axis and x-axis at the points P and Q respectively. If (2, 5) is the mid-point of PQ, then find the coordinates of P and Q.
View SolutionThe line 3x - 4y + 12 = 0 meets x-axis at point A and y-axis at point B. Find :
equation of perpendicular bisector of line segment AB
View SolutionA line cuts the x-axis at A(7,0) and the y-axis at B(0,−5) A variable line PQ is drawn perpendicular to AB cutting the x-axis in P and the y-axis in Q. If AQ and BP intersect at R, find the locus of R.
View SolutionA line intersects the Y- axis and X-axis at the points P and Q, respectively. If (2,-5) is the mid- point of PQ, then the coordinates of P and Q are, respectively.
View SolutionThe line 3x - 4y + 12 = 0 meets x-axis at point A and y-axis at point B. Find :
the co-ordinates of A and B.
View SolutionThe straight line 2x+3y=24 meets the x-axis at P and the y-axis at Q. The perpendicular bisector of PQ meets the line through (-2,0) parallel to the y-axis at R. Find the area of the ΔPQR.
View SolutionA and B are two points on the x-axis and y-axis respectively. P(2, -3) is the mid point of AB. Find the
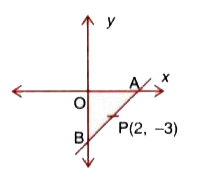
equation of line AB.
View Solution
