In class XI of a school 40% of the students study Mathematics and 30% study Biology. 10% of the class study both mathematics and biology. If a student is selected at random from the class, find the probability that he will be studying Mathematics or Biology or both.
To solve the problem, we need to find the probability that a randomly selected student studies Mathematics or Biology or both. We can use the principle of inclusion-exclusion for this.
1. Define the Events:
- Let A be the event that a student studies Mathematics.
- Let B be the event that a student studies Biology.
2. Given Probabilities:
- The probability that a student studies Mathematics, P(A)=40%=40100=0.4.
- The probability that a student studies Biology, P(B)=30%=30100=0.3.
- The probability that a student studies both Mathematics and Biology, P(A∩B)=10%=10100=0.1.
3. Use the Inclusion-Exclusion Principle:
- We want to find the probability that a student studies either Mathematics or Biology or both, which is represented as P(A∪B).
- The formula for the union of two events is:
P(A∪B)=P(A)+P(B)−P(A∩B)
4. Substitute the Values:
- Now, substitute the known values into the formula:
P(A∪B)=P(A)+P(B)−P(A∩B)=0.4+0.3−0.1
5. Calculate:
- Perform the calculation:
P(A∪B)=0.4+0.3−0.1=0.6
6. Final Answer:
- The probability that a randomly selected student studies Mathematics or Biology or both is P(A∪B)=0.6.
Summary:
The required probability is 0.6 or 60%.
---
- Let A be the event that a student studies Mathematics.
- Let B be the event that a student studies Biology.
- The probability that a student studies Mathematics, P(A)=40%=40100=0.4.
- The probability that a student studies Biology, P(B)=30%=30100=0.3.
- The probability that a student studies both Mathematics and Biology, P(A∩B)=10%=10100=0.1.
- We want to find the probability that a student studies either Mathematics or Biology or both, which is represented as P(A∪B).
- The formula for the union of two events is:
P(A∪B)=P(A)+P(B)−P(A∩B)
- Now, substitute the known values into the formula:
P(A∪B)=P(A)+P(B)−P(A∩B)=0.4+0.3−0.1
- Perform the calculation:
P(A∪B)=0.4+0.3−0.1=0.6
- The probability that a randomly selected student studies Mathematics or Biology or both is P(A∪B)=0.6.
The required probability is 0.6 or 60%.
Topper's Solved these Questions
Similar Questions
In a class of 60 students, 30 opted for Mathematics, 32 opted for Biology and 24 opted for both Mathematics and Biology. If one of these students is selected at random, find the probability that :
(i) The students opted for Mathematics or Biology.
(i) The students has opted neither Mathematics nor Biology.
(iii) The students has opted Mathematics but not Biology.View SolutionA class has 20 girls and 16 boys. One student is selected at random. Find the probability that the student is a girl.
View Solution
Knowledge Check
- Question 1 - Select One
A class has 12 boys and 4 girls. If three students are selected at random from the class,
what is the probability that all will be boys?A155B14C13D1128
In a school 25% students are failed in Mathematics, 15% in Physics and 5% students fail in both Mathematics and Physics. A student is selected at random.
(i) If he is fail in Physics, find the probability that he is fail in Mathematics.
(ii) If he is fail in Mathematics, find the probability that he in fail in Physics.
(iii) Find the probability that he is fail either in Mathematics or in Physics.View SolutionThere are 60% students in Maths and 30% in Biology. If 10% students are in both subjects. Find the probability that a randomly selected student has Maths or Biology.
View SolutionIn a class, 5% of the boys and 10% of the girls have an IQ of more than 150. In this class 60% of the students are boys. If a student is selected at random and found to have an IQ of more than 150, find the probability that the student is a boy.
View SolutionIn a medical examination of students of a class, the following blood groups are recorded.
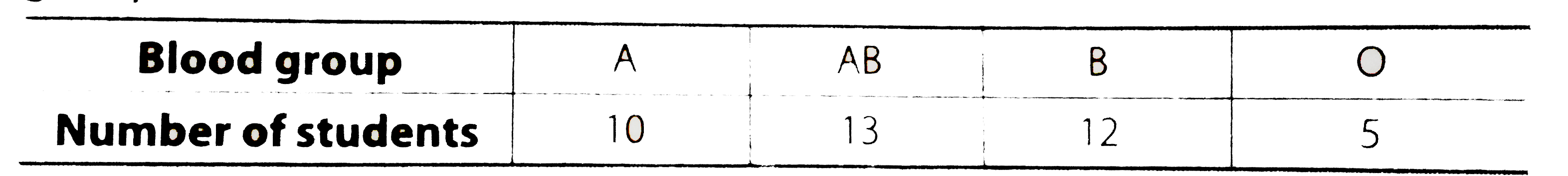
A student is selected at random from the class. The probability that he/she has blood group B, isView SolutionIn a class of 50 students, 20 are boys and rest are girls. A student is selected at random. Find the probability of selecting a boy.
View SolutionA class consists of 50 students out of which there are 10 girls. In the class 2 girls and 5 boys are rank holders in an examination. If a student is selected at random from the class and is found to be a rank holder, what is the probability that the student selected is a girl?
View SolutionIn a class of 125 students 70 passed in Mathematics, 55 in statistics, and 30 in both. Then find the probability that a student selected at random from the class has passed in only one subject.
View Solution

