Let f:[−π2, π2]→A be defined by f(x)=sinx . If f is a bijection, write set A .
To solve the problem, we need to determine the set A such that the function f(x)=sinx is a bijection from the interval (−π2,π2) to A.
1. Understand the Function and Its Domain:
The function given is f(x)=sinx, and the domain is (−π2,π2).
2. Determine the Range of the Function:
To find the set A, we need to determine the range of the sine function over the specified interval. The sine function is continuous and monotonically increasing in the interval (−π2,π2).
3. Calculate the Values at the Endpoints:
- At x=−π2:
f(−π2)=sin(−π2)=−1
- At x=π2:
f(π2)=sin(π2)=1
4. Identify the Range:
Since f(x) increases from −1 to 1 as x moves from −π2 to π2, the range of f(x) is:
(−1,1)
5. Set A:
Since f is a bijection, the range must equal the codomain A. Therefore, we can conclude that:
A=(−1,1)
Final Answer:
The set A is:
A=(−1,1)
The function given is f(x)=sinx, and the domain is (−π2,π2).
To find the set A, we need to determine the range of the sine function over the specified interval. The sine function is continuous and monotonically increasing in the interval (−π2,π2).
- At x=−π2:
f(−π2)=sin(−π2)=−1
- At x=π2:
f(π2)=sin(π2)=1
Since f(x) increases from −1 to 1 as x moves from −π2 to π2, the range of f(x) is:
(−1,1)
Since f is a bijection, the range must equal the codomain A. Therefore, we can conclude that:
A=(−1,1)
The set A is:
A=(−1,1)
Topper's Solved these Questions
Similar Questions
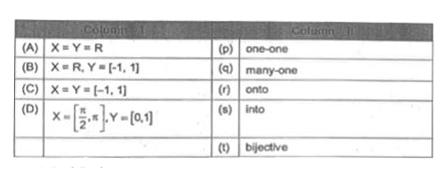
Let f:X→Y be a function defined by f(x)=sinx.
 View Solution
View SolutionLet f:{−π2, π2}→R be a function defined by f(x)=cos[x]. Write range (f) .
View SolutionIf f:R−R is defined by f(x)=2x+7. Prove that f is a bijection. Also, find the inverse of f.
View SolutionClassify f:R→R , defined by f(x)=sinx as injection, surjection or bijection.
View SolutionIf f:R→ is defined by f(x)=2x+7. Prove that f is a bijection. Also, find the inverse of f.
View SolutionLet f:[−π/2,π/2]→[−1,1] where f(x)=sinx. Find whether f(x) is one-one or not.
View SolutionLet f:[0,4π]→[0,π] be defined by f(x)=cos−1(cosx). The number of points x∈[0,4π] 4satisfying the equation f(x)=10−x10 is
View SolutionLet f:[0,4π]→[0,π] be defined by f(x)=cos−1(cosx). The number of points x∈[0,4π] 4satisfying the equation f(x)=10−x10 is
View SolutionLet f:[0,4π]→[0,π] be defined by f(x)=cos−1(cosx). The number of points x∈[0,4π] 4satisfying the equation f(x)=10−x10 is
View SolutionLet f:[0,4π]→[0,π] be defined by f(x)=cos−1(cosx). The number of points x∈[0,4π] 4satisfying the equation f(x)=10−x10 is
View Solution

