Prove that f(x)=ax+b , where a, b are constants and a<0 is a decreasing function on R .
To prove that the function f(x)=ax+b is a decreasing function on R given that a<0, we can follow these steps:
Step 1: Define the function
We start with the function:
f(x)=ax+b
where a and b are constants, and a<0.
Step 2: Find the derivative of the function
To determine if the function is decreasing, we need to find its derivative:
f′(x)=ddx(ax+b)
Calculating the derivative, we get:
f′(x)=a
Step 3: Analyze the derivative
Since a is a constant and we know from the problem statement that a<0, we can conclude:
f′(x)<0
Step 4: Apply the condition for decreasing functions
A function is considered decreasing on an interval if its derivative is less than or equal to zero throughout that interval. In this case, since f′(x)=a<0 for all x∈R, we can conclude that:
f′(x)<0for all x∈R
Step 5: Conclusion
Since the derivative f′(x) is negative for all x∈R, we conclude that the function f(x)=ax+b is a decreasing function on R.
Summary
Thus, we have proved that if a<0, then f(x)=ax+b is a decreasing function on R.
---
We start with the function:
f(x)=ax+b
where a and b are constants, and a<0.
To determine if the function is decreasing, we need to find its derivative:
f′(x)=ddx(ax+b)
Calculating the derivative, we get:
f′(x)=a
Since a is a constant and we know from the problem statement that a<0, we can conclude:
f′(x)<0
A function is considered decreasing on an interval if its derivative is less than or equal to zero throughout that interval. In this case, since f′(x)=a<0 for all x∈R, we can conclude that:
f′(x)<0for all x∈R
Since the derivative f′(x) is negative for all x∈R, we conclude that the function f(x)=ax+b is a decreasing function on R.
Thus, we have proved that if a<0, then f(x)=ax+b is a decreasing function on R.
Topper's Solved these Questions
Similar Questions
A function has the form f(x)=ax+b, where a and b are constants. If f(2)=1 and f(−3)=11, the function is defined by
View SolutionLet f(x)=ax2−b|x|, where aandb are constants. Then at x=0,f(x) has a maxima whenever a>0,b>0 a maxima whenever a>0,b<0 minima whenever a>0,b<0 neither a maxima nor a minima whenever a>0,b<0
View Solution
Knowledge Check
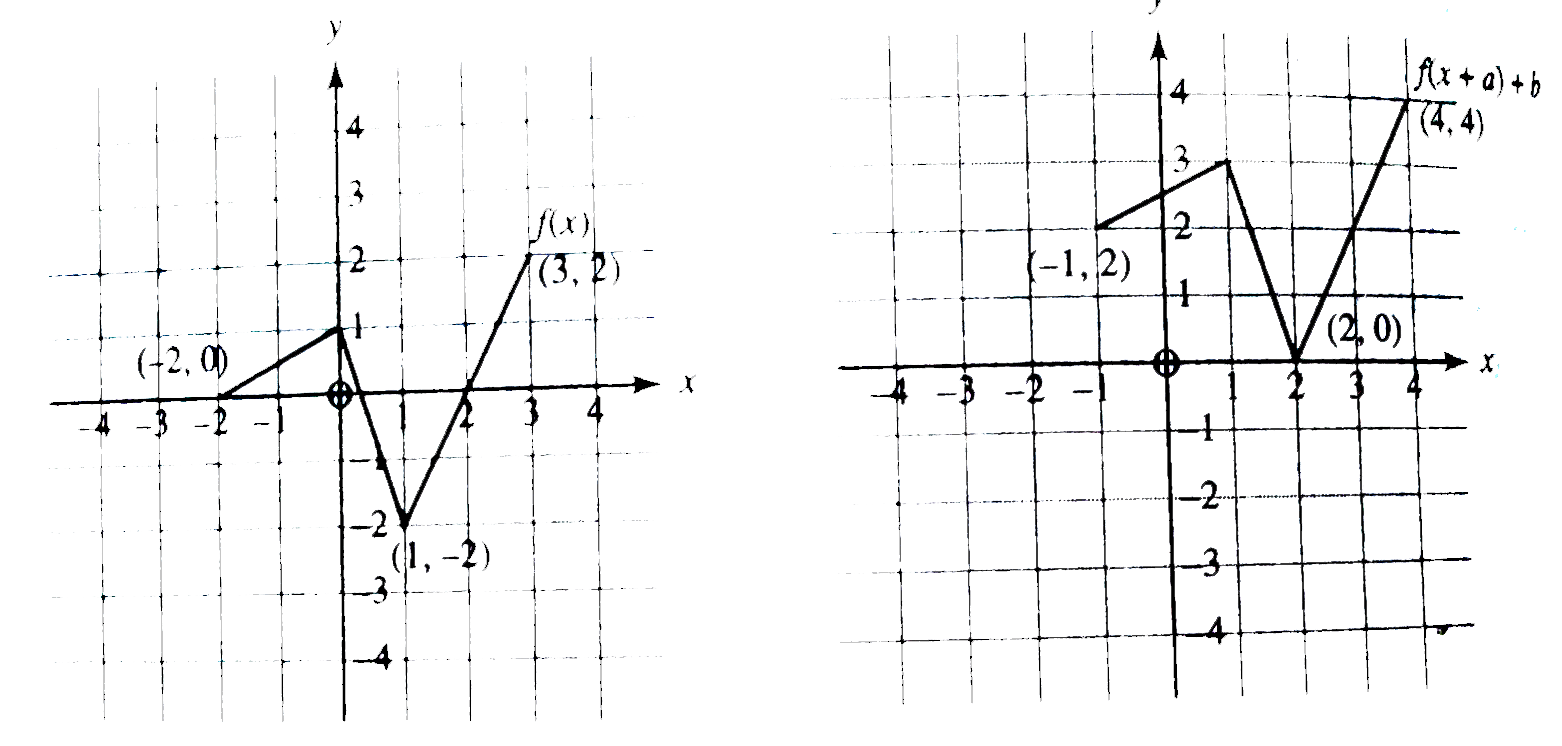
The graphs shown above represent f(x) and f(x+a) + b, where a and b are constants. Which of the following is the ordered pair (a,b) ?A(1,2)B(2,1)C(-1,2)D(2,-1)
Let f(x)=a+b|x|+c|x|4 , where a,bandc are real constants. Then, f(x) is differentiable at x=0, if a=0 (b) b=0 (c) c=0 (d) none of these
View SolutionThe potential in the electric field varies as V=−ax2+b with respect to x - coordinate, where a and b are constants. Find the charge density ρ(x) in a space.
View SolutionForm the differential equation of y=a(x+b), where a,b are constants.
View SolutionLet f(x)=a+b|x|+c|x|2, where a,b,c are real constants. The, f'(0) exists if
View SolutionSuppose f(x) is a function satisfying the following conditions: f(0)=2,f(1)=1 f has a minimum value at x=52 For all x,f′(x)=|2ax2ax−12ax+b+1+1−12(ax+b)2ax+2b+12ax+b| where a,b are some constants. Determine the constants a,b , and the function f(x)
View SolutionSuppose f(x) is a function satisfying the following conditions: f(0)=2,f(1)=1 f has a minimum value at x=52 For all x,f′(x)=|2ax2ax−12ax+b+1+1−12(ax+b)2ax+2b+12ax+b| where a,b are some constants. Determine the constants a,b , and the function f(x)
View SolutionIf f(a−x)=f(a+x) and f(b−x)=f(b+x) for all real x, where a,b(a>b>0) are constants, then prove that f(x) is a periodic function.
View Solution
