ABCD is parallelogram. If the coordinates of A,B,C are (−2,−1),(3,0) and (1,−2) respectively, find the coordinates of D.
To find the coordinates of point D in the parallelogram ABCD, we can use the properties of vectors. Here are the steps to solve the problem:
Step 1: Identify the coordinates of points A, B, and C
We are given:
- A = (-2, -1)
- B = (3, 0)
- C = (1, -2)
Step 2: Represent the points as position vectors
We can express the coordinates of points A, B, C, and D as position vectors:
- Position vector of A, →A=−2^i−1^j
- Position vector of B, →B=3^i+0^j
- Position vector of C, →C=1^i−2^j
- Position vector of D, →D=x^i+y^j
Step 3: Use the property of parallelograms
In a parallelogram, the vector →AB is equal to the vector →DC. Therefore, we can write:
→AB=→B−→A=→DC=→C−→D
Step 4: Calculate →AB
Calculating →AB:
→AB=→B−→A=(3^i+0^j)−(−2^i−1^j)=(3+2)^i+(0+1)^j=5^i+1^j
Step 5: Set up the equation for →DC
Now, we can express →DC:
→DC=→C−→D=(1^i−2^j)−(x^i+y^j)=(1−x)^i+(−2−y)^j
Step 6: Set the vectors equal
Since →AB=→DC, we have:
5^i+1^j=(1−x)^i+(−2−y)^j
Step 7: Compare coefficients
Now, we can compare the coefficients of ^i and ^j:
1. For ^i:
5=1−x⟹x=1−5=−4
2. For ^j:
1=−2−y⟹y=−2−1=−3
Step 8: Write the coordinates of D
Thus, the coordinates of point D are:
D=(x,y)=(−4,−3)
Final Answer
The coordinates of point D are D(−4,−3).
---
We are given:
- A = (-2, -1)
- B = (3, 0)
- C = (1, -2)
We can express the coordinates of points A, B, C, and D as position vectors:
- Position vector of A, →A=−2^i−1^j
- Position vector of B, →B=3^i+0^j
- Position vector of C, →C=1^i−2^j
- Position vector of D, →D=x^i+y^j
In a parallelogram, the vector →AB is equal to the vector →DC. Therefore, we can write:
→AB=→B−→A=→DC=→C−→D
Calculating →AB:
→AB=→B−→A=(3^i+0^j)−(−2^i−1^j)=(3+2)^i+(0+1)^j=5^i+1^j
Now, we can express →DC:
→DC=→C−→D=(1^i−2^j)−(x^i+y^j)=(1−x)^i+(−2−y)^j
Since →AB=→DC, we have:
5^i+1^j=(1−x)^i+(−2−y)^j
Now, we can compare the coefficients of ^i and ^j:
1. For ^i:
5=1−x⟹x=1−5=−4
2. For ^j:
1=−2−y⟹y=−2−1=−3
Thus, the coordinates of point D are:
D=(x,y)=(−4,−3)
The coordinates of point D are D(−4,−3).
Topper's Solved these Questions
Similar Questions
ABCD is a parallelogram. If the coordinates of A, B, C are (2,3), (1,4)and (0,−2) respectively, find the coordinates of D.
View SolutionThe centroid of a triangle ABC is at the point (1,1,1). If the coordinates of A and B are (3,-5,7) and (-1,7,-6) respectively, find the coordinates of the point C.
View Solution
Knowledge Check
In the rectangular coordinate system shown, ABCD is a parallogram. If the coordinates of the points A,B,C and D are (0,2),(a,b),(a,2) and (0,0), respectively, then b =
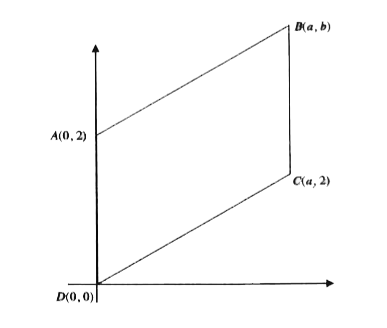 A0B2C3D4
A0B2C3D4
The centroid of a triangle ABC is at the point (1,1,1). If the coordinates of A and B are (3,5,7)and (1,7,6), respectively, find the coordinates of the point C.
View SolutionSuppose ABCD is a quadrilateral such that the coordinates of A, B and C are (1,3)(−2,6)and(5,−8) respectively. For what choices of coordinates of D will make ABCD a trapezium ?
View SolutionSuppose ABCD is a quadrilateral such that the coordinates of A, B and C are (1,3)(−2,6)and(5,−8) respectively. For what choices of coordinates of D will make ABCD a trapezium ?
View SolutionIf A and B are (1, 4) and (5, 2) respectively, find the coordinates of P when AP/BP=3/4 .
View SolutionThe three vertices of a parallelogram taken in order are (−1,0), (3,1) and (2,2) respectively. Find the coordinates of the fourth vertex.
View SolutionA ,B and C are the points (2,0),(5,0)and(5,3) respectively. Find coordinates of D such that ABCD is a square.
View SolutionThe coordinates of the mid points of sides AB, BC and CA of ABC are D(1,2,−3), E(3,0,1)and F(−1,1,−4) respectively. Write the coordinates of its centroid.
View Solution
