Subtract as indicated: 425−215
To solve the problem 425−215, we will follow these steps:
Step 1: Convert the mixed fractions to improper fractions.
A mixed fraction consists of a whole number and a proper fraction. To convert a mixed fraction to an improper fraction, we use the formula:
\(
\text{Improper Fraction} = \left(\text{Whole Number} \times \text{Denominator}\right) + \text{Numerator} \div \text{Denominator}
\)
For 425:
- Whole number = 4
- Numerator = 2
- Denominator = 5
Calculating:
\(
4 \times 5 + 2 = 20 + 2 = 22
\)
So, 425 becomes:
\(
\frac{22}{5}
\)
For 215:
- Whole number = 2
- Numerator = 1
- Denominator = 5
Calculating:
\(
2 \times 5 + 1 = 10 + 1 = 11
\)
So, 215 becomes:
\(
\frac{11}{5}
\)
Step 2: Subtract the improper fractions.
Now we have:
\(
\frac{22}{5} - \frac{11}{5}
\)
Since the denominators are the same, we can subtract the numerators directly:
\(
\frac{22 - 11}{5} = \frac{11}{5}
\)
Step 3: Write the final answer.
The result of the subtraction 425−215 is:
\(
\frac{11}{5}
\)
Summary:
So, the final answer is:
\(
\frac{11}{5}
\)
---
A mixed fraction consists of a whole number and a proper fraction. To convert a mixed fraction to an improper fraction, we use the formula:
\text{Improper Fraction} = \left(\text{Whole Number} \times \text{Denominator}\right) + \text{Numerator} \div \text{Denominator}
\)
- Whole number = 4
- Numerator = 2
- Denominator = 5
\(
4 \times 5 + 2 = 20 + 2 = 22
\)
So, 425 becomes:
\(
\frac{22}{5}
\)
- Whole number = 2
- Numerator = 1
- Denominator = 5
\(
2 \times 5 + 1 = 10 + 1 = 11
\)
So, 215 becomes:
\(
\frac{11}{5}
\)
Now we have:
\(
\frac{22}{5} - \frac{11}{5}
\)
\(
\frac{22 - 11}{5} = \frac{11}{5}
\)
The result of the subtraction 425−215 is:
\(
\frac{11}{5}
\)
So, the final answer is:
\(
\frac{11}{5}
\)
Topper's Solved these Questions
Similar Questions
Subtract as indicated: 434−216
View SolutionSubtract as indicated: 567−223
View SolutionSubtract as indicated: 83−59
View SolutionArrange the given ratios as indicated:
a. In ascending order:
In descending order:
i. 2:3, 3:5, 5:6, 2:9 ii. 1:4, 3:5, 5:6, 7:10
View SolutionSubtract using horizontal method :
2a+3b from 4a+5b
View SolutionSubtract: 415from 215
View SolutionSubtract 34 from 56.
View SolutionSubtract the sum of 23and12 from the sum of 56,712and415
View SolutionFind 425−215
View Solution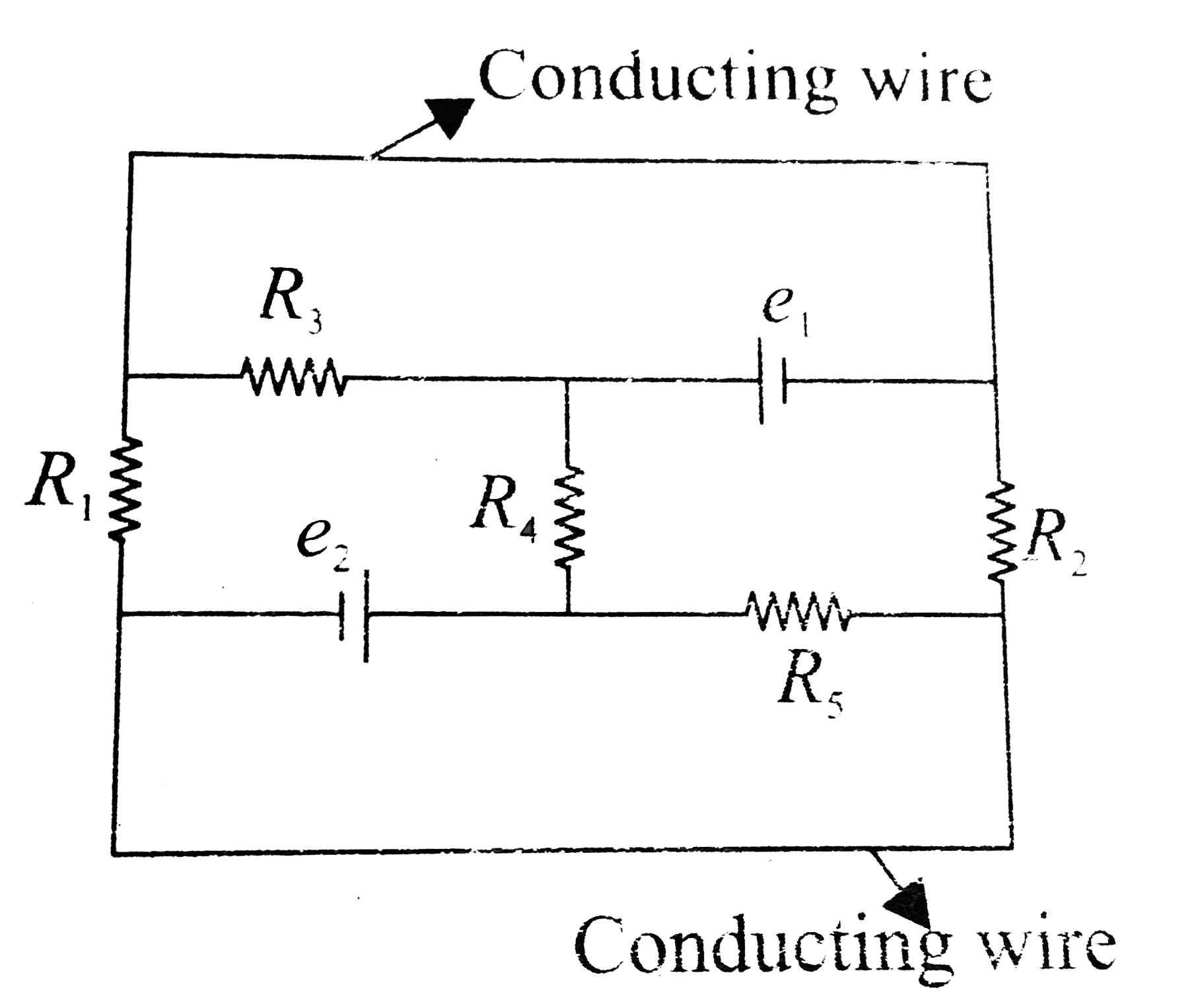
In the given cirucit, determine current through branch having indicated resistor R4 Given R1=R2=4Ω,R3=R4=2Ω
R5=5Ωε1=5V,ε2=10V
View Solution
