Fill in the blanks: English alphabet Letter, Line Symmetry, Number of Lines of symmetry, Rotational Symmetry, Order of rotational Symmetry Z S H O E N C, Nil - Yes Yes Yes - -, 0 - - - - - -, Yes - Yes Yes - Yes -, 2 - - - - - -
To fill in the blanks for the given question about the letters Z, S, H, O, E, N, and C regarding their symmetry properties, we will analyze each letter step by step.
1. Letter Z:
- Line Symmetry: No (there is no line that divides Z into two identical halves).
- Number of Lines of Symmetry: 0.
- Rotational Symmetry: Yes (it looks the same when rotated 180 degrees).
- Order of Rotational Symmetry: 2.
2. Letter S:
- Line Symmetry: No (it does not have a line that divides it into two identical halves).
- Number of Lines of Symmetry: 0.
- Rotational Symmetry: Yes (it looks the same when rotated 180 degrees).
- Order of Rotational Symmetry: 2.
3. Letter H:
- Line Symmetry: Yes (it can be divided vertically and horizontally into two identical halves).
- Number of Lines of Symmetry: 2 (one vertical and one horizontal).
- Rotational Symmetry: Yes (it looks the same when rotated 180 degrees).
- Order of Rotational Symmetry: 2.
4. Letter O:
- Line Symmetry: Yes (it can be divided in multiple ways to create identical halves).
- Number of Lines of Symmetry: Infinite (any line through the center creates symmetry).
- Rotational Symmetry: Yes (it looks the same when rotated by any angle).
- Order of Rotational Symmetry: Infinite.
5. Letter E:
- Line Symmetry: Yes (it can be divided vertically into two identical halves).
- Number of Lines of Symmetry: 1 (only one vertical line).
- Rotational Symmetry: No (it does not look the same when rotated).
- Order of Rotational Symmetry: 0.
6. Letter N:
- Line Symmetry: No (it does not have a line that divides it into two identical halves).
- Number of Lines of Symmetry: 0.
- Rotational Symmetry: Yes (it looks the same when rotated 180 degrees).
- Order of Rotational Symmetry: 2.
7. Letter C:
- Line Symmetry: Yes (it can be divided vertically).
- Number of Lines of Symmetry: 1 (only one vertical line).
- Rotational Symmetry: No (it does not look the same when rotated).
- Order of Rotational Symmetry: 0.
Final Table:
| Letter | Line Symmetry | Number of Lines of Symmetry | Rotational Symmetry | Order of Rotational Symmetry |
|--------|---------------|-----------------------------|---------------------|------------------------------|
| Z | No | 0 | Yes | 2 |
| S | No | 0 | Yes | 2 |
| H | Yes | 2 | Yes | 2 |
| O | Yes | Infinite | Yes | Infinite |
| E | Yes | 1 | No | 0 |
| N | No | 0 | Yes | 2 |
| C | Yes | 1 | No | 0 |
- Line Symmetry: No (there is no line that divides Z into two identical halves).
- Number of Lines of Symmetry: 0.
- Rotational Symmetry: Yes (it looks the same when rotated 180 degrees).
- Order of Rotational Symmetry: 2.
- Line Symmetry: No (it does not have a line that divides it into two identical halves).
- Number of Lines of Symmetry: 0.
- Rotational Symmetry: Yes (it looks the same when rotated 180 degrees).
- Order of Rotational Symmetry: 2.
- Line Symmetry: Yes (it can be divided vertically and horizontally into two identical halves).
- Number of Lines of Symmetry: 2 (one vertical and one horizontal).
- Rotational Symmetry: Yes (it looks the same when rotated 180 degrees).
- Order of Rotational Symmetry: 2.
- Line Symmetry: Yes (it can be divided in multiple ways to create identical halves).
- Number of Lines of Symmetry: Infinite (any line through the center creates symmetry).
- Rotational Symmetry: Yes (it looks the same when rotated by any angle).
- Order of Rotational Symmetry: Infinite.
- Line Symmetry: Yes (it can be divided vertically into two identical halves).
- Number of Lines of Symmetry: 1 (only one vertical line).
- Rotational Symmetry: No (it does not look the same when rotated).
- Order of Rotational Symmetry: 0.
- Line Symmetry: No (it does not have a line that divides it into two identical halves).
- Number of Lines of Symmetry: 0.
- Rotational Symmetry: Yes (it looks the same when rotated 180 degrees).
- Order of Rotational Symmetry: 2.
- Line Symmetry: Yes (it can be divided vertically).
- Number of Lines of Symmetry: 1 (only one vertical line).
- Rotational Symmetry: No (it does not look the same when rotated).
- Order of Rotational Symmetry: 0.
|--------|---------------|-----------------------------|---------------------|------------------------------|
| Z | No | 0 | Yes | 2 |
| S | No | 0 | Yes | 2 |
| H | Yes | 2 | Yes | 2 |
| O | Yes | Infinite | Yes | Infinite |
| E | Yes | 1 | No | 0 |
| N | No | 0 | Yes | 2 |
| C | Yes | 1 | No | 0 |
Topper's Solved these Questions
Similar Questions
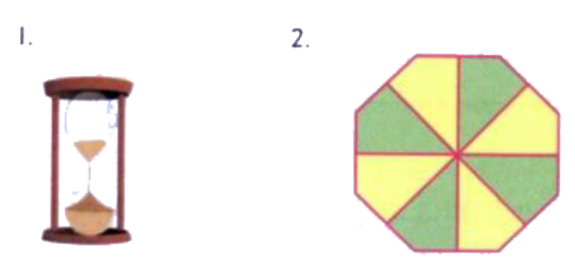
Write the number of lines of symmetry, the order of rotational symmetry, and the angle of rotational symmetry for the given figures.
View SolutionWrite three capital letters of the English alphabet that have line symmetry but not rotational symmetry?
View SolutionGive an example of a letter of the English alphabet which has (i) No line of symmetry (ii) Rotational symmetry of order 2.
View SolutionList all letters of the English alphabet that have:
a. one line of symmetry.
b. two lines of symmetry.
c. no line of symmetry.
View SolutionWhich letters of the English alphabet have :
no lines of symmetry
View SolutionWrite three capital letters of the English alphabet that do not have line symmetry but have rotational symmetry?
View SolutionWhich letters of the English alphabet have :
a single horizontal line of symmetry
View SolutionIf a figure has two or more lines of symmetry, should it have rotational symmetry of order more than 1?
View SolutionWhich letters of the English alphabet have :
a single vertical line of symmetry
View SolutionWhich letters of the English alphabet have :
one horizontal and one vertical line of symmetry
View Solution
