The runs scored in a cricket match by 11 players are as follows: Find the mean, mode and median of this data.
To find the mean, mode, and median of the runs scored by 11 players in a cricket match, we will follow these steps:
Step 1: Arrange the Data in Ascending Order
First, we need to arrange the runs scored by the players in ascending order. Let's assume the runs scored are as follows:
- 6, 8, 10, 10, 10, 15, 15, 50, 80, 100, 120
Arranging these in ascending order:
- 6, 8, 10, 10, 10, 15, 15, 50, 80, 100, 120
Step 2: Find the Median
To find the median, we need to determine the middle value of the ordered data. Since there are 11 observations (an odd number), the median will be the value at the position given by the formula:
Median Position=n+12
Where n is the number of observations.
Calculating:
Median Position=11+12=122=6
Now, we find the 6th observation in our ordered list:
- 1st: 6
- 2nd: 8
- 3rd: 10
- 4th: 10
- 5th: 10
- 6th: 15
Thus, the median is 15.
Step 3: Find the Mean
To find the mean, we calculate the sum of all the observations and then divide by the number of observations.
Calculating the sum:
Sum=6+8+10+10+10+15+15+50+80+100+120=424
Now, divide the sum by the number of observations (11):
Mean=42411≈38.5454
Thus, the mean is approximately 38.55.
Step 4: Find the Mode
The mode is the value that appears most frequently in the data set. From our ordered data:
- 6 (1 time)
- 8 (1 time)
- 10 (3 times)
- 15 (2 times)
- 50 (1 time)
- 80 (1 time)
- 100 (1 time)
- 120 (1 time)
The number that appears the most is 10, which appears 3 times.
Summary of Results:
- Mean: 38.55
- Median: 15
- Mode: 10
---
First, we need to arrange the runs scored by the players in ascending order. Let's assume the runs scored are as follows:
- 6, 8, 10, 10, 10, 15, 15, 50, 80, 100, 120
- 6, 8, 10, 10, 10, 15, 15, 50, 80, 100, 120
To find the median, we need to determine the middle value of the ordered data. Since there are 11 observations (an odd number), the median will be the value at the position given by the formula:
Median Position=n+12
Where n is the number of observations.
Median Position=11+12=122=6
- 1st: 6
- 2nd: 8
- 3rd: 10
- 4th: 10
- 5th: 10
- 6th: 15
To find the mean, we calculate the sum of all the observations and then divide by the number of observations.
Sum=6+8+10+10+10+15+15+50+80+100+120=424
Mean=42411≈38.5454
The mode is the value that appears most frequently in the data set. From our ordered data:
- 6 (1 time)
- 8 (1 time)
- 10 (3 times)
- 15 (2 times)
- 50 (1 time)
- 80 (1 time)
- 100 (1 time)
- 120 (1 time)
- Mean: 38.55
- Median: 15
- Mode: 10
Topper's Solved these Questions
Similar Questions
Find the mean, median and mode of the following data
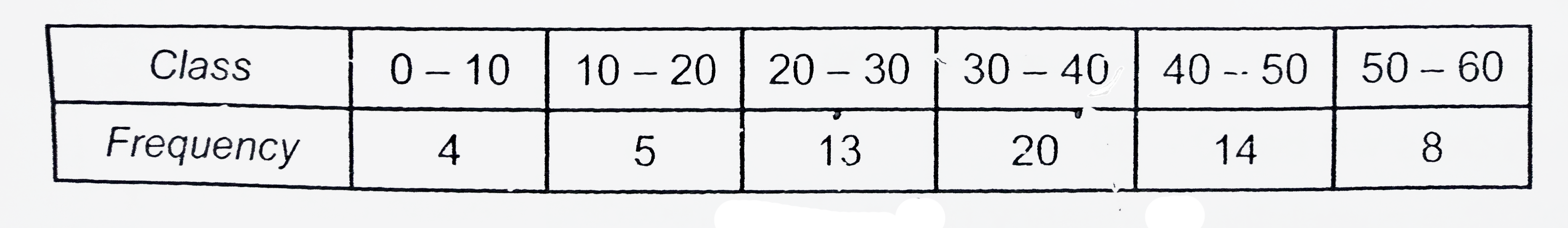
View SolutionFind the mean, median and mode of the following data :
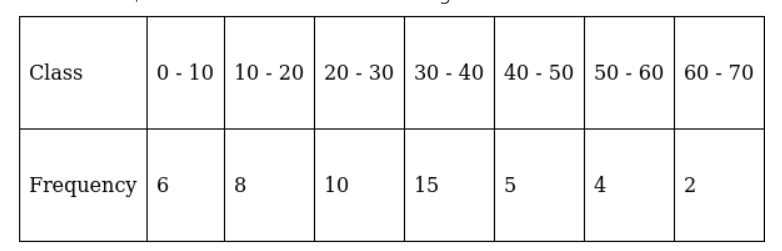
View SolutionThe scores in mathematics test (out of 25) of 15 students are as follows: Find the mode and median of this data. Are they same?
View SolutionFind the median and mode of the data:
View SolutionFind mean, median and mode of the following data :
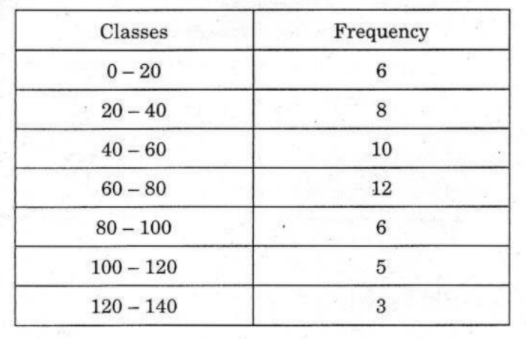
View SolutionThe runs scored in a cricket match by 11 players is as follows: 6, 15, 120, 50, 100, 80, 10, 15, 8, 10, 15. Find the mean, mode and median of this data. Are the three same?
View SolutionThe runs scored in a cricket match by 11 players is as follows:6, 15, 120, 50, 100, 80, 10, 15, 8, 10, 15Find the mean, mode and median of this data. Are the three same?
View SolutionFind the mean deviation about median for the following data:

View SolutionFind the mean deviation using median for the following datas:

View SolutionFind the median of the following data.
View Solution
