The mean of and is Then the median is 19 (b) 43 30 (d) None of these
To solve the problem step by step, we need to find the median of the data set given that the mean is equal to x.
Step 1: Understand the Data Set
The data set consists of the numbers: 10, 15, 19, 30, 43, 69, and x. We know that the mean of these numbers is x.
Step 2: Write the Mean Formula
The mean is calculated using the formula:
Mean=Sum of observationsNumber of observations
In this case, the mean is given as x.
Step 3: Calculate the Sum of Observations
The sum of the observations is:
10+15+19+30+43+69+x
Calculating the sum of the known numbers:
10+15+19+30+43+69=186
Thus, the sum of all observations can be written as:
186+x
Step 4: Set Up the Equation
Since the mean is given as x and there are 7 observations (including x), we can set up the equation:
x=186+x7
Step 5: Solve for x
To eliminate the fraction, multiply both sides by 7:
7x=186+x
Now, isolate x by subtracting x from both sides:
7x−x=186
This simplifies to:
6x=186
Now, divide both sides by 6:
x=1866=31
Step 6: Update the Data Set
Now that we have the value of x, we can update our data set:
10,15,19,30,31,43,69
Step 7: Arrange the Data in Ascending Order
The data is already in ascending order:
10,15,19,30,31,43,69
Step 8: Find the Median
To find the median, we use the formula for the median when the number of observations n is odd:
Median=n+12th observation
Here, n=7:
Median=7+12=82=4th observation
The 4th observation in the ordered data set is:
30
Conclusion
Thus, the median of the data set is:
Median=30
Options Check
Among the given options:
(a) 19, (b) 43, (c) 30, (d) None of these
The correct answer is (c) 30.
---
The data set consists of the numbers: 10, 15, 19, 30, 43, 69, and x. We know that the mean of these numbers is x.
The mean is calculated using the formula:
Mean=Sum of observationsNumber of observations
In this case, the mean is given as x.
The sum of the observations is:
10+15+19+30+43+69+x
Calculating the sum of the known numbers:
10+15+19+30+43+69=186
Thus, the sum of all observations can be written as:
186+x
Since the mean is given as x and there are 7 observations (including x), we can set up the equation:
x=186+x7
To eliminate the fraction, multiply both sides by 7:
7x=186+x
Now, isolate x by subtracting x from both sides:
7x−x=186
This simplifies to:
6x=186
Now, divide both sides by 6:
x=1866=31
Now that we have the value of x, we can update our data set:
10,15,19,30,31,43,69
The data is already in ascending order:
10,15,19,30,31,43,69
To find the median, we use the formula for the median when the number of observations n is odd:
Median=n+12th observation
Here, n=7:
Median=7+12=82=4th observation
The 4th observation in the ordered data set is:
30
Thus, the median of the data set is:
Median=30
Among the given options:
(a) 19, (b) 43, (c) 30, (d) None of these
The correct answer is (c) 30.
Topper's Solved these Questions
Similar Questions
The median of the data is 7 (b) 9 11 (d) 10
View SolutionIf the mean of observations is then 43 (b) 42 44 (d) 45
View SolutionIf the mean of 9, 10, 15, x, 6, 8 and 12 is 11 and is 11. The median of the observations is
View SolutionThe median of the data is 10 (b) 11 9 (d) None of these
View SolutionIf the mean of 26 , 19 , 15 , 24 , and x is x , then find the median of the data .
View SolutionFind the mean deviation using median:
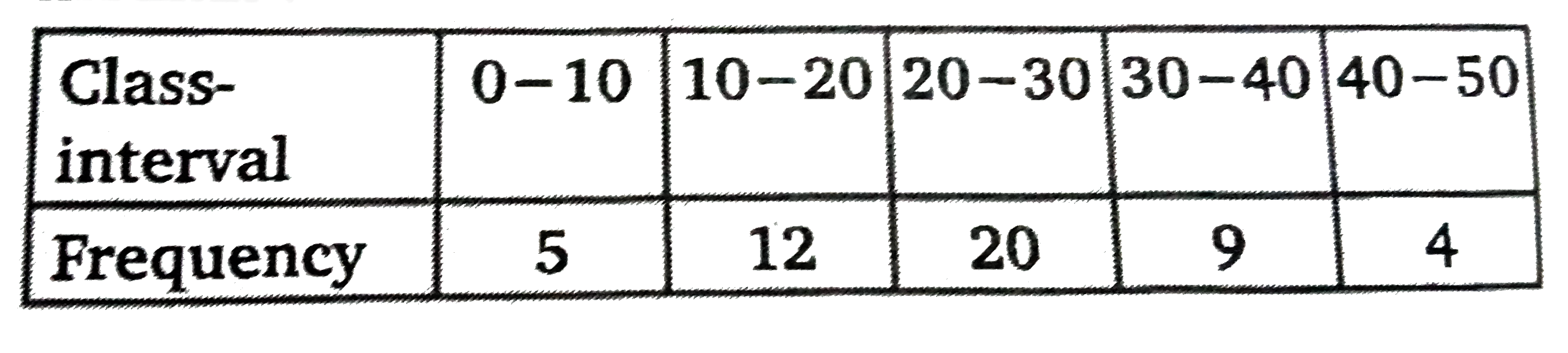
View SolutionThe median of 5,19,14,6,8,9,12,13,21 is
View SolutionIf mode of a series exceeds its mean by 12, then mode exceeds the median by (a) 4 (b) 8 (c) 6 (d) 10
View SolutionThe arithmetic mean and mode of a data are 24 and 12 respectively, then its median is (a) 25 (b) 18 (c) 20 (d) 22
View SolutionFor a normal distribution if the mean is M, mode is M0 and median is Md, then
View Solution
