[ABC is a right-angled triangle and O is the mid point of the side opposite to the right angle.Explain why O is equidistant from A ,B and C .(The dotted lines are drawn additionally to help you).]
To solve the problem, we need to show that point O, the midpoint of side BC in right-angled triangle ABC, is equidistant from points A, B, and C. Here’s a step-by-step solution:
Step 1: Identify the Right-Angled Triangle
Let triangle ABC be a right-angled triangle with the right angle at point A. The sides opposite to the right angle are BC.
Step 2: Locate the Midpoint O
Point O is defined as the midpoint of side BC. This means that O divides BC into two equal segments, BO and OC.
Step 3: Draw Parallel Lines
To demonstrate the equidistance, draw a line segment AD parallel to BC, where D is a point on the line extended from BC. This creates a parallelogram ABCD.
Step 4: Establish Properties of the Parallelogram
Since AD is parallel to BC and AB is parallel to CD, we have a parallelogram ABCD. In a parallelogram, opposite sides are equal and the angles are supplementary.
Step 5: Identify Right Angles
Since triangle ABC is right-angled at A, angle A is 90 degrees. Consequently, angle D must also be 90 degrees because consecutive angles in a parallelogram sum up to 180 degrees.
Step 6: Conclude that ABCD is a Rectangle
Since both pairs of opposite angles are right angles, parallelogram ABCD is a rectangle.
Step 7: Use Properties of Rectangles
In a rectangle, the diagonals are equal and bisect each other. Therefore, we have:
- AC = BD (diagonals are equal)
- AO = OC (since O is the midpoint of BC)
- BO = OD (since O is the midpoint of BD)
Step 8: Show Equidistance
From the properties established:
- AO = OC
- BO = OD
- Since diagonals bisect each other, we can conclude that AO = BO = OC = OD.
Thus, O is equidistant from points A, B, and C.
Final Conclusion
Therefore, point O is equidistant from points A, B, and C, as required.
---
Let triangle ABC be a right-angled triangle with the right angle at point A. The sides opposite to the right angle are BC.
Point O is defined as the midpoint of side BC. This means that O divides BC into two equal segments, BO and OC.
To demonstrate the equidistance, draw a line segment AD parallel to BC, where D is a point on the line extended from BC. This creates a parallelogram ABCD.
Since AD is parallel to BC and AB is parallel to CD, we have a parallelogram ABCD. In a parallelogram, opposite sides are equal and the angles are supplementary.
Since triangle ABC is right-angled at A, angle A is 90 degrees. Consequently, angle D must also be 90 degrees because consecutive angles in a parallelogram sum up to 180 degrees.
Since both pairs of opposite angles are right angles, parallelogram ABCD is a rectangle.
In a rectangle, the diagonals are equal and bisect each other. Therefore, we have:
- AC = BD (diagonals are equal)
- AO = OC (since O is the midpoint of BC)
- BO = OD (since O is the midpoint of BD)
From the properties established:
- AO = OC
- BO = OD
- Since diagonals bisect each other, we can conclude that AO = BO = OC = OD.
Therefore, point O is equidistant from points A, B, and C, as required.
Topper's Solved these Questions
Similar Questions
Prove that the mid-point of the hypotenuse of a right triangle is equidistant from its vertices.
View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
View SolutionProve that the mid-point of the hypotenuse of right angled triangle is equidistant from its vertices.
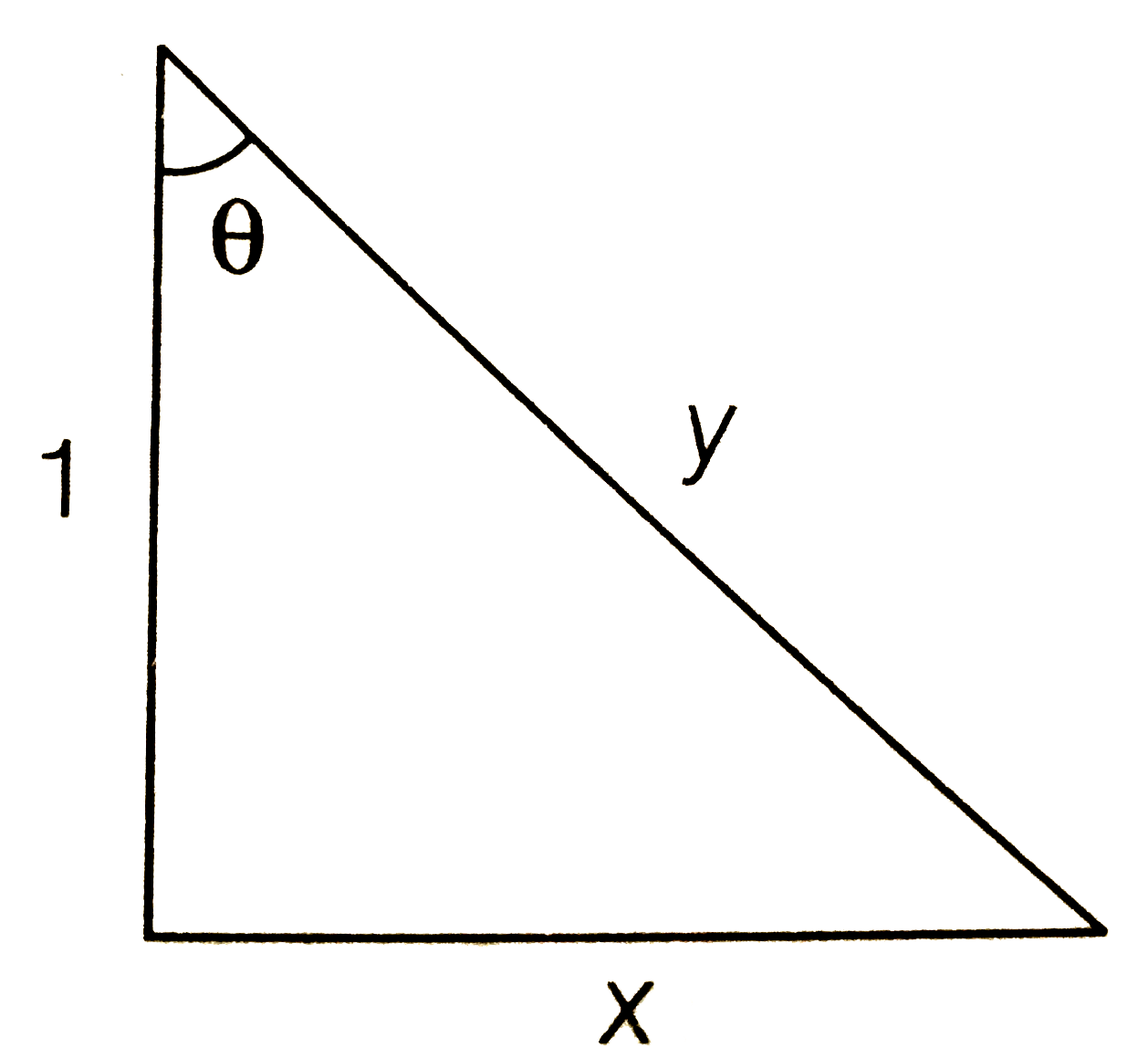
View SolutionA right angled triangle has legs 1 and x. The hypotenuse is y and angle opposite to the side x is θ. Shown as

View SolutionABC is a right angled triangle of given area S.Find the sides of the triangle for which the area of circumscribed circle is least.
View SolutionIn Fig. 14.40, a right triangle BOA is given. C is the mid-point of the hypotenuse AB . Show that it is equidistant from the vertices O, A and B . (FIGURE)
View SolutionIn a right -angle triangle, the length of the sides containing the right angles are aandb. With the mid-point of each sides as centres three semicircles are drawn outiside the triangle. Find the area of Δ and the semicircles together
View SolutionABC is a triangle right angled at B;and P is the mid-point of AC . Prove that: PQ ⊥AB (ii) Q is the mid point of AB PB=PA=12AC
View SolutionABC is right -angled triangle of given area S. Find the sides of the triangle for which the area of the circumscribed circle is least.
View Solution
