A rectangular strip 25cm x 7cm is rotated about the longer side. Find the total surface area of the solid thus generated.
To find the total surface area of the solid generated by rotating a rectangular strip of dimensions 25 cm x 7 cm about its longer side, we can follow these steps:
1. Identify the Dimensions of the Rectangular Strip:
- Length (longer side) = 25 cm
- Breadth (shorter side) = 7 cm
2. Determine the Shape Formed by Rotation:
- When the rectangular strip is rotated about its longer side (25 cm), it forms a right circular cylinder.
3. Identify the Radius and Height of the Cylinder:
- The radius (r) of the cylinder is equal to the breadth of the rectangular strip, which is 7 cm.
- The height (h) of the cylinder is equal to the length of the rectangular strip, which is 25 cm.
4. Use the Formula for Total Surface Area of a Cylinder:
- The formula for the total surface area (TSA) of a cylinder is:
TSA=2πr(h+r)
- Here, r=7 cm and h=25 cm.
5. Substitute the Values into the Formula:
- Substitute r and h into the formula:
TSA=2×227×7×(25+7)
6. Calculate the Expression:
- First, calculate (25+7)=32.
- Now substitute back into the equation:
TSA=2×227×7×32
- The 7 in the numerator and denominator cancels out:
TSA=2×22×32
- Now calculate 2×22=44:
TSA=44×32
- Finally, calculate 44×32=1408.
7. Final Result:
- The total surface area of the solid generated is 1408cm2.
- Length (longer side) = 25 cm
- Breadth (shorter side) = 7 cm
- When the rectangular strip is rotated about its longer side (25 cm), it forms a right circular cylinder.
- The radius (r) of the cylinder is equal to the breadth of the rectangular strip, which is 7 cm.
- The height (h) of the cylinder is equal to the length of the rectangular strip, which is 25 cm.
- The formula for the total surface area (TSA) of a cylinder is:
TSA=2πr(h+r)
- Here, r=7 cm and h=25 cm.
- Substitute r and h into the formula:
TSA=2×227×7×(25+7)
- First, calculate (25+7)=32.
- Now substitute back into the equation:
TSA=2×227×7×32
- The 7 in the numerator and denominator cancels out:
TSA=2×22×32
- Now calculate 2×22=44:
TSA=44×32
- Finally, calculate 44×32=1408.
- The total surface area of the solid generated is 1408cm2.
Topper's Solved these Questions
Similar Questions
The sides containing right angle of a right angled triangle are 8 cm and 6 cm. It is rotated about the side of 8 cm. Find the total surface area of the formed solid.
View SolutionThe sides containing right angles of a right angled triangle are 8 cm and 6 cm it is rotated about its hypotenuse . Find the volume and total surface area of the solid formed.
View Solution
Knowledge Check
A rectangular park is 25 m long and 15 m wide. A path 3 m is constructed outside the park. Find the area of the path.
A277m2B276m2C266m2D256m2
A right angled triangle of which the sides containing the right angle are 6.3 cm and 10cm in length, is made to turn round on the longer side. Find the volume of the solid, thus generated. Also, find its curved surface area.
View SolutionThe sides containing right angles of a right angled triangle are 8 cm and 6 cm. It is rotated about its hypotenus. Find the volume and total surface area of the formed solid.
View SolutionA semi-circle of radius 17.5 cm is rotated about its diameter. Find the curved surface of so generated solid.
View SolutionFind the surface area of a cube whose edge is 11 cm
View SolutionA solid is in the form of a cylinder with hemispherical ends. Total height of the solid is 19 cm and the diameter of the cylinder is 7 cm. Find the volume and total surface area of the solid.
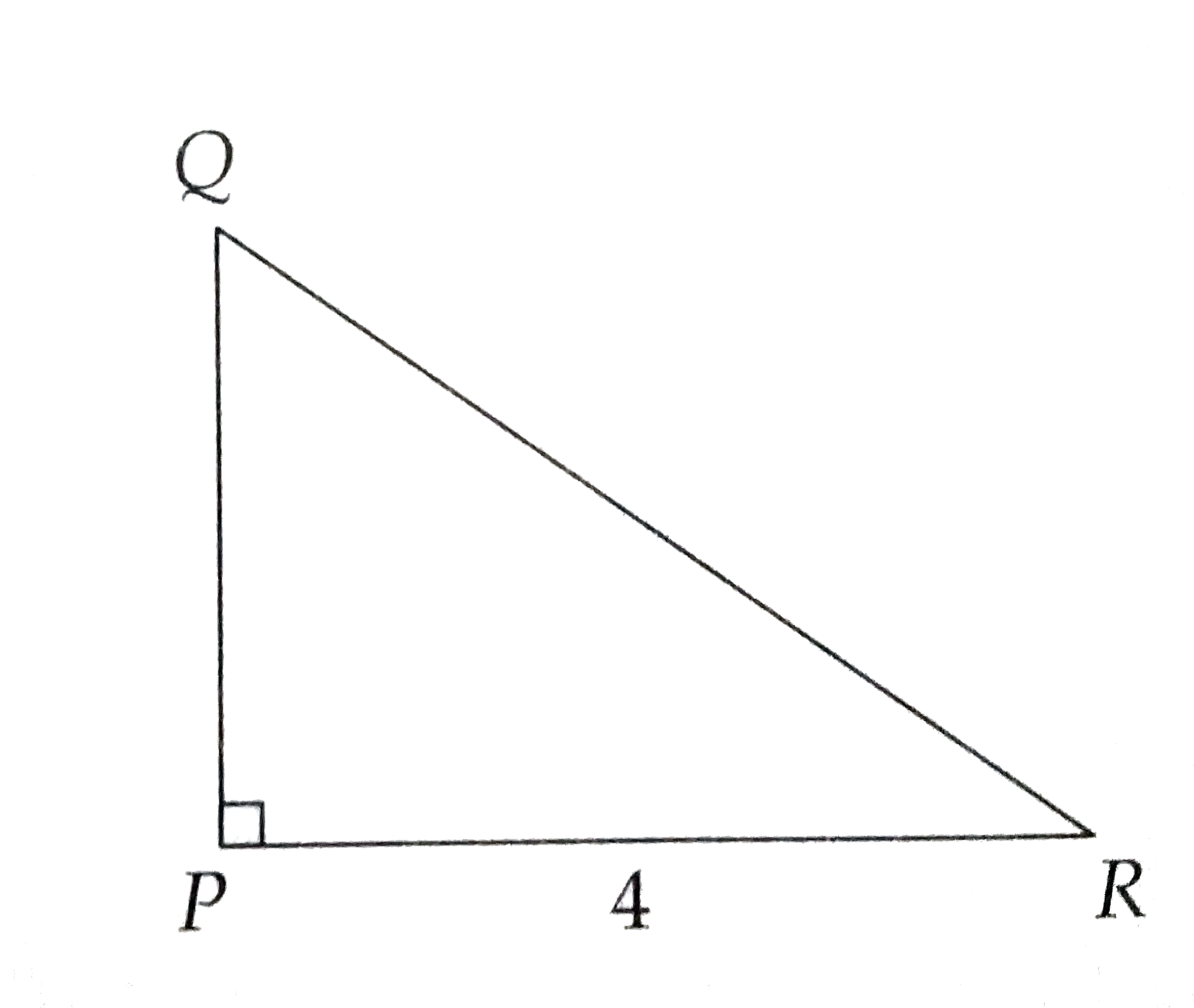
View SolutionThe measure of angle angle QPR is 90 degrees, and the area of triangle PQR is 6. If triangle PQR is rotated 360∘ about side PR, what is the total surface area of the resulting solid ?
View SolutionA solid is in the form of a cylinder with hemispherical ends. The total height of the solid is 19 cm and the diameter of the cylinder is 7 cm. Find the volume and total surface area of the solid (Use π=22/7 )
View Solution
