Figure shows a particle slideing on a frictionles track which terminates in straight horizontal section. If the particle starts slipping from the point. A, how far way from the track will the particle hit the ground ?
The correct Answer is:A, C, D
H=1m,h=0.5m
Applying law of conservation of Energy for point A and B
mgH=12mv2+mgh
→g−(12)v2=0.5g
→v2=2(g−0.5g)=g
→v=√g=3.1ms
After pointg B the body exhibits projectile motion for which
θ=0
y=−0.5
So, -0.5=4.9xxt^2<br>rarr t=0.31sec<br>So,x(v costheta)t<br>=3.1xx0.31=1m
So the practile will hit the ground at a horizontal distance m from B.
Topper's Solved these Questions
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Question for Short Answer
16 Videos
- WORK AND ENERGYBOOK - HC VERMA ENGLISHCHAPTER - WORK AND ENERGYEXERCISE - Objective -2
8 Videos
- WAVE MOTION AND WAVES ON A STRINGBOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer
7 Videos
Similar Questions
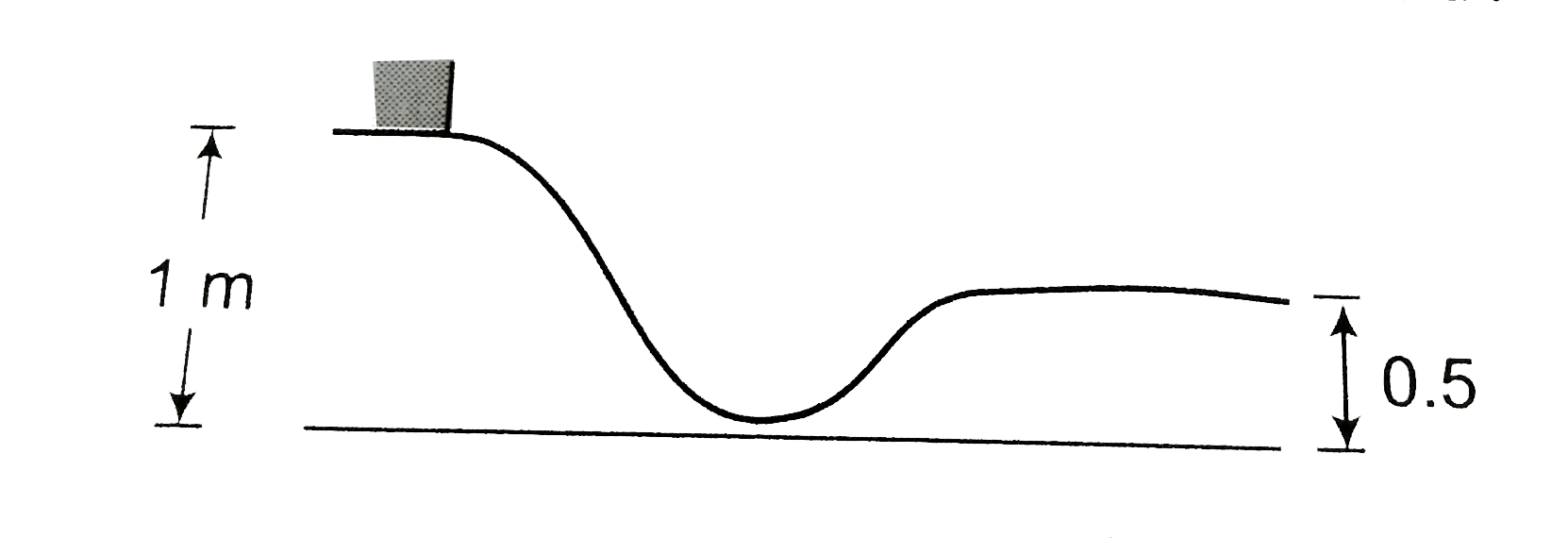
The figure shown a particle sliding on a frictionless track, which teminates in a straight horizontal section. If the particle starts slipping from the point A, how far away from the track will the particle hit the ground?
View Solution
The figure shown a particle sliding on a frictionless track, which teminates in a straight horizontal section. If the particle starts slipping from the point A, how far away from the track will the particle hit the ground?
View Solution
Knowledge Check

The figure shown a particle sliding on a frictionless track, which teminates in a straight horizontal section. If the particle starts slipping from the point A, how far away from the track will the particle hit the ground?A1mB2mC3mD4mThe velocity-time graph of a particle in straight line motion is veloity-time graph of a particle in straight line motion is shown in. The particle starts its motion from origin.
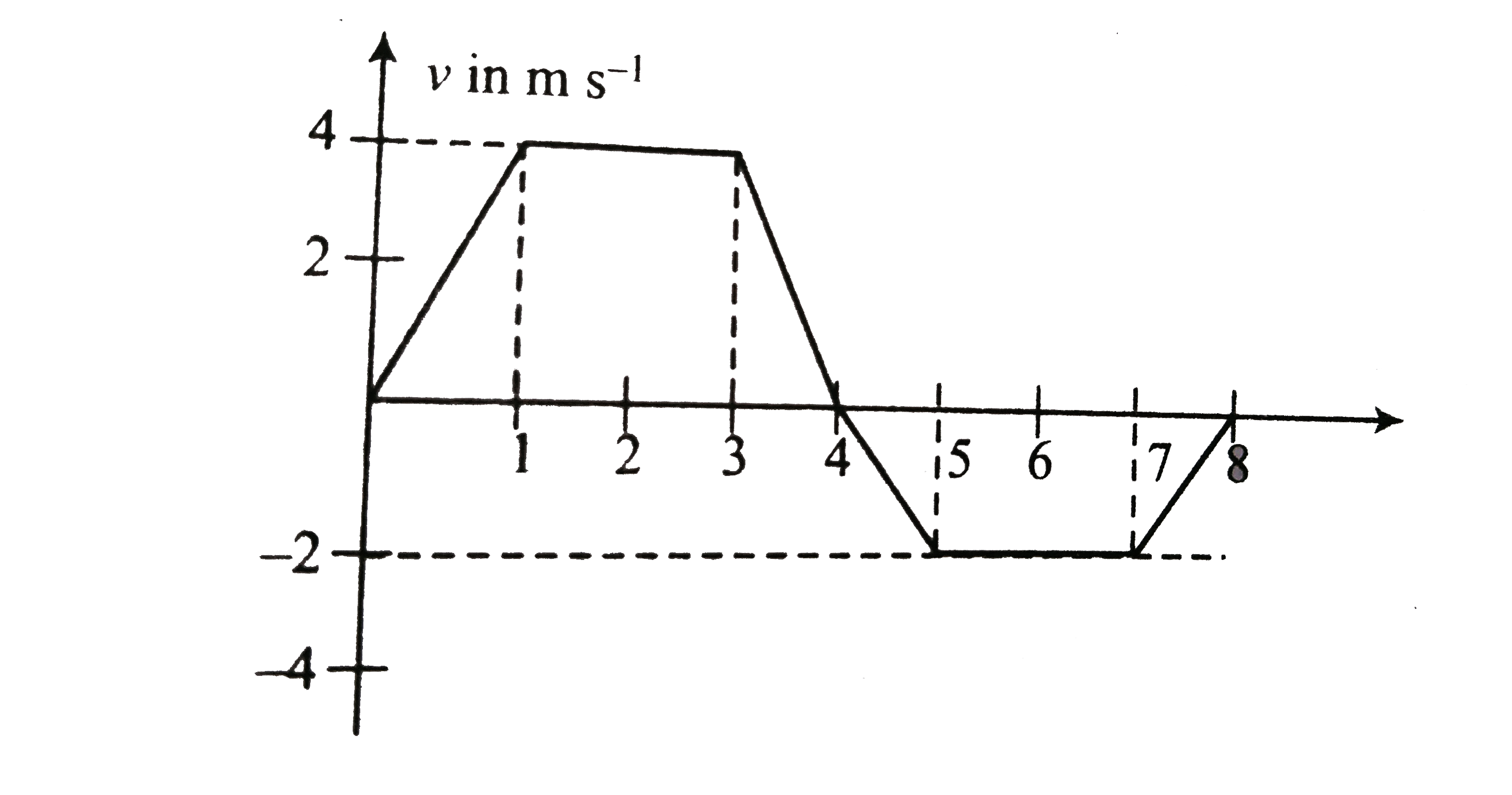 .
.
The distance of the particle from the origin after 8s is .A18mB16mC8mD6mA stone is just released from the window of a train moving along a horizontal straight track. The stone will hit the ground in
AStraight line pathBCircular pathCParabolic pathDHyperbolic path
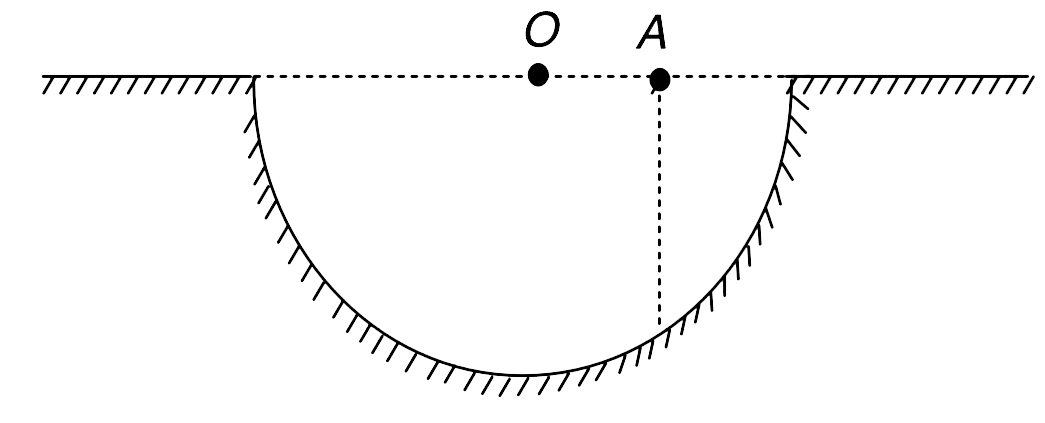
Figure shows a circular frictionless track of radius R, centred at point O. A particle of mass M is released from point A(OA=R/2). After collision with the track, the particle moves along the track.
(a) Find the coefficient of restitution e.
(b) What will be value of e if the velocity of the particle becomes horizontal just after collision ?
View SolutionThe velocity-time graph of a particle in straight line motion is velocity-time graph of a particle in straight line motion is shown in. The particle starts its motion from origin.
 .
.
The distance of the particle from the origin after 8s is .
View SolutionA stone is just released from the window of a train moving along a horizontal straight track. The stone will hit the ground in
View SolutionFigure shows a circular frications trck of radius R, centred at point O. A particle of mass M is released from point A(OA=R/2). After collision with the track, the particle moves along on the track. Then the coefficient of restitution e is.

View SolutionFigure shows a circular frications trck of radius R, centred at point O. A particle of mass M is released from point A(OA=R/2). After collision with the track, the particle moves along on the track. Then the coefficient of restitution e is.

View Solution
