A nearsighted man can clearly see objects up to a distance of 1.5m. Calculate the power of the lens of the spectacles necessary for the remedy of this defect.
To solve the problem of calculating the power of the lens necessary for a nearsighted man who can clearly see objects only up to a distance of 1.5 m, we will follow these steps:
Step 1: Understand the condition of the nearsighted man
A nearsighted person (myopic) can see objects clearly only up to a certain distance, which is given as 1.5 m in this case. Beyond this distance, objects appear blurry.
Step 2: Determine the required focal length of the lens
For a nearsighted person, we need to use a concave lens to correct their vision. The lens should create an image of distant objects (at infinity) at the near point of the person, which is 1.5 m.
Using the lens formula:
1f=1v−1u
Where:
- f = focal length of the lens
- v = image distance (for distant objects, we take it as infinity, so v=∞)
- u = object distance (the near point of the man, which is -1.5 m, negative because it is on the same side as the object)
Substituting the values:
1f=1∞−1−1.5
1f=0+23
f=32=1.5 m
Step 3: Calculate the power of the lens
The power P of a lens is given by the formula:
P=1f (in meters)
Since we have f=−1.5 m (the focal length is negative for a concave lens):
P=1−1.5=−23 diopters
P≈−0.67 diopters
Conclusion
The power of the lens required to correct the nearsightedness of the man is approximately -0.67 diopters.
---
A nearsighted person (myopic) can see objects clearly only up to a certain distance, which is given as 1.5 m in this case. Beyond this distance, objects appear blurry.
For a nearsighted person, we need to use a concave lens to correct their vision. The lens should create an image of distant objects (at infinity) at the near point of the person, which is 1.5 m.
1f=1v−1u
Where:
- f = focal length of the lens
- v = image distance (for distant objects, we take it as infinity, so v=∞)
- u = object distance (the near point of the man, which is -1.5 m, negative because it is on the same side as the object)
1f=1∞−1−1.5
1f=0+23
f=32=1.5 m
The power P of a lens is given by the formula:
P=1f (in meters)
Since we have f=−1.5 m (the focal length is negative for a concave lens):
P=1−1.5=−23 diopters
P≈−0.67 diopters
The power of the lens required to correct the nearsightedness of the man is approximately -0.67 diopters.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - OPTICAL INSTRUMENTSEXERCISE - Worked Out Examples10videos
OPTICAL INSTRUMENTS
- BOOK - HC VERMA ENGLISHCHAPTER - OPTICAL INSTRUMENTSEXERCISE - Objective -111videos
OPTICAL INSTRUMENTS
- BOOK - HC VERMA ENGLISHCHAPTER - MAGNETIC PROPERTIES OF MATTEREXERCISE - Short Answer6videos
MAGNETIC PROPERTIES OF MATTER
- BOOK - HC VERMA ENGLISHCHAPTER - PERMANENT MAGNETSEXERCISE - Short Answer11videos
PERMANENT MAGNETS
Similar Questions
The ciliary muscles of eye control the curvature of the lens in the eye and hence can alter the effective focal length of the system. When the muscles are fully relaxed, the focal length is maximum. When the muscles are strained, the curvature of lens increases. That means radius of curvature decreases and focal length decreases. For a clear vision, the image must be on the retina. The image distance is therefore fixed for clear vision and it equals the distance of retina from eye lens. It is about 2.5cm for a grown up person.
A perosn can theoretically have clear vision of an object situated at any large distance from the eye. The smallest distance at which a person can clearly see is related to minimum possible focal length. The ciliary muscles are most strained in this position. For an average grown up person, minimum distance of the object should be around 25cm.
A person suffering from eye defects uses spectacles (eye glass). The function of lens of spectacles is to form the image of the objects within the range in which the person can see clearly. The image o the spectacle lens becomes object for the eye lens and whose image is formed on the retina.
The number of spectacle lens used for th eremedy of eye defect is decided by the power fo the lens required and the number of spectacle lens is equal to the numerical value of the power of lens with sign. For example, if power of the lens required is +3D (converging lens of focal length 100/3cm ), then number of lens will be +3.
For all the calculations required, you can use the lens formula and lensmaker's formula. Assume that the eye lens is equiconvex lens. Neglect the distance between the eye lens and the spectacle lens.
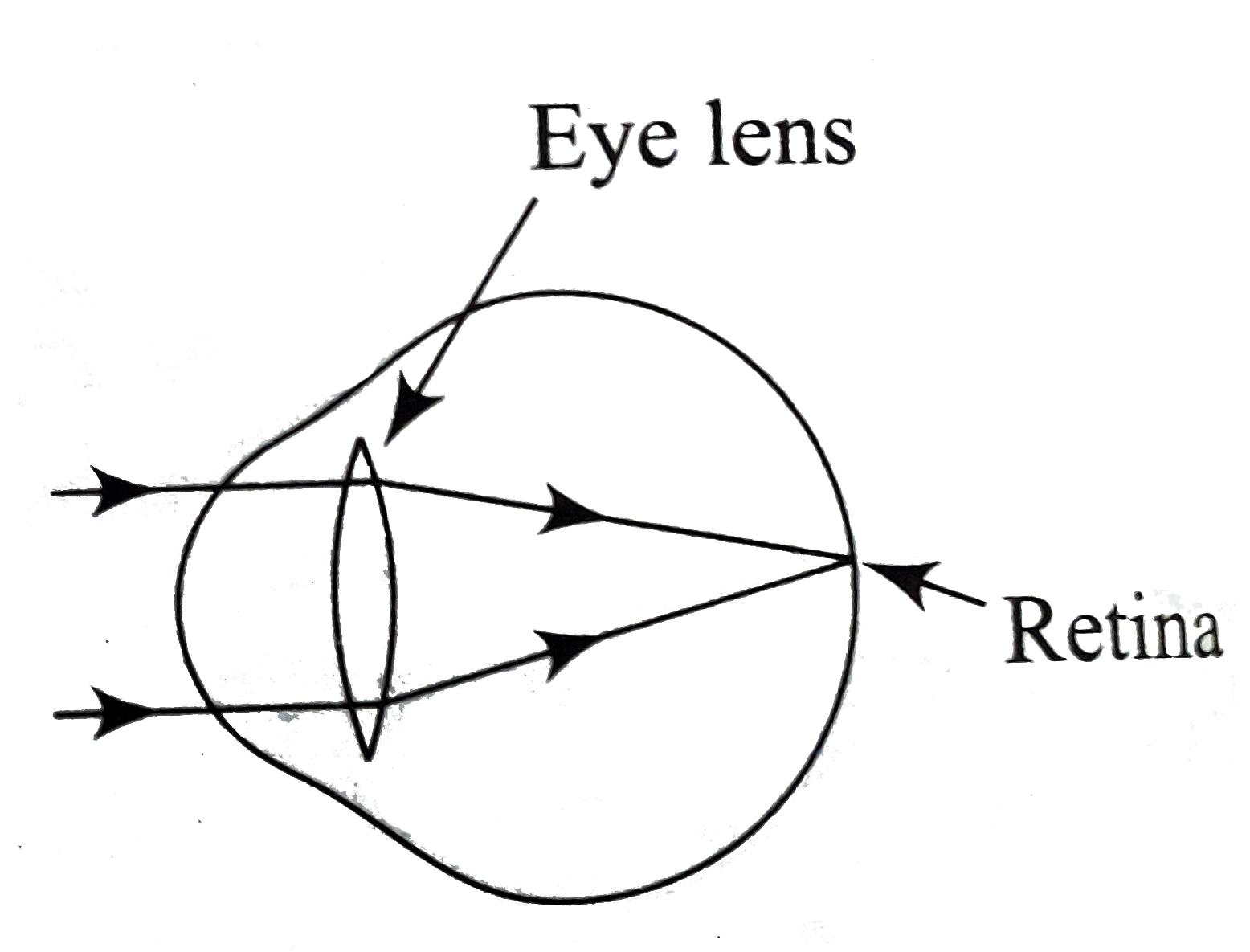
Q. A near sighted man can clearly see ojects only upto a distance of 100cm and not beyond this. The number of the spectacle lenses necessary for the remedy of this defect will beView SolutionA nearsighted person cannot clearly see beyond 200 cm. Find the power of the lens needed to see objects at large distances.
View SolutionIf a person can sees clearly at a distance of 100 cm, then find the power of leris used to see object at 40 cm.
View SolutionA person cannot see objects clearly beyond 125 cm. The power of the lens to correct the vision is
View SolutionThe ciliary muscles of eye control the curvature of the lens in the eye and hence can alter the effective focal length of the system. When the muscles are fully relaxed, the focal length is maximum. When the muscles are strained, the curvature of lens increases. That means radius of curvature decreases and focal length decreases. For a clear vision, the image must be on the retina. The image distance is therefore fixed for clear vision and it equals the distance of retina from eye lens. It is about 2.5cm for a grown up person.
A perosn can theoretically have clear vision of an object situated at any large distance from the eye. The smallest distance at which a person can clearly see is related to minimum possible focal length. The ciliary muscles are most strained in this position. For an average grown up person, minimum distance of the object should be around 25cm.
A person suffering from eye defects uses spectacles (eye glass). The function of lens of spectacles is to form the image of the objects within the range in which the person can see clearly. The image o the spectacle lens becomes object for the eye lens and whose image is formed on the retina.
The number of spectacle lens used for th eremedy of eye defect is decided by the power fo the lens required and the number of spectacle lens is equal to the numerical value of the power of lens with sign. For example, if power of the lens required is +3D (converging lens of focal length 100/3cm ), then number of lens will be +3.
For all the calculations required, you can use the lens formula and lensmaker's formula. Assume that the eye lens is equiconvex lens. Neglect the distance between the eye lens and the spectacle lens.
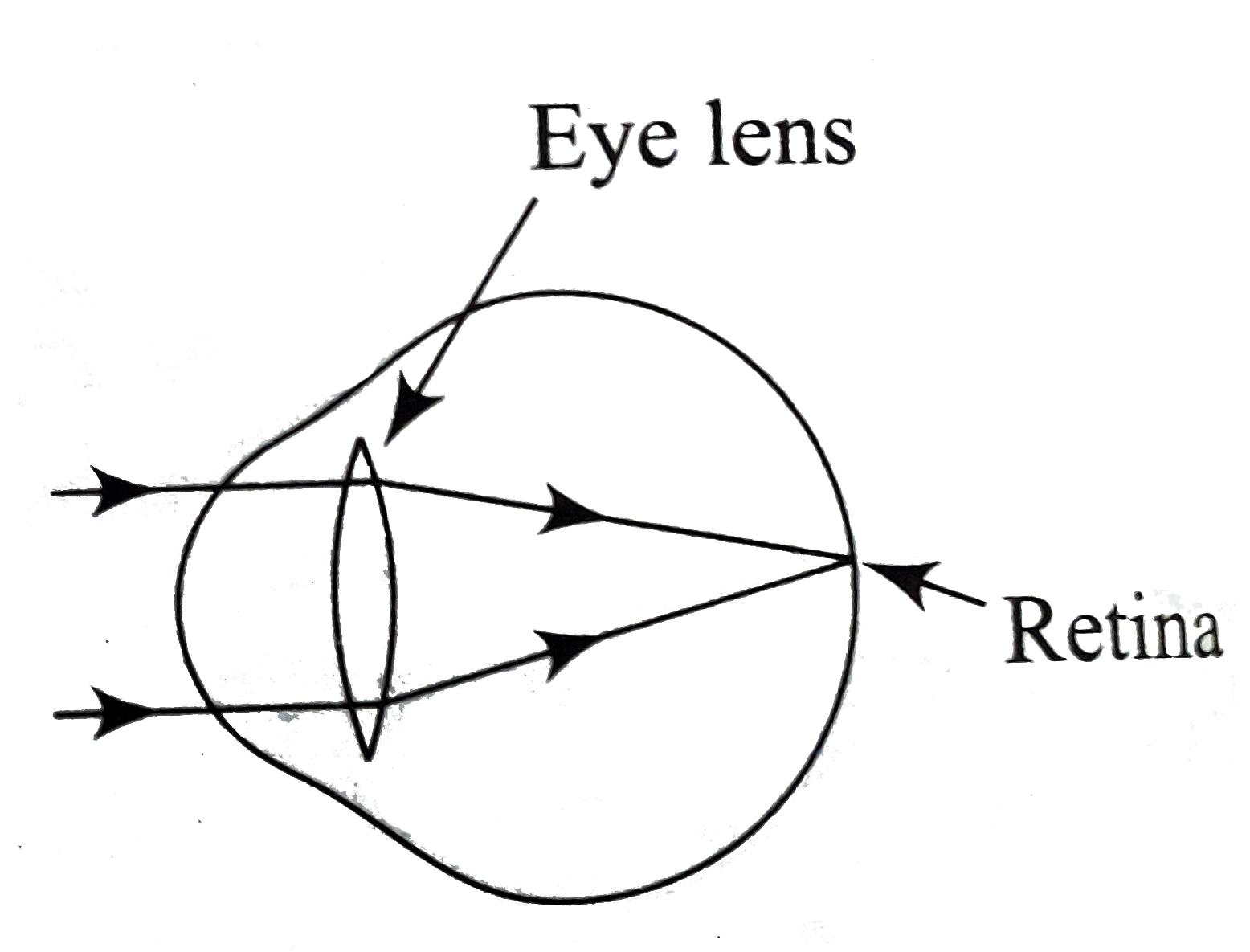
Q. A far sighted man connot see objects clearly unless they are at least 100cm from his eyes. The number of the spectacle lenses that will make his range of clear vision equal to an average grown up person will beView Solutiona man can see upto 100cm of the distant object. The power of the lens required to see far objects will be:
View SolutionThe ciliary muscles of eye control the curvature of the lens in the eye and hence can alter the effective focal length of the system. When the muscles are fully relaxed, the focal length is maximum. When the muscles are strained, the curvature of lens increases. That means radius of curvature decreases and focal length decreases. For a clear vision, the image must be on the retina. The image distance is therefore fixed for clear vision and it equals the distance of retina from eye lens. It is about 2.5cm for a grown up person.
A perosn can theoretically have clear vision of an object situated at any large distance from the eye. The smallest distance at which a person can clearly see is related to minimum possible focal length. The ciliary muscles are most strained in this position. For an average grown up person, minimum distance of the object should be around 25cm.
A person suffering from eye defects uses spectacles (eye glass). The function of lens of spectacles is to form the image of the objects within the range in which the person can see clearly. The image o the spectacle lens becomes object for the eye lens and whose image is formed on the retina.
The number of spectacle lens used for th eremedy of eye defect is decided by the power fo the lens required and the number of spectacle lens is equal to the numerical value of the power of lens with sign. For example, if power of the lens required is +3D (converging lens of focal length 100/3cm ), then number of lens will be +3.
For all the calculations required, you can use the lens formula and lensmaker's formula. Assume that the eye lens is equiconvex lens. Neglect the distance between the eye lens and the spectacle lens.
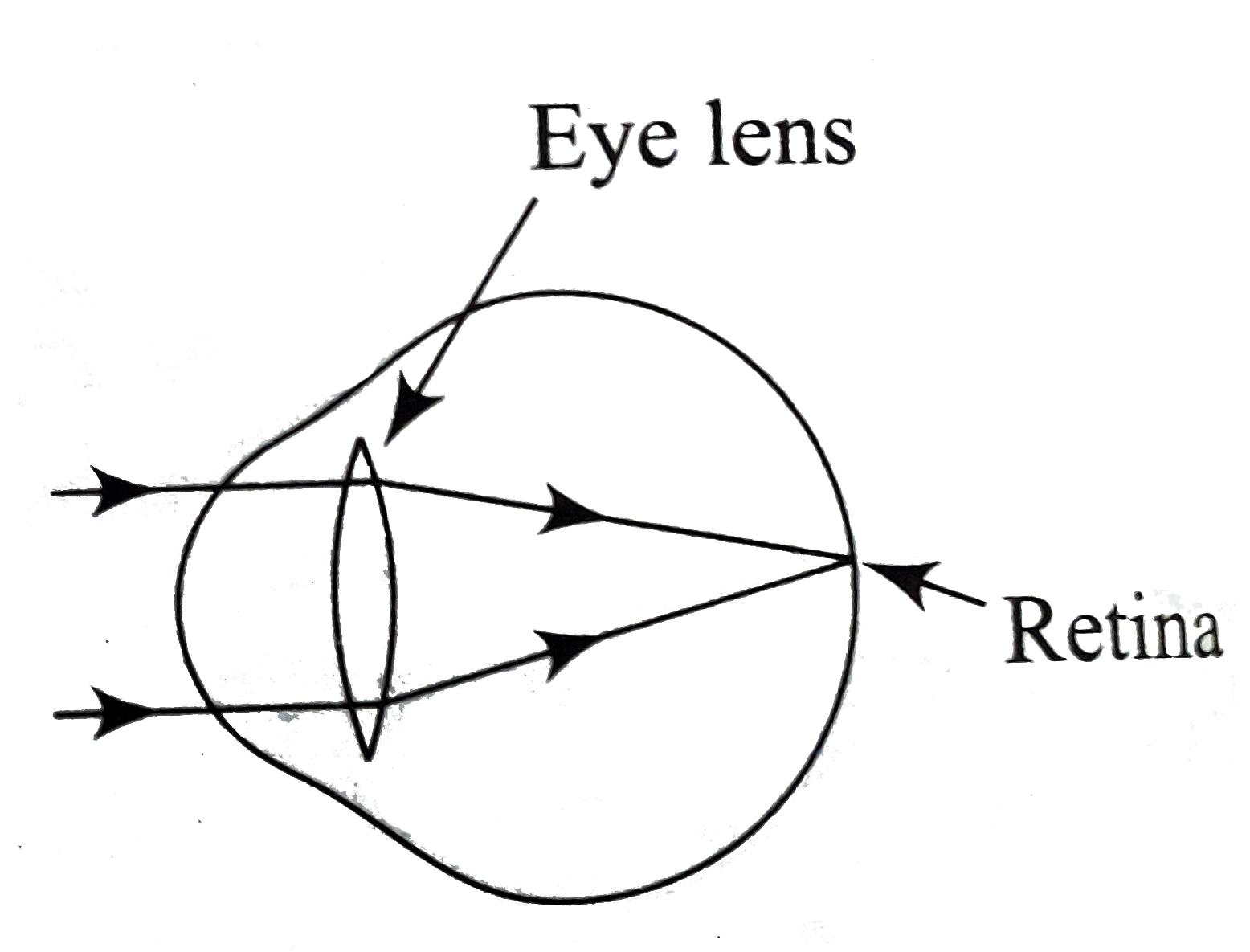
Q. A person can see objects clearly from distance 10cm to ∞. Then, we can say that the person isView SolutionThe ciliary muscles of eye control the curvature of the lens in the eye and hence can alter the effective focal length of the system. When the muscles are fully relaxed, the focal length is maximum. When the muscles are strained, the curvature of lens increases. That means radius of curvature decreases and focal length decreases. For a clear vision, the image must be on the retina. The image distance is therefore fixed for clear vision and it equals the distance of retina from eye lens. It is about 2.5cm for a grown up person.
A perosn can theoretically have clear vision of an object situated at any large distance from the eye. The smallest distance at which a person can clearly see is related to minimum possible focal length. The ciliary muscles are most strained in this position. For an average grown up person, minimum distance of the object should be around 25cm.
A person suffering from eye defects uses spectacles (eye glass). The function of lens of spectacles is to form the image of the objects within the range in which the person can see clearly. The image o the spectacle lens becomes object for the eye lens and whose image is formed on the retina.
The number of spectacle lens used for th eremedy of eye defect is decided by the power fo the lens required and the number of spectacle lens is equal to the numerical value of the power of lens with sign. For example, if power of the lens required is +3D (converging lens of focal length 100/3cm ), then number of lens will be +3.
For all the calculations required, you can use the lens formula and lensmaker's formula. Assume that the eye lens is equiconvex lens. Neglect the distance between the eye lens and the spectacle lens.
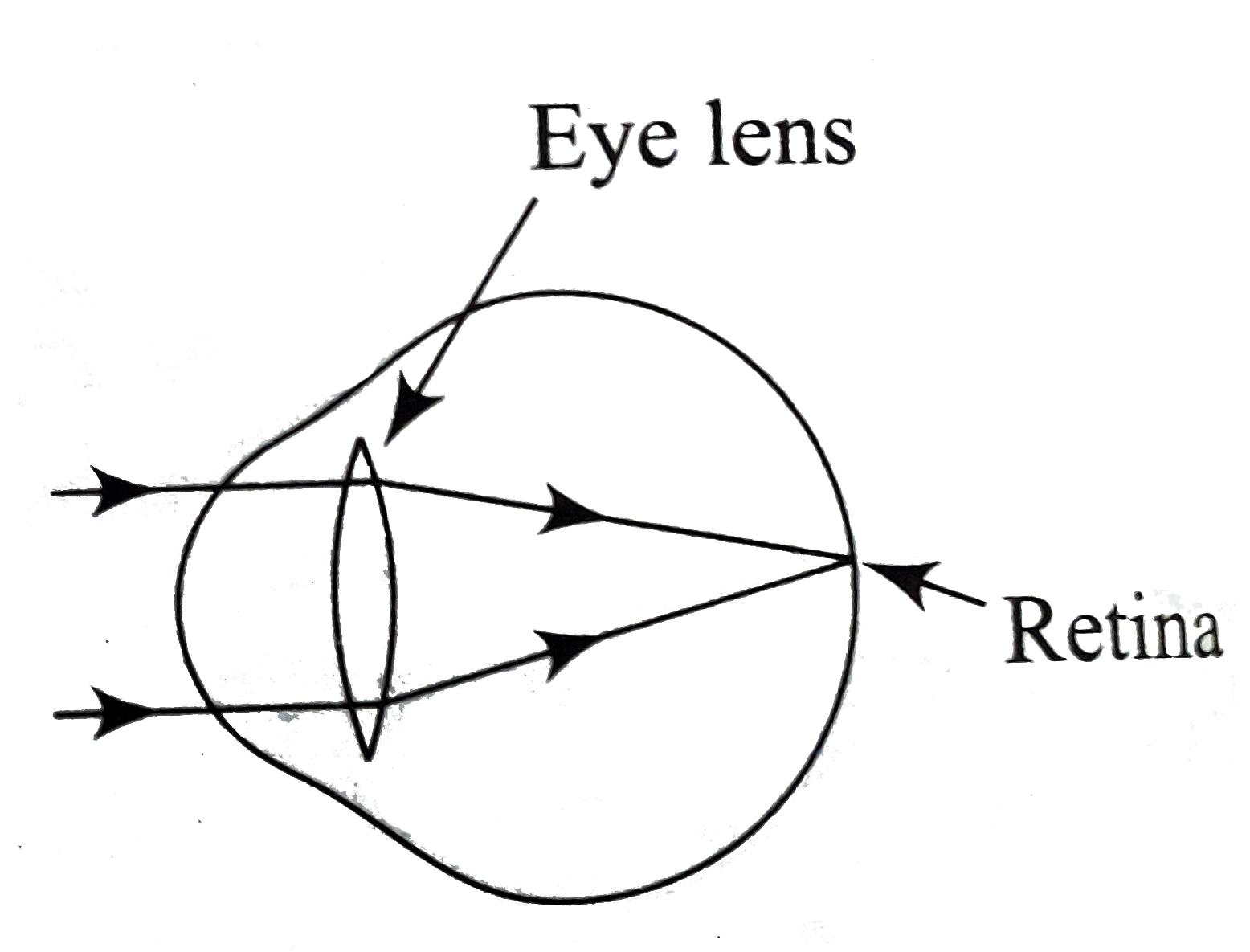
Q. Minimum focal length of eye lens of a normal peson isView SolutionThe ciliary muscles of eye control the curvature of the lens in the eye and hence can alter the effective focal length of the system. When the muscles are fully relaxed, the focal length is maximum. When the muscles are strained, the curvature of lens increases. That means radius of curvature decreases and focal length decreases. For a clear vision, the image must be on the retina. The image distance is therefore fixed for clear vision and it equals the distance of retina from eye lens. It is about 2.5cm for a grown up person.
A perosn can theoretically have clear vision of an object situated at any large distance from the eye. The smallest distance at which a person can clearly see is related to minimum possible focal length. The ciliary muscles are most strained in this position. For an average grown up person, minimum distance of the object should be around 25cm.
A person suffering from eye defects uses spectacles (eye glass). The function of lens of spectacles is to form the image of the objects within the range in which the person can see clearly. The image o the spectacle lens becomes object for the eye lens and whose image is formed on the retina.
The number of spectacle lens used for th eremedy of eye defect is decided by the power fo the lens required and the number of spectacle lens is equal to the numerical value of the power of lens with sign. For example, if power of the lens required is +3D (converging lens of focal length 100/3cm ), then number of lens will be +3.
For all the calculations required, you can use the lens formula and lensmaker's formula. Assume that the eye lens is equiconvex lens. Neglect the distance between the eye lens and the spectacle lens.
Q. Maximum focal length of a eye lens of a normal person isView SolutionA person cannot see beyond a distance of 50 cm.The power of corrective lens required to see distant object is
View Solution

