An electronically driven loudspeaker is placed near the open end of a resonance column apparatus. The length of air column in the tube is 80 cm. The frequency of the loudspeaker can be varied between 20 Hz and 2 kHz. Find the frequencies at which the column will resonate. Speed of sound in air =320ms−1
The correct Answer is:A, B, C
To find the resonant frequencies of the air column in the resonance column apparatus, we can follow these steps:
Step 1: Identify the parameters
- Length of the air column (L) = 80 cm = 0.8 m
- Speed of sound in air (V) = 320 m/s
Step 2: Use the formula for the fundamental frequency of a closed organ pipe
The fundamental frequency (ν₀) for a closed organ pipe is given by the formula:
ν₀=V4L
Step 3: Calculate the fundamental frequency
Substituting the values into the formula:
ν₀=320m/s4×0.8m=3203.2=100Hz
Step 4: Determine the resonant frequencies
The resonant frequencies (ν) for a closed organ pipe can be calculated using the formula:
ν=(2n+1)ν₀
where n is a non-negative integer (0, 1, 2, ...).
Step 5: Calculate the resonant frequencies for different values of n
1. For n=0:
ν0=(2×0+1)×100=100Hz
2. For n=1:
ν1=(2×1+1)×100=300Hz
3. For n=2:
ν2=(2×2+1)×100=500Hz
4. For n=3:
ν3=(2×3+1)×100=700Hz
5. For n=4:
ν4=(2×4+1)×100=900Hz
6. For n=5:
ν5=(2×5+1)×100=1100Hz
7. For n=6:
ν6=(2×6+1)×100=1300Hz
8. For n=7:
ν7=(2×7+1)×100=1500Hz
9. For n=8:
ν8=(2×8+1)×100=1700Hz
10. For n=9:
ν9=(2×9+1)×100=1900Hz
11. For n=10:
ν10=(2×10+1)×100=2100Hz(not valid, exceeds 2 kHz)
Step 6: List the valid resonant frequencies
The valid resonant frequencies within the range of 20 Hz to 2000 Hz are:
- 100 Hz
- 300 Hz
- 500 Hz
- 700 Hz
- 900 Hz
- 1100 Hz
- 1300 Hz
- 1500 Hz
- 1700 Hz
- 1900 Hz
Final Answer:
The frequencies at which the column will resonate are:
- 100 Hz, 300 Hz, 500 Hz, 700 Hz, 900 Hz, 1100 Hz, 1300 Hz, 1500 Hz, 1700 Hz, and 1900 Hz.
- Length of the air column (L) = 80 cm = 0.8 m
- Speed of sound in air (V) = 320 m/s
The fundamental frequency (ν₀) for a closed organ pipe is given by the formula:
ν₀=V4L
Substituting the values into the formula:
ν₀=320m/s4×0.8m=3203.2=100Hz
The resonant frequencies (ν) for a closed organ pipe can be calculated using the formula:
ν=(2n+1)ν₀
where n is a non-negative integer (0, 1, 2, ...).
1. For n=0:
ν0=(2×0+1)×100=100Hz
2. For n=1:
ν1=(2×1+1)×100=300Hz
3. For n=2:
ν2=(2×2+1)×100=500Hz
4. For n=3:
ν3=(2×3+1)×100=700Hz
5. For n=4:
ν4=(2×4+1)×100=900Hz
6. For n=5:
ν5=(2×5+1)×100=1100Hz
7. For n=6:
ν6=(2×6+1)×100=1300Hz
8. For n=7:
ν7=(2×7+1)×100=1500Hz
9. For n=8:
ν8=(2×8+1)×100=1700Hz
10. For n=9:
ν9=(2×9+1)×100=1900Hz
11. For n=10:
ν10=(2×10+1)×100=2100Hz(not valid, exceeds 2 kHz)
The valid resonant frequencies within the range of 20 Hz to 2000 Hz are:
- 100 Hz
- 300 Hz
- 500 Hz
- 700 Hz
- 900 Hz
- 1100 Hz
- 1300 Hz
- 1500 Hz
- 1700 Hz
- 1900 Hz
The frequencies at which the column will resonate are:
- 100 Hz, 300 Hz, 500 Hz, 700 Hz, 900 Hz, 1100 Hz, 1300 Hz, 1500 Hz, 1700 Hz, and 1900 Hz.
Topper's Solved these Questions
Similar Questions
A cylindrical metal tube has a length of 50 cm and is open at both ends. Find the frequencies between 1000 Hz and 2000 Hz at which the air column in the tube can resonate. Speed of sound in air is 340ms−1.
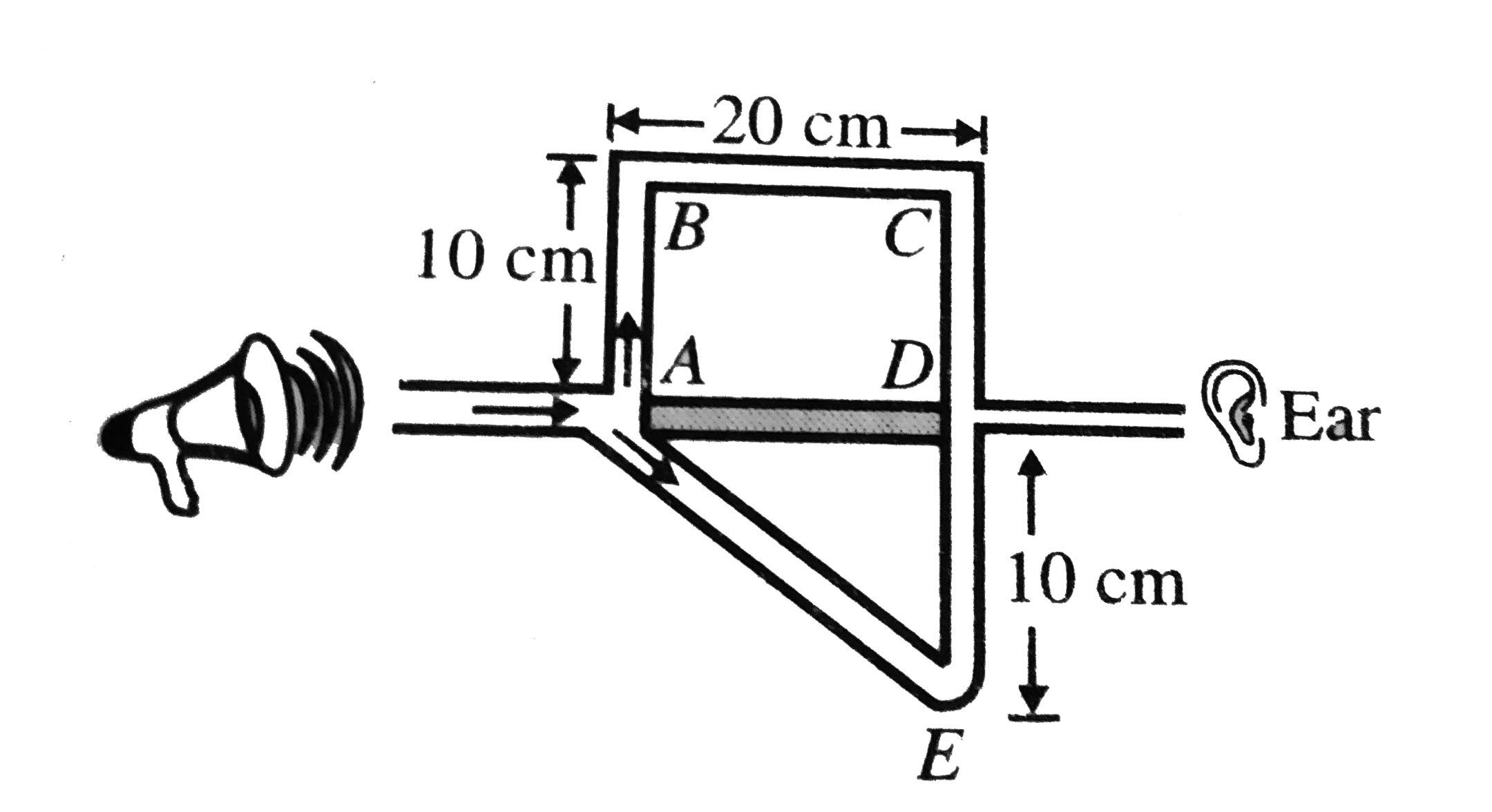
View SolutionFigure 7.20 shows a tube structure in which signal is sent from one end and is received at the other end . The frequency of the sound source can be varied electronically between 2000→5000Hz. Find the frequencies at which maxima of intensity are detected . The speed of sound in air 340m/s.
View SolutionA closed organ pipe can vibrate at a minimum frequency of 500 Hz. Find the length of the tube. Speed of sound in air =340ms−1.
View SolutionTwo successive resonance frequencies in an open organ pipe are 1944 Hz and 2592 Hz. Find the length of the tube. The speed of sound in air is 324ms−1.
View SolutionFind the fundamental, first overtone and second overtone frequencies of an open organ pipe of length 20 cm. Speed of sound in air is 340ms−1.
View SolutionA standing tuning fork of frequency f is used to find the velocity of sound in air by resonance column appartus. The difference two resonating lengths is 1.0m. Then the velocity of sound in air is
View Solutiontwo pipes have each of length 2 m, one is closed at on end and the other is open at both ends. The speed of sound in air is 340 m/s . The frequency at which both can resonate is ?
View SolutionThe speed of sound in an air column of 80cm closed at one end is 320m/s. Find the natural freqencies of air column between 20Hz and 2000Hz.
View SolutionA pipe 1 m length is closed at one end. Taking the speed of sound in air as 320ms−1 .the air column in the pipe cannot resonate for the frequency (in Hz )
View SolutionIn a resonance column experiment the frequency of tuning fork used is 1000Hz and the length of pipe is 100m. Ignoring end correction find the length (in cm) of air column at which second resonance is observed. [Take speed of sound =330m/s]
View Solution
