When an object cools down, heat is withdrawn from it. Does the entropy of the object decrease in this process? If yes, is it a violation of the second law of thermodynamics stated in terms of increase in entropy?
Step by step video & image solution for When an object cools down, heat is withdrawn from it. Does the entropy of the object decrease in this process? If yes, is it a violation of the second law of thermodynamics stated in terms of increase in entropy? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The potential energy of a freely falling object decreases progressively. Does this violate the law of conservation of energy ? Why ?
View SolutionAssertion : When a glass of hot milk is placed in a room and allowed to cool, its entropy decreases.
Reason : Allowing hot object to cool does not violate the second law of thermodynamics.
View SolutionWhen we heat an object, it expands. Is work done by the object in this process? Is heat given to the object equal to the increase in its internal energy?
View SolutionDuring winters, moisture condenses in the form of dew and can be seen on plant leaves and grass. The entropy of the system in such cases decreases as liquids process lesser disorder as compared to gases. With reference to the second law, which statement is correct. for the above process?
View SolutionDuring winters, moisture condenses in the form of dew and can be seen on plant leaves and grass. The entropy of the system in such cases decreases as liquids possess lesser disorder as compared to gases. With reference to the second law, which statement in correct, for the above process?
View SolutionSome statements are given with regard to entropy. The incorrect statement(s) are
(A) The absolute entropy of substances cannot be determined
(B) In standard state entropy of elements is always positive
(C ) The entropy of universe always decreases
(D) In a spontaneous process, for an isolated system the entropy of the system generally increases
View SolutionAs per second law of thermodynamics a process taken place spontaneously if and only if the entropy of the universe increases due to the process. Change in entropy is given by
ΔS=QrevT
What is the change in entropy of the universe due to the following reaction occuring at 27∘C ?
A+2B→C+2D,ΔH=+1.8kJmol−1
Molar entropy values of A, B, C and D are 1,2,3 and 4JK−1mol−1 respectively.
View SolutionIf an object is released in air, the magnitude of its acceleration would begain at the free-fall value, but it would decrease continously to zero as the object continued to fall.
for which one of the choices given does the solid line best represent the speed of the object as a function of time when it is dropped from rest in air?
Note The deshed line shows the free-fall under vacuum graph for comparison.
A.
B.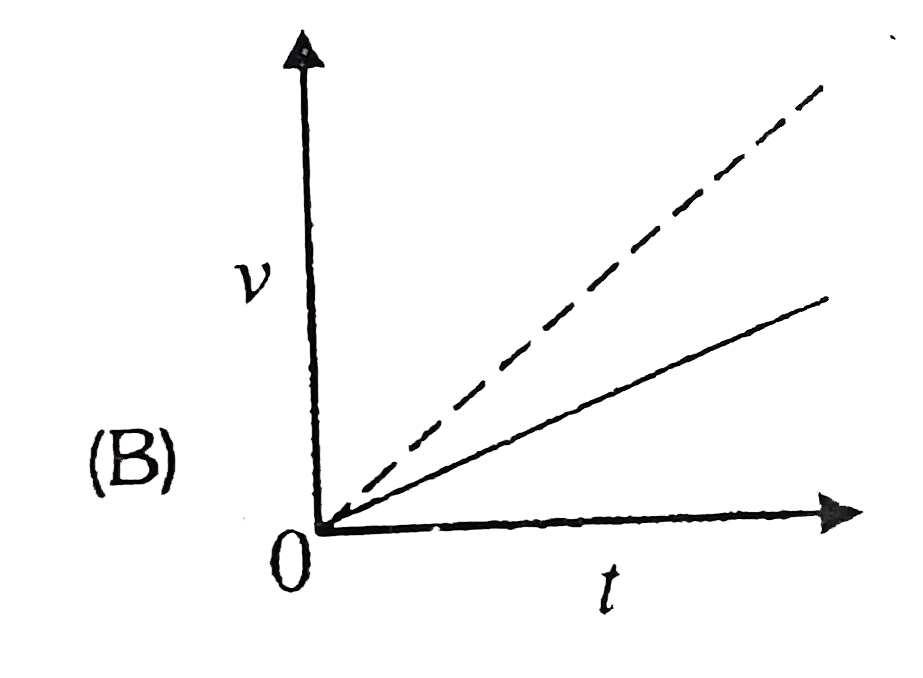
C.
D.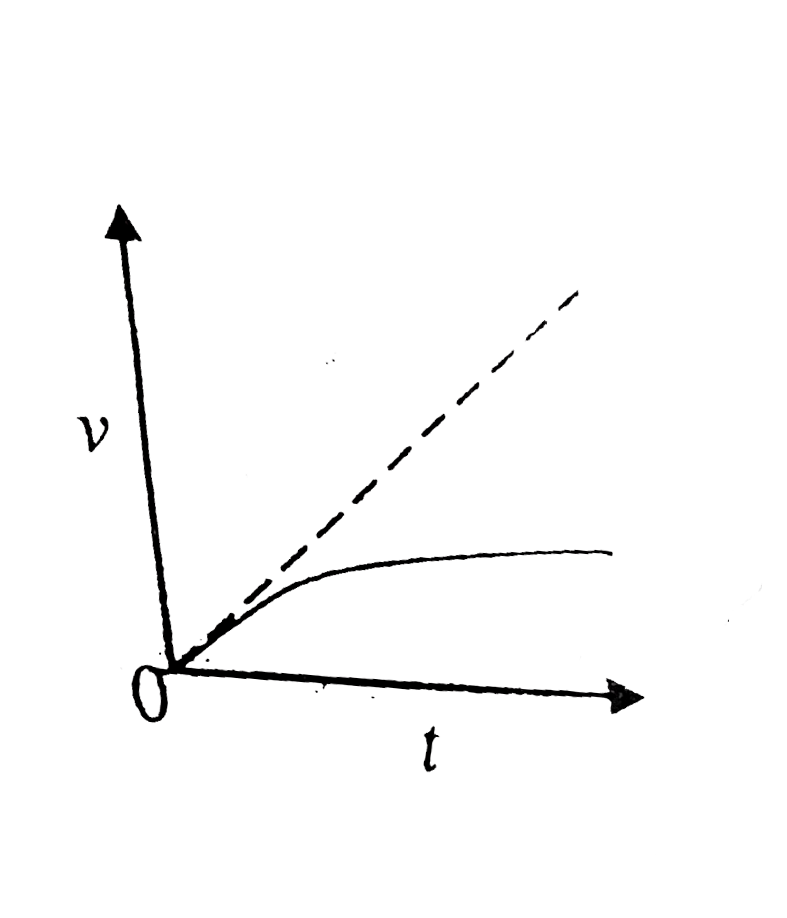
View SolutionDirection :
Resistive force proportional to object velocity
At low speeds, the resistive force acting on an object that is moving a viscous medium is effectively modeleld as being proportional to the object velocity. The mathematical representation of the resistive force can be expressed as
R=−bv
Where v is the velocity of the object and b is a positive constant that depends onthe properties of the medium and on the shape and dimensions of the object. The negative sign represents the fact that the resistance froce is opposite to the velocity.
Consider a sphere of mass m released frm rest in a liquid. Assuming that the only forces acting on the spheres are the resistive froce R and the weight mg, we can describe its motion using Newton's second law. though the buoyant force is also acting on the submerged object the force is constant and effect of this force be modeled by changing the apparent weight of the sphere by a constant froce, so we can ignore it here.
Thus mg−bv=mdvdt⇒dvdt=g−bmv
Solving the equation
v=mgb(1−e−bt/m)
where e=2.71 is the base of the natural logarithm
The acceleration becomes zero when the increasing resistive force eventually the weight. At this point, the object reaches its terminals speed v1 and then on it continues to move with zero acceleration
mg−bT=0
⇒mT=mgb
Hence v=vT(1−evtm)
In an experimental set-up four objects I,II,III,IV were released in same liquid. Using the data collected for the subsequent motions value of constant b were calculated. Respective data are shown in table.
ObjectIIIIIIVMass (in kg.)1234Constant bin (N-s)/m3.71.41.42.8
If an object of mass 2 kg and constant b=4N−sm has terminal speed vT in a liquid then time required to reach 0.63vT from start of the motion is :
View SolutionDirection :
Resistive force proportional to object velocity
At low speeds, the resistive force acting on an object that is moving a viscous medium is effectively modeleld as being proportional to the object velocity. The mathematical representation of the resistive force can be expressed as
R=−bv
Where v is the velocity of the object and b is a positive constant that depends on the properties of the medium and on the shape and dimensions of the object. The negative sign represents the fact that the resistance froce is opposite to the velocity.
Consider a sphere of mass m released frm rest in a liquid. Assuming that the only forces acting on the spheres are the resistive froce R and the weight mg, we can describe its motion using Newton's second law. though the buoyant force is also acting on the submerged object the force is constant and effect of this force be modeled by changing the apparent weight of the sphere by a constant froce, so we can ignore it here.
Thus mg−bv=mdvdt⇒dvdt=g−bmv
Solving the equation
v=mgb(1−e−bt/m)
where e=2.71 is the base of the natural logarithm
The acceleration becomes zero when the increasing resistive force eventually the weight. At this point, the object reaches its terminals speed v1 and then on it continues to move with zero acceleration
mg−bT=0
⇒mT=mgb
Hence v=vT(1−evtm)
In an experimental set-up four objects I,II,III,IV were released in same liquid. Using the data collected for the subsequent motions value of constant b were calculated. Respective data are shown in table.
ObjectIIIIIIVMass (in kg.)1234Constant bin (N-s)/m3.71.41.42.8
Which object would first acquire half of their respective terminal speed in minimum time from start of the motion of all were released simultaneously ?
View Solution