Three samples A, B and C of the same gas (γ=1.5) have equal volumes and temperatures. The volume of each sample is doubled, the process being isothermal for A, adiabtic for B and isobaric for C. If the final pressures are equal for the three samples, Find the ratio of the initial pressures.
To solve the problem, we need to analyze the three processes (isothermal, adiabatic, and isobaric) and use the ideal gas law and the properties of each process to find the initial pressures of the gas samples A, B, and C.
Step 1: Understand the initial conditions
Let the initial pressures of samples A, B, and C be PA, PB, and PC respectively. The initial volume V and temperature T are the same for all three samples.
Step 2: Analyze the isothermal process for sample A
In an isothermal process, the pressure and volume are related by the equation:
PAV=nRT
When the volume is doubled (i.e., V becomes 2V), the final pressure P′A can be expressed as:
P′A⋅(2V)=nRT
Since the temperature remains constant, we can equate the two:
P′A=PAV2V=PA2
Given that the final pressure for all samples is equal to P0, we have:
P′A=P0⟹PA=2P0
Step 3: Analyze the adiabatic process for sample B
For an adiabatic process, the relationship between pressure and volume is given by:
PBVγ=P′B(2V)γ
where γ=1.5. Thus:
PBV1.5=P0(2V)1.5
This simplifies to:
PBV1.5=P0⋅21.5V1.5
Cancelling V1.5 from both sides gives:
PB=P0⋅21.5=P0⋅2√2
Step 4: Analyze the isobaric process for sample C
In an isobaric process, the pressure remains constant. Therefore:
P′C=PC=P0
Step 5: Set up the ratio of initial pressures
Now we have:
- PA=2P0
- PB=2√2P0
- PC=P0
The ratio of the initial pressures PA:PB:PC is:
PA:PB:PC=2P0:2√2P0:P0
Dividing through by P0:
=2:2√2:1
Final Ratio
Thus, the final ratio of the initial pressures is:
2:2√2:1
Let the initial pressures of samples A, B, and C be PA, PB, and PC respectively. The initial volume V and temperature T are the same for all three samples.
In an isothermal process, the pressure and volume are related by the equation:
PAV=nRT
When the volume is doubled (i.e., V becomes 2V), the final pressure P′A can be expressed as:
P′A⋅(2V)=nRT
Since the temperature remains constant, we can equate the two:
P′A=PAV2V=PA2
Given that the final pressure for all samples is equal to P0, we have:
P′A=P0⟹PA=2P0
For an adiabatic process, the relationship between pressure and volume is given by:
PBVγ=P′B(2V)γ
where γ=1.5. Thus:
PBV1.5=P0(2V)1.5
This simplifies to:
PBV1.5=P0⋅21.5V1.5
Cancelling V1.5 from both sides gives:
PB=P0⋅21.5=P0⋅2√2
In an isobaric process, the pressure remains constant. Therefore:
P′C=PC=P0
Now we have:
- PA=2P0
- PB=2√2P0
- PC=P0
PA:PB:PC=2P0:2√2P0:P0
Dividing through by P0:
=2:2√2:1
Thus, the final ratio of the initial pressures is:
2:2√2:1
Topper's Solved these Questions
Similar Questions
Three samples of the same gas A,B and C (γ=3/2) have initially equal volume. Now the volume of each sample is doubled. The process is adiabatic for A. Isobaric for B and isothermal for C. If the final pressures are equal for all three samples, find the ratio of their initial pressures
View SolutionThree samples A, B and C of the same gas (γ=1.5) have equal volumes and temperatures. The volume of each sample is doubled, the process being isothermal for A, adiabatic for B and isobaric for C. If the final pressures are equal for the three samples, Find the ratio of the initial pressures.
View Solution
Knowledge Check
- Question 1 - Select One
Three samples of the same gas A,B and C (γ=3/2) have initially equal volume. Now the volume of each sample is doubled. The process is adiabatic for A. Isobaric for B and isothermal for C. If the final pressures are equal for all three samples, find the ratio of their initial pressures
A2:1:√2B2√2:1:2C√2:1:2D√2:2:1 - Question 2 - Select One
Three samples of the same gas A,B and C (γ=3/2) have initially equal volume. Now the volume of each sample is doubled. The process is adiabatic for A. Isobaric for B and isothermal for C. If the final pressures are equal for all three samples, find the ratio of their initial pressures
A2:1:√2B2√2:1:2C√2:1:2D√2:2:1 - Question 3 - Select One
A sample of an ideal gas occupies a volume V at pressure P and absolute temperature T. The masss of each molecule is m, then the density of the gas is
AmKTBPmKTCPkmDPKT
Two gases have the same initial pressure, volume and temperature. They expand to the same final volume, one adiabatically and the other isothermally
View SolutionA gas for which γ=1.5 is suddenly compressed to 1/4 th of the initial volume. Then the ratio of the final to initial pressure is
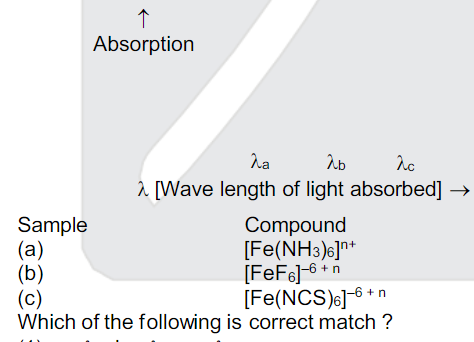
View SolutionThe absorption spectra of three samples A,B,C is given:
 View Solution
View SolutionIf the aboslute temperature of a gas having volume Vcm3 is doubled and the pressure is reduced to half, the final volume will be
View SolutionIn a process on a system, the initial pressure and volume are equal to final pressure and volume, then
View Solution

