Two neutral particles are kept 1m apart. Suppose by some mechanism some charge is transferred from one particle to the other and the electric potential energy lost is completely converted into a photon. Calculate the longest and the next smaller wavelength of the photon possible. h=6.63×10−34Js.
To solve the problem, we need to calculate the electric potential energy lost when a charge is transferred between two neutral particles and how this energy is converted into the energy of a photon. We will find the longest and the next smaller wavelength of the photon possible.
1. Understanding Electric Potential Energy:
The electric potential energy (U) between two point charges +q and −q separated by a distance r is given by:
U=14πϵ0q2r
where ϵ0 is the permittivity of free space.
2. Energy of a Photon:
The energy (E) of a photon is related to its wavelength (λ) by the equation:
E=hcλ
where h is Planck's constant and c is the speed of light.
3. Setting the Energies Equal:
Since the electric potential energy lost is converted into the energy of the photon, we can set these two equations equal:
14πϵ0q2r=hcλ
4. Rearranging for Wavelength:
Rearranging the equation to solve for λ:
λ=hc⋅4πϵ0⋅rq2
5. Substituting Known Values:
- h=6.63×10−34Js
- c=3×108m/s
- ϵ0=8.85×10−12C2/N m2
- r=1m
- The smallest charge q=1.6×10−19C
Plugging in these values:
λ=(6.63×10−34)⋅(3×108)⋅(4π⋅8.85×10−12)⋅1(1.6×10−19)2
6. Calculating the Longest Wavelength:
First, calculate the denominator:
(1.6×10−19)2=2.56×10−38
Now calculate the numerator:
4π⋅8.85×10−12≈1.11×10−10
Therefore,
λ=(6.63×10−34)⋅(3×108)⋅(1.11×10−10)2.56×10−38
After performing the calculations, we find:
λ≈863m
7. Calculating the Next Smaller Wavelength:
For the next smaller wavelength, we consider a charge of 2q:
λ=hc⋅4πϵ0⋅r(2q)2=hc⋅4πϵ0⋅r4q2=14⋅hc⋅4πϵ0⋅rq2
Thus, the next wavelength will be:
λ≈8634≈215.75m
Final Answers:
- Longest wavelength: λ1≈863m
- Next smaller wavelength: λ2≈215.75m
The electric potential energy (U) between two point charges +q and −q separated by a distance r is given by:
U=14πϵ0q2r
where ϵ0 is the permittivity of free space.
The energy (E) of a photon is related to its wavelength (λ) by the equation:
E=hcλ
where h is Planck's constant and c is the speed of light.
Since the electric potential energy lost is converted into the energy of the photon, we can set these two equations equal:
14πϵ0q2r=hcλ
Rearranging the equation to solve for λ:
λ=hc⋅4πϵ0⋅rq2
- h=6.63×10−34Js
- c=3×108m/s
- ϵ0=8.85×10−12C2/N m2
- r=1m
- The smallest charge q=1.6×10−19C
λ=(6.63×10−34)⋅(3×108)⋅(4π⋅8.85×10−12)⋅1(1.6×10−19)2
First, calculate the denominator:
(1.6×10−19)2=2.56×10−38
Now calculate the numerator:
4π⋅8.85×10−12≈1.11×10−10
Therefore,
λ=(6.63×10−34)⋅(3×108)⋅(1.11×10−10)2.56×10−38
λ≈863m
For the next smaller wavelength, we consider a charge of 2q:
λ=hc⋅4πϵ0⋅r(2q)2=hc⋅4πϵ0⋅r4q2=14⋅hc⋅4πϵ0⋅rq2
Thus, the next wavelength will be:
λ≈8634≈215.75m
- Longest wavelength: λ1≈863m
- Next smaller wavelength: λ2≈215.75m
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYEXERCISE - question for short answer13videos
PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITY
- BOOK - HC VERMA ENGLISHCHAPTER - PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITYEXERCISE - objective II7videos
PHOTO ELECTRIC EFFECT AND WAVE PARTICLE DUALITY
- BOOK - HC VERMA ENGLISHCHAPTER - PERMANENT MAGNETSEXERCISE - Short Answer11videos
PERMANENT MAGNETS
- BOOK - HC VERMA ENGLISHCHAPTER - PHOTOMETRYEXERCISE - All Questions38videos
PHOTOMETRY
Similar Questions
Calculate the momentum of the particle which has de Broglie wavelength 1Ã…(10−10m) and h=6.6×10−34J−sec.
View SolutionCalculate the momentum of a particle which has a de Broglie wavelength of 2Å,(h=6.6×10−34kgm2s−1)
View SolutionAn electron and a photon each has a wavelength of 1.0nm. Find (a) their momenta, (b) the energy of the photon , and © the kinetic energy electron.
Take h=6.63×10−34Js.View SolutionAn electron and a photon each have a wavelength of 2.00nm. Find (i) their momenta (ii) the energy of the photon and (iii) the kinetic energy of electron. h=6.63×10−34Js
View SolutionMonochromatic light of wavelength 632.8nm is produced by a helium-neon laser. The power emitted is 9.42mW. (a) Find the energy and momentum of each photon in the light beam. (b) How many photons per second, on the average, arrive at a target irradiated by this beam? (Assume the beam to have uniform cross-section which is less than the target area). (c) How fast does a hydrogen atom have to travel in order to have the same momentum as that of the photon. h=6.663×10−34Js,1a.m.u.=1.66×10−27kg.
View SolutionA thin uniform bar lies on a frictionless horizonta surface and is free to move in any way on th surface. Its mass is 0.16 kg and length √3meters. Two particless, each of mass 0.08 kg, are moving on the same surface and towards the bar in a direction perpendicular to the bar, one with a velocity of 10 m/s, and other with 6m/s as shown in fig. The first particle strikes the bar at point A and the other at point B. Points A and B are at a distane of 0.5m from the centre of the bar. The particles strike the bar at the same instant of time and stick to the bar on collision. Calculate the loss of the kinetic energy of the system in the above collision process.
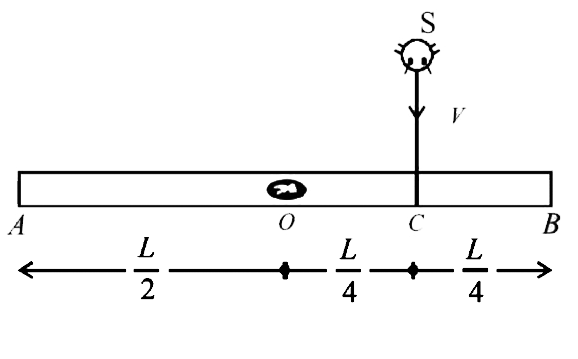 View Solution
View SolutionCalculate the number of photons emitted per second by a 10 watt sodium vapor lamp. Assume that 90% of the consumed energy is converted into light. Wavelength of sodium light is 590nm. h=6.62×10−34Js
View SolutionCalculate the number of photons emitted per second by a 10 watt sodium vapor lamp. Assume that 90% of the consumed energy is converted into light. Wavelength of sodium light is 590nm. h=6.62×10−34Js
View SolutionThe positron is a fundamental particle with the same mass as that of the electron and with a charge equal to that of an electron but of opposite sign. When a positron and an electron collide, they may annihilate each other. The energy corresponding to their mass appears in two photons of equal energy. Find the wavelength of the radiation emitted. [Take : mass of electron =(0.5/c2)MeV and hc=1.2×10−12MeV−m, where h is the Planck's constant and c is the velocity of light in air.
View SolutionThe energy of stable states of the hydrogen atom is given by En=−2.18×10−8/n2[J] where n denotes the principal quantum number.
Calculate the de Broglie wavelength of the electrons emitted from a copper crystal when irradiated by photons from the first line and the sixth line of the Lyman series.
h=6.6256×10−34Js, me=9.1091×1031kg, c=2.99792×108ms−1View Solution

