Consider the reaction.
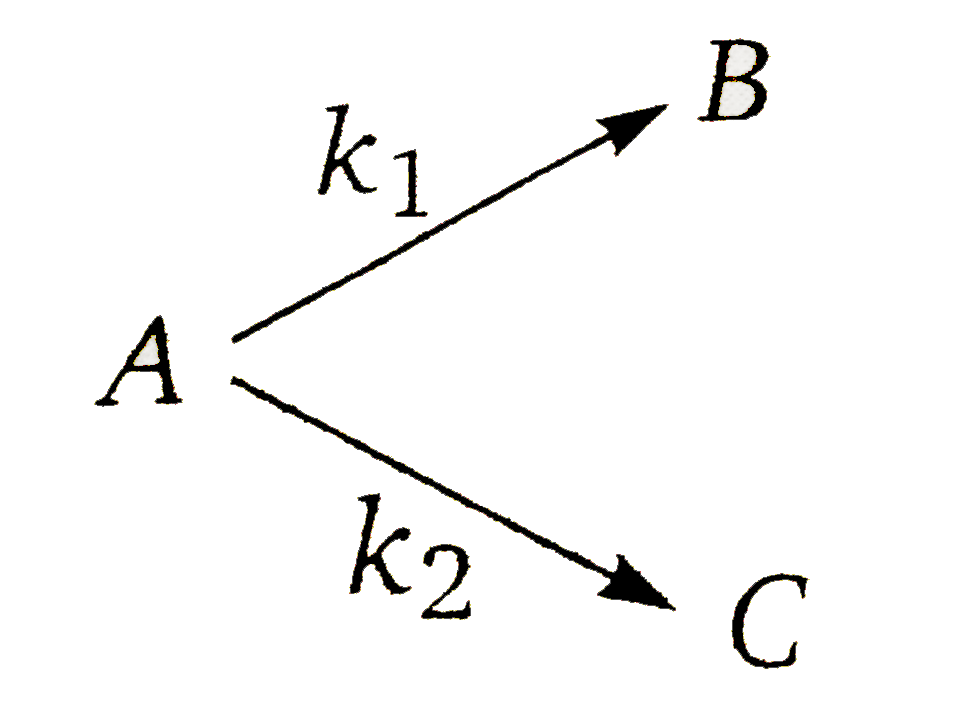
The rate constant for two parallel reactions were found to be and . If the corresponding energies of activation of the parallel reaction are 100 and 120 kJ/mol respectively, what is the net energy of activation of A
100 kJ/mol
120 kJ/mol
116 kJ/mol
220 kJ/mol
The correct Answer is:C
Step by step video & image solution for Consider the reaction. The rate constant for two parallel reactions were found to be 10^(-2) dm^(-2)mol^(-1)s^(-1) and 4xx10^(-2)dm^(-3)mol^(-1)s^(-1) . If the corresponding energies of activation of the parallel reaction are 100 and 120 kJ/mol respectively, what is the net energy of activation (E_(a)) of A by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Similar Questions
The substance undergoes first order decomposition.The decomposition follows two parallel first order reactons as :

K1=10−2sec−1andK2=4⋅10−2sec−1
If the corresponding activation energies of parallel reaction are 100 and 120 kJ mol−1 then the net activation energy of A is/are:
View SolutionThe value of rate constant for a second order reaction is 6.7×10−5mol−Ls− at 298 K and 1.64×10−4mol−Ls− at 313 K. Find the Arrhenius frequency factor A and activation energy of the reaction.
View SolutionThe value of rate constant for a second order reaction is 6.7×10−5mol−Ls− at 298 K and 1.64×10−4mol−Ls− at 313 K. Find the Arrhenius frequency factor A and activation energy of the reaction.
View SolutionThe value of rate constant for a second order reaction is 6.7×10−5mol−Ls− at 298 K and 1.64×10−4mol−Ls− at 313 K. Find the Arrhenius frequency factor A and activation energy of the reaction.
View SolutionThe activation energies of the forward and backward reactions in the case of a chemical reaction are 30.5 and 45.4kJ/mol respectively. The reaction is:
View SolutionThe rate constant of a reaction is 1.2×10−3s−1 at 30∘C and 2.1×10−3s−1 at 40∘C. Calculate the energy of activation of the reaction.
View SolutionRate constant k=1.2×103mol−1Ls−1 and Ea=2.0×102kJmol−1. When T→∞:
View SolutionIdentify the order of the reaction from the rate constant value =3.2×10−5Lmol−1s−1.
View SolutionIdentify the order of the reaction from the rate constant value =3.2×10−5Lmol−1s−1.
View SolutionThe rate constant for the reaction in gaseous phase
O+O3→2O2 is 8.0×10−15 cm3 molecule−1s−1 at 298 K. Corresponding value in dm3 mol−1s−1 is :
View Solution
