The energy of an electron moving in nth Bohr's orbit of an element is given by En=−13.6n2Z2 eV/ atom (Z=atomic number). The graph of E vs. Z2 (keeping "n" constant) will be :
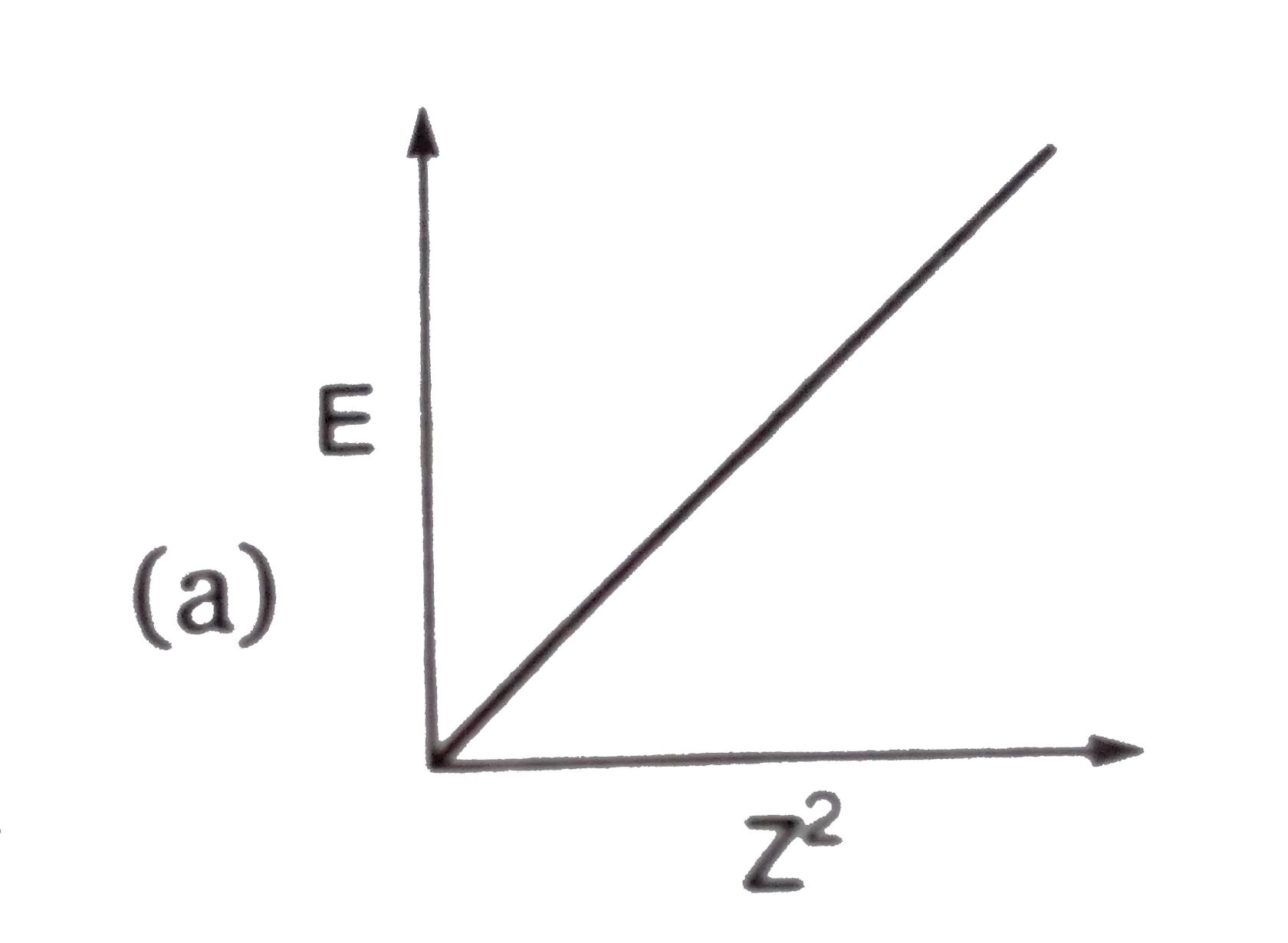
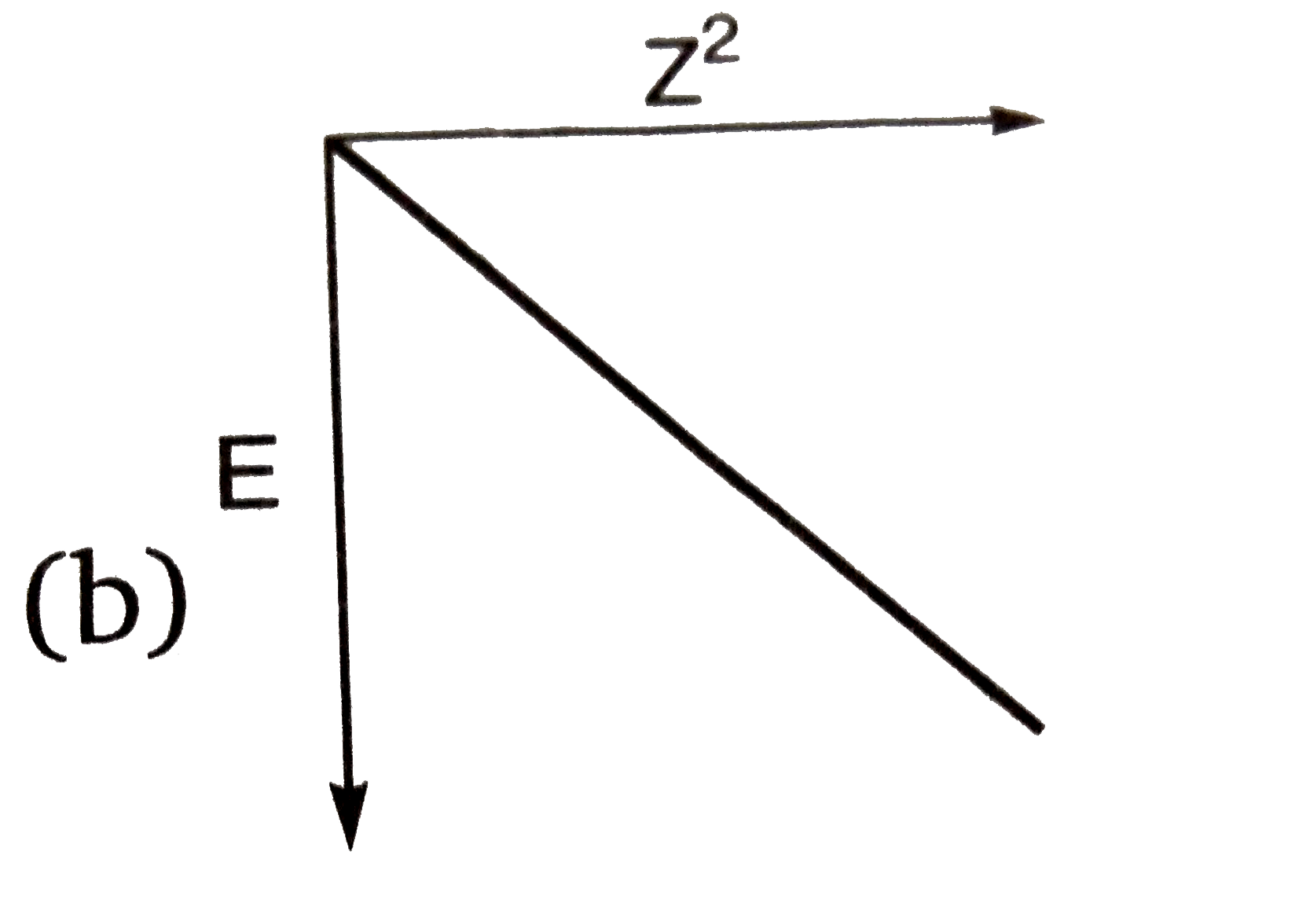
The correct Answer is:B
To solve the problem, we need to analyze the relationship between the energy of an electron in the nth Bohr orbit and the atomic number (Z) as given by the formula:
En=−13.6n2Z2
1. Identify the relationship:
The formula indicates that the energy En is proportional to Z2. This can be expressed as:
En∝−Z2
This means that as Z2 increases, En becomes more negative.
2. Rearranging the equation:
We can rewrite the equation in terms of Z2:
En=−13.6n2Z2
Here, we can see that if we plot En on the y-axis and Z2 on the x-axis, we can identify the slope of the line.
3. Identifying the slope:
The equation can be rearranged to the form:
En=m⋅Z2
where m=−13.6n2. Since m is negative, this indicates that the slope of the line is negative.
4. Graph characteristics:
Since the relationship is linear and the slope is negative, the graph of E versus Z2 will be a straight line that slopes downwards from left to right.
5. Conclusion:
Therefore, the graph of E versus Z2 (keeping n constant) will be a straight line with a negative slope.
Final Answer:
The correct graph of E versus Z2 is a straight line with a negative slope.
The formula indicates that the energy En is proportional to Z2. This can be expressed as:
En∝−Z2
This means that as Z2 increases, En becomes more negative.
We can rewrite the equation in terms of Z2:
En=−13.6n2Z2
Here, we can see that if we plot En on the y-axis and Z2 on the x-axis, we can identify the slope of the line.
The equation can be rearranged to the form:
En=m⋅Z2
where m=−13.6n2. Since m is negative, this indicates that the slope of the line is negative.
Since the relationship is linear and the slope is negative, the graph of E versus Z2 will be a straight line that slopes downwards from left to right.
Therefore, the graph of E versus Z2 (keeping n constant) will be a straight line with a negative slope.
The correct graph of E versus Z2 is a straight line with a negative slope.
Topper's Solved these Questions
- ATOMIC STUCTUREBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ATOMIC STUCTUREEXERCISE - level 2
30 Videos
- ATOMIC STUCTUREBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ATOMIC STUCTUREEXERCISE - Level 3 (Passage 1)
3 Videos
- CHEMICAL EQUILIBRIUMBOOK - NARENDRA AWASTHI ENGLISHCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Match the column
1 Videos
Similar Questions
Time period of revolution of an electron in nth orbit in a hydrogen like atom is given by T=T0naZb,Z=atomic number
View SolutionEnergy of the electron in nth orbit of hydrogen atom is given by En=−13.6n2eV. The amount of energy needed to transfer electron from first orbit to third orbit is
View Solution
Knowledge Check
The angular speed of the electron in the nth Bohr orbit of the hydrogen atom is proportional to
Adirectly proportional to nBinversely proportional to √nCinversely proportional to n2Dinversely proportional to n3
When an electron jumps from n1thorbit→n_(2) th orbit, the energy radiated is given by
View SolutionDerive an expression for the radius of nth Bohr’s orbit in Hydrogen atom.
View SolutionProve that En=−13.6×z2n2eV/a→m for nth orbit in single electron species.
View SolutionAccording to Bohr's theory, the electronic energy of an electron in the nth orbit is given by En=(−2.17×10−18)×z2n2J
Calculate the longest wavelength of light that will be needed in remove an electron from the third Bohr orbit of He⊕
View SolutionIn the nth orbit, the energy of an electron En=−13.6n2eV for hydrogen atom. The energy required to take the electron from first orbit to second orbit will be
View SolutionEnergy of electron in its nth orbit is given by (En=−13.6n2×z)ev . Consider a hydrogen atom , find the amount of energy needed to transfer electron from 1st orbit to 3rd orbit:
View SolutionThe energy of an electron in first Bohr's orbit of H atom is −13.6eV. The energy value of electron in the first excited state of Li2+ is :
View Solution
