The free energy change ΔG=0, when
the system is at equilibrium
catalyst is added
reactants are initially mixed thoroughly
the reactants are completely consumed
The correct Answer is:A
To determine when the free energy change (ΔG) is equal to zero, we can analyze the conditions under which this occurs. Here’s a step-by-step solution:
Step 1: Understanding Free Energy Change (ΔG)
Free energy change (ΔG) is a thermodynamic quantity that indicates the spontaneity of a reaction. It is defined as the difference between the free energy of the products and the free energy of the reactants.
Step 2: Equilibrium Condition
At equilibrium, the rates of the forward and reverse reactions are equal, and there is no net change in the concentrations of reactants and products. Under these conditions, the system has reached a state of balance.
Step 3: ΔG at Equilibrium
At equilibrium, the change in free energy (ΔG) is equal to zero. This can be expressed mathematically as:
ΔG=ΔG0+RTlnQ
Where:
- ΔG0 is the standard free energy change,
- R is the universal gas constant,
- T is the temperature in Kelvin,
- Q is the reaction quotient.
When the system is at equilibrium, Q equals the equilibrium constant K, and thus:
ΔG=0
Step 4: Analyzing Other Options
1. Catalyst Addition: Adding a catalyst speeds up the reaction but does not change the free energy change of the reaction. Therefore, ΔG is not zero just because a catalyst is added.
2. Reactants Mixed Thoroughly: Mixing reactants does not guarantee that the system is at equilibrium. Therefore, ΔG is not necessarily zero.
3. Reactants Completely Consumed: If reactants are completely consumed, products are formed, and there will still be free energy associated with the products. Hence, ΔG is not zero.
Conclusion
The only condition under which ΔG is equal to zero is when the system is at equilibrium.
Final Answer
ΔG = 0 when the system is at equilibrium.
---
Free energy change (ΔG) is a thermodynamic quantity that indicates the spontaneity of a reaction. It is defined as the difference between the free energy of the products and the free energy of the reactants.
At equilibrium, the rates of the forward and reverse reactions are equal, and there is no net change in the concentrations of reactants and products. Under these conditions, the system has reached a state of balance.
At equilibrium, the change in free energy (ΔG) is equal to zero. This can be expressed mathematically as:
ΔG=ΔG0+RTlnQ
Where:
- ΔG0 is the standard free energy change,
- R is the universal gas constant,
- T is the temperature in Kelvin,
- Q is the reaction quotient.
ΔG=0
1. Catalyst Addition: Adding a catalyst speeds up the reaction but does not change the free energy change of the reaction. Therefore, ΔG is not zero just because a catalyst is added.
2. Reactants Mixed Thoroughly: Mixing reactants does not guarantee that the system is at equilibrium. Therefore, ΔG is not necessarily zero.
The only condition under which ΔG is equal to zero is when the system is at equilibrium.
ΔG = 0 when the system is at equilibrium.
Topper's Solved these Questions
Similar Questions
The free energy change, ΔG = 0, when
View SolutionWhat is the free energy change, 'ΔG' When 1.0 mole of water at 100∘C and 1 atm pressure is converted into steam at 100∘C and 1 atm pressure
View SolutionThe relationship between the free energy change (ΔG) and entropy change (ΔS) at constant temperature (T) si
View SolutionA reaction is spontaneous if free energy change ΔG , for the reaction is negative . The question arises, how we can explain , the spontaneous of reversible reaction taking the example of dissociation equilibrium N2O4⇔2NO2(g) the variation of free energy with the fraction of N2O4 dissociated under standard conditions is shown in the figure below.
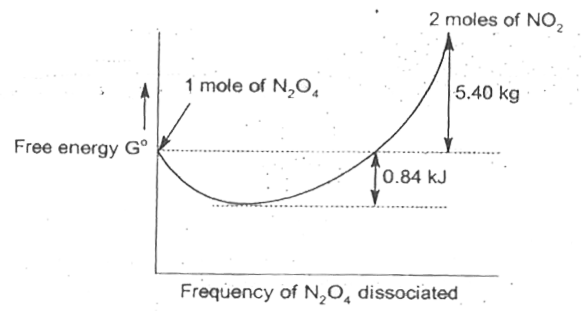
When 2 moles of NO2 change into equilibrium mixture the value of ΔG∘ is
View SolutionFor the reaction, NH4Cl(s)→NH3(g)+HCl(g) at 25∘C, enthalpy change ΔH=+177kJmol−1 and entropy change ΔS=+285Jmol−1K−1. Calculate the free energy change ΔG at 25∘C and predict whether the reaction is spontaneous or not.
View SolutionWhat is the free energy change (ΔG) for galvanic and electrolytic cel ?
View SolutionFor a chemical reaction, the free energy change (ΔG) is negative. The reaction is
View SolutionThe equilibrium constant Kp for the homogeneous reaction is 10−3. The standard Gibbs free energy change ΔGɵ for the reaction at 27∘C(usingR=2calK−1mol−1) is
View SolutionThe equilibrium constant Kp for a homogeneous gaseous reaction is 10−8. The standard Gibbs free energy change ΔGɵ for the reaction (usingR=2calK−1mol−1) is
View SolutionA reaction is spontaneous if free energy change ΔG for the reaction is negative . The question arises, how we can explain , the spontaneous reversible reaction. Taking the example of dissociation equilibrium N2O4⇔2NO2(g) the variation of free energy with the fraction of N2O4 dissociated under standard conditions is shown in the figure below.
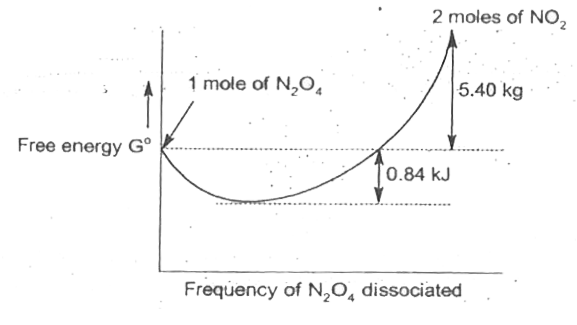
When 1 mole of N2O4 changes into the equilibrium mixture the value of ΔG∘ is
View Solution
