From the following ΔH∘ and ΔS∘ values, predict which of reactions I, II and III would be spontaneous at 25∘C.
ΔH∘(kJ)ΔS∘(J/K)I.+10.5+30II.+1.8−113III.−126+84
III
I
II and III
I and II
The correct Answer is:A
To determine which of the reactions I, II, and III are spontaneous at 25°C, we need to calculate the Gibbs free energy change (ΔG) for each reaction using the following formula:
ΔG=ΔH−TΔS
Where:
- ΔG = Gibbs free energy change
- ΔH = Change in enthalpy (in joules)
- T = Temperature in Kelvin
- ΔS = Change in entropy (in joules per Kelvin)
Step 1: Convert the temperature to Kelvin.
- Given temperature = 25°C
- Convert to Kelvin:
T=25+273=298 K
Step 2: Calculate ΔG for Reaction I.
- Given:
- ΔH = +10.5 kJ = +10.5 × 1000 J = +10500 J
- ΔS = +30 J/K
- Substitute into the Gibbs free energy equation:
ΔGI=10500 J−(298 K×30 J/K)
=10500 J−8940 J=1560 J
- Since ΔG_I is positive, Reaction I is non-spontaneous.
Step 3: Calculate ΔG for Reaction II.
- Given:
- ΔH = +1.8 kJ = +1.8 × 1000 J = +1800 J
- ΔS = -113 J/K
- Substitute into the Gibbs free energy equation:
ΔGII=1800 J−(298 K×−113 J/K)
=1800 J+33764 J=35564 J
- Since ΔG_{II} is positive, Reaction II is non-spontaneous.
Step 4: Calculate ΔG for Reaction III.
- Given:
- ΔH = -126 kJ = -126 × 1000 J = -126000 J
- ΔS = +84 J/K
- Substitute into the Gibbs free energy equation:
ΔGIII=−126000 J−(298 K×84 J/K)
=−126000 J−25032 J=−151032 J
- Since ΔG_{III} is negative, Reaction III is spontaneous.
Conclusion:
- Reaction I: Non-spontaneous (ΔG > 0)
- Reaction II: Non-spontaneous (ΔG > 0)
- Reaction III: Spontaneous (ΔG < 0)
Thus, the only spontaneous reaction at 25°C is Reaction III.
- ΔG = Gibbs free energy change
- ΔH = Change in enthalpy (in joules)
- T = Temperature in Kelvin
- ΔS = Change in entropy (in joules per Kelvin)
- Given temperature = 25°C
- Convert to Kelvin:
T=25+273=298 K
- Given:
- ΔH = +10.5 kJ = +10.5 × 1000 J = +10500 J
- ΔS = +30 J/K
- Substitute into the Gibbs free energy equation:
ΔGI=10500 J−(298 K×30 J/K)
=10500 J−8940 J=1560 J
- Since ΔG_I is positive, Reaction I is non-spontaneous.
- Given:
- ΔH = +1.8 kJ = +1.8 × 1000 J = +1800 J
- ΔS = -113 J/K
- Substitute into the Gibbs free energy equation:
ΔGII=1800 J−(298 K×−113 J/K)
=1800 J+33764 J=35564 J
- Since ΔG_{II} is positive, Reaction II is non-spontaneous.
- Given:
- ΔH = -126 kJ = -126 × 1000 J = -126000 J
- ΔS = +84 J/K
- Substitute into the Gibbs free energy equation:
ΔGIII=−126000 J−(298 K×84 J/K)
=−126000 J−25032 J=−151032 J
- Since ΔG_{III} is negative, Reaction III is spontaneous.
- Reaction I: Non-spontaneous (ΔG > 0)
- Reaction II: Non-spontaneous (ΔG > 0)
- Reaction III: Spontaneous (ΔG < 0)
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Which of the following must be true?
(I) The area of triangle P.
(II) The area of triangle Q.
(III) The area of triangle R.
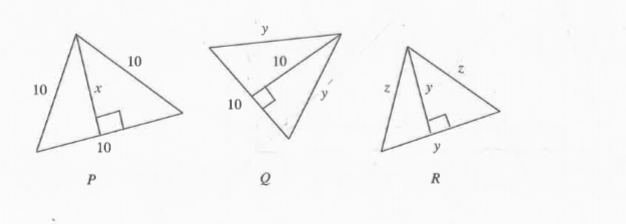 AI = II = IIIBI lt II lt IIICI gt II lt IIIDIII lt I lt II
AI = II = IIIBI lt II lt IIICI gt II lt IIIDIII lt I lt II - Question 2 - Select One
The first (ΔiH1 and second (ΔiH2 ionization enthalpies (in kJ/mol) and electron gain enthalpy (in kJ/mol) of few elements are given below:
ElementsΔiH1ΔiH2ΔegHI5207300−60II16813374−328III8991757−48IV23725251+48
Which of the following is likely to be an alkali metal?AI and IVBV and IICII and VDIV and V - Question 3 - Select One
For a given reaction, ΔH=35.5kJmol−1 and ΔS=83.6JK−1mol−1. The reaction is spontaneous at (Assume that ΔHandΔS do not vary with temperature)
AT lt 425 KBTgt 425 KCAll temperaturesDTgt 298 K
A reaction has ΔH=−33kJandΔS=−58J/K. This reaction would be:
View SolutionConsider the reaction
2NO(g)+O2(g)→2NO2(g), Predict whether the reaction is spontaneous at 298 K. ΔfG(NO)=86.69kJ/mol,ΔfG(NO2=51.84kJ/molView SolutionFrom the following values of ΔHandΔS, decide whether or not these reaction are feasible at 298 K.
Reaction A : ΔH=−10.5×103Jmol−1,ΔS=+31JK−1mol−1
Reaction B : ΔH=−11.7×103Jmol−1,ΔS=−105JK−1mol−1View SolutionFor a given reaction, ΔH=35.5KJmol−1 and ΔS=83.6JK−1mol−1. The reaction is spontaneous at: (Assume that ΔHandδS so not vary with temperature)
View SolutionFor the reaction at 25∘C,X2O4(l)→2XO2(g),ΔH=2.1kcalandΔS=20 cal K−1. The reaction would be
View Solution

