Water is flowing condinuously from a tap of area 10−4m2 the water velocity as it leaves the top is 1m/s find out area of the water stream at a distance 0.15 m below the top
0.5×10−4m2
1×10−4m2
2×10−4m2
0.25×10−4m2
The correct Answer is:A
To solve the problem of finding the area of the water stream at a distance of 0.15 m below the tap, we can follow these steps:
Step 1: Understand the Given Information
- Area of the tap (A1) = 10−4m2
- Velocity of water as it leaves the tap (V1) = 1m/s
- Distance below the tap (h) = 0.15m
- Acceleration due to gravity (g) = 10m/s2 (approximately)
Step 2: Apply the Equation of Continuity
The equation of continuity states that the product of the area and velocity at one point is equal to the product of the area and velocity at another point:
A1V1=A2V2
Where:
- A1 = area at the tap
- V1 = velocity at the tap
- A2 = area at a distance below the tap
- V2 = velocity at that distance
Step 3: Calculate the Velocity at 0.15 m Below the Tap
To find V2, we can use the following kinematic equation:
V22=V21+2gh
Substituting the known values:
V22=(1)2+2⋅10⋅0.15
V22=1+3=4
Taking the square root:
V2=√4=2m/s
Step 4: Substitute Values into the Equation of Continuity
Now that we have V2, we can find A2:
A2=A1V1V2
Substituting the values:
A2=(10−4)⋅(1)2
A2=10−42=0.5×10−4m2
Step 5: Final Answer
Thus, the area of the water stream at a distance of 0.15 m below the tap is:
A2=0.5×10−4m2
- Area of the tap (A1) = 10−4m2
- Velocity of water as it leaves the tap (V1) = 1m/s
- Distance below the tap (h) = 0.15m
- Acceleration due to gravity (g) = 10m/s2 (approximately)
The equation of continuity states that the product of the area and velocity at one point is equal to the product of the area and velocity at another point:
A1V1=A2V2
Where:
- A1 = area at the tap
- V1 = velocity at the tap
- A2 = area at a distance below the tap
- V2 = velocity at that distance
To find V2, we can use the following kinematic equation:
V22=V21+2gh
Substituting the known values:
V22=(1)2+2⋅10⋅0.15
V22=1+3=4
Taking the square root:
V2=√4=2m/s
Now that we have V2, we can find A2:
A2=A1V1V2
Substituting the values:
A2=(10−4)⋅(1)2
A2=10−42=0.5×10−4m2
Thus, the area of the water stream at a distance of 0.15 m below the tap is:
A2=0.5×10−4m2
Topper's Solved these Questions
Similar Questions
Water is flowing continuously from a tap of area 10−4m2 the water velocity as it leaves the top is 1m/s find out area of the water stream at a distance 0.15 m below the top
View SolutionWater is flowing continuously from a tap having an internal diameter 8×10−3m. The water velocity as it leves the tap is 0.4ms−1. The diameter of the water stream at a distance 2×10−1m below the tap is close to (g=10m/s2)
View Solution
Knowledge Check
Water is flowing continuously from a tap having an internal diameter 8×10−3m. The water velocity as it leves the tap is 0.4ms−1. The diameter of the water stream at a distance 2×10−1m below the tap is close to (g=10m/s2)
A5.0×10−3 mB7.5×10−3 mC9.6×10−3mD3.6×10−3 m
Water falls from a tap with A0=4m2, A=1m2 and h=2 m, then velocity v is
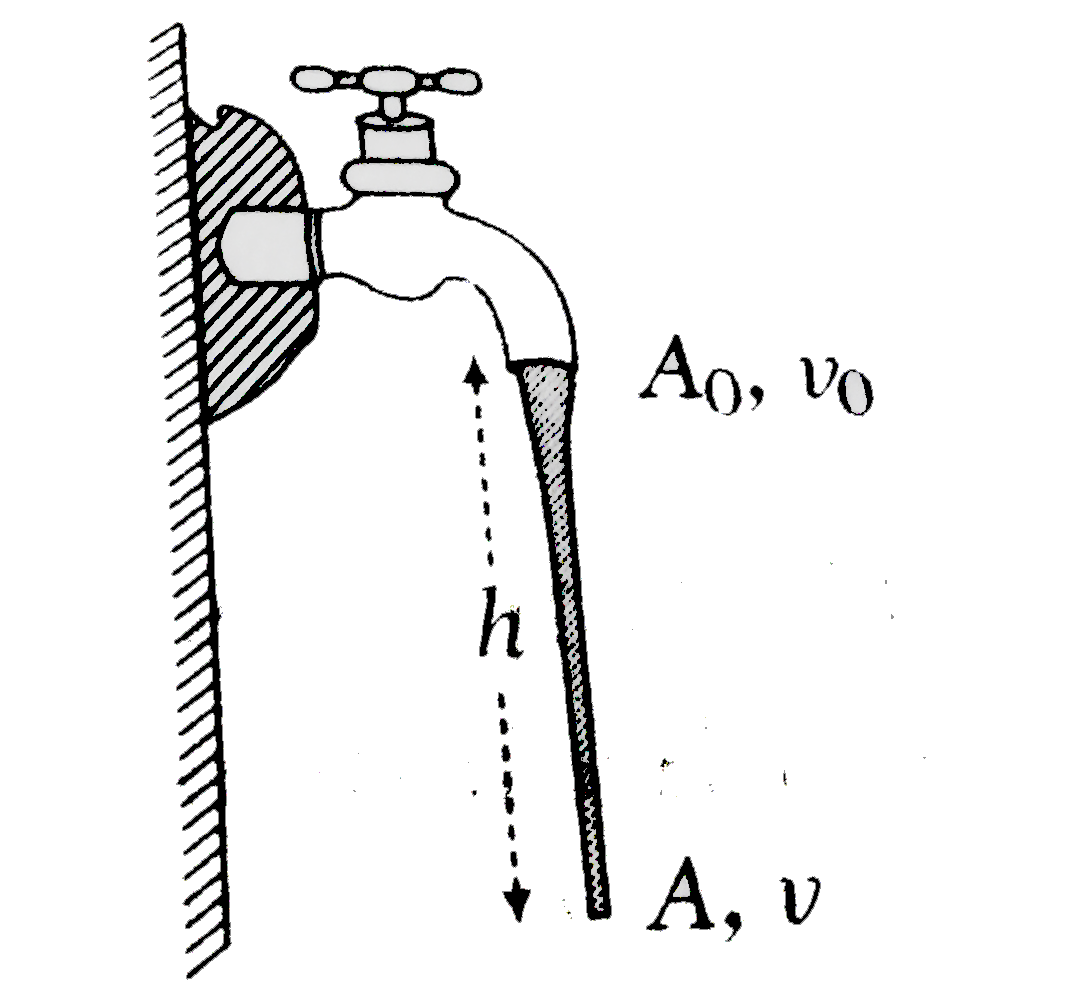
View SolutionA horizontal pipeline carries water in a streamline flow. At a point along the tube where the cross sectional area is 10−2(m2), the water velcity is 2m/s and the pressure is 8000Pa. The pressure of water at another point where cross sectional area is 0.5×(10)−2(m2) is
View SolutionA tent is in the shape of a cylinder surmounted by a conical top. If the height and diameter of the cylindrical part are 2.1 m and 4 m respectively, and the slant height of the top is 2.8 m, find the area of the canvas used for making the tent. Also find the rate of the canvas with 500 per m2
View SolutionA tent is in the shape of a cylinder surmounted by a conical top. If the height and diameter of the cylindrical part are 2.1 m and 4 m respectively, and the slant height of the top is 2.8 m, find the area of the canvas used for making the tent. Also find the rate of the canvas with 500 per m2
View SolutionWater flows into a large tank with flat bottom at the rate of 10−4m3s−1. Water is also leaking out of a hole of area 1 cm2 at its bottom. If the height of the water in the tank remains steady, then this height is :
View Solution_E01_063_Q01.png)
Water is flowing through a channel that is 12 m wide with a speed of 0.75m/s. the water then flows into four identical channels that have a width of 4.0 m the depth of the water does not change as it flows into the four channels. What is the speed of the water in one of the smaller channels?
View SolutionA pebbel is thrown horizantally from the top of a 20 m high tower with an initial velocity of 10m/s.The air drag is negligible . The speed of the peble when it is at the same distance from top as well as base of the tower (g=10m/s2)
View Solution
