A cylinder of length l and radius r is heated to temperature T. A longitudeinal compressive force F is applied on cylinder to keep its length same. Find coefficient of volume expansion.
FYtπr2
3FYtπr2
2FYtπr2
None of these
The correct Answer is:B
To find the coefficient of volume expansion of a cylinder subjected to a longitudinal compressive force while being heated, we can follow these steps:
1. Identify the Given Parameters:
- Length of the cylinder, L
- Radius of the cylinder, R
- Temperature, T
- Longitudinal compressive force, F
2. Determine the Stress on the Cylinder:
- Stress (σ) is defined as the force applied per unit area. The cross-sectional area A of the cylinder is given by:
A=πR2
- Therefore, the stress can be expressed as:
σ=FA=FπR2
3. Relate Stress to Strain Using Young's Modulus:
- Young's modulus (Y) is defined as the ratio of stress to strain:
Y=σϵ
- Where ϵ (strain) is defined as the change in length (ΔL) divided by the original length (L):
ϵ=ΔLL
4. Express Change in Length Due to Temperature:
- The change in length (ΔL) due to thermal expansion can be expressed as:
ΔL=αLT
- Here, α is the coefficient of linear expansion.
5. Substitute the Expression for Strain:
- Substituting ΔL into the strain equation gives:
ϵ=αLTL=αT
6. Combine the Equations:
- From the definition of Young's modulus, we can write:
Y=σϵ=FπR2αT
- Rearranging gives:
α=FYπR2⋅1T
7. Relate Linear Expansion to Volume Expansion:
- The coefficient of volume expansion (γ) is related to the coefficient of linear expansion (α) by the formula:
γ=3α
8. Final Expression for Coefficient of Volume Expansion:
- Substituting the expression for α into the equation for γ:
γ=3(FYπR2T)
Conclusion
The coefficient of volume expansion γ for the cylinder is given by:
γ=3FYπR2T
- Length of the cylinder, L
- Radius of the cylinder, R
- Temperature, T
- Longitudinal compressive force, F
- Stress (σ) is defined as the force applied per unit area. The cross-sectional area A of the cylinder is given by:
A=πR2
- Therefore, the stress can be expressed as:
σ=FA=FπR2
- Young's modulus (Y) is defined as the ratio of stress to strain:
Y=σϵ
- Where ϵ (strain) is defined as the change in length (ΔL) divided by the original length (L):
ϵ=ΔLL
- The change in length (ΔL) due to thermal expansion can be expressed as:
ΔL=αLT
- Here, α is the coefficient of linear expansion.
- Substituting ΔL into the strain equation gives:
ϵ=αLTL=αT
- From the definition of Young's modulus, we can write:
Y=σϵ=FπR2αT
- Rearranging gives:
α=FYπR2⋅1T
- The coefficient of volume expansion (γ) is related to the coefficient of linear expansion (α) by the formula:
γ=3α
- Substituting the expression for α into the equation for γ:
γ=3(FYπR2T)
The coefficient of volume expansion γ for the cylinder is given by:
γ=3FYπR2T
Topper's Solved these Questions
Similar Questions
The radius of a cylinder is r and heigth is h. Find its volume.
View SolutionA solid cylinder of length L and radius r is heat upto same temperature as that of a cube of edge length a. If both have same material, volume and allowed to cool under similar conditions, then ratio of amount of radiations radiated will be (Neglect radiation emitted from flat surfaces of the cylinder)
View Solution
Knowledge Check
A wire of length L and radius r is clamped at one end. On stretching the other end of the wire with a force F, the increase in its length is 1. If another wire of same material but of length 2L and radius 2r is stretched with a force 2F, the increase in its leagth will be
Al4Bl2ClD2l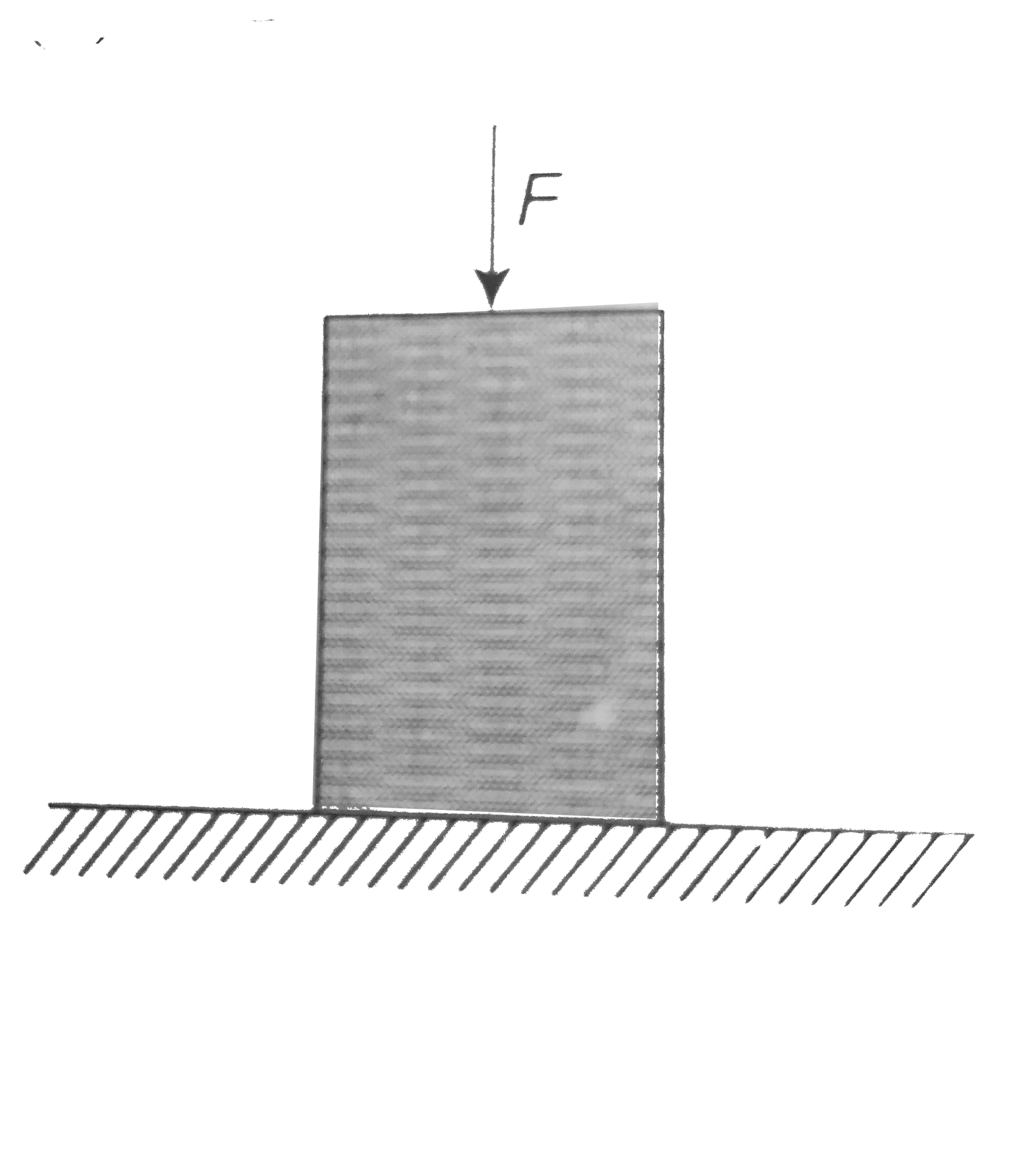
A metal cylinder of length L is subjected to a uniform compressive to a uniform compressive force F as shown in the figure. The material of the cylinder has Young's modulus Y and poisson's ratio σ The change in volume of the cylinder isAsigamFLYB(1−σ)FLYC(1+2σ)FLYD(1−2σ)FLY

A uniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force F is applied at the top point P of the cylinder so that it start pure rolling. The acceleration of the cylinder is
View SolutionUniform solid cylinder of mass m and radius R is placed on a rough horizontal surface. A horizontal constant force is applied at the top point P of the cylinder so that it start pure rolling .In the above question, the frictional force on the cylinder is
View SolutionA glass cylinder contains m0=100g of mercury at a temperature of t0=0∘C. When temperature becomes t1=20∘C the cylinder contains m1=99.7g of mercury The coefficient of volume expansion of mercury γHe=18×(10−5/∘C Assume that the temperature of the mercury is equal to that of the cylinder. The coefficient of linear expansion of glass α is
View SolutionA cylinder of same height and radius is placed on the top of a hemisphere. Find the curved surface area of the shape if the length of the shape by 7cm.
View SolutionA horizontal cylinder of length l contains water. On the left a constant force F is applied on the piston and on the right there is a small hole of area a. What is the velocity of the piston? Hence, calculate the work done in emptying the vessel in time t. brgt
 given area of cylinder is A
given area of cylinder is A
View SolutionA hole of radius r1 is made centrally in a uniform circular disc of thickness d and radius r2 . The inner surface (a cylinder of length d and radius r1) is maintained at a temperature θ1 and the outer surface (a cylinder of length d and radius r2) is maintained at a temperature θ2(θ1>θ2) .The thermal conductivity of the material of the disc is K. Calculate the heat flowing per unit time through the disc.
View Solution
