Question

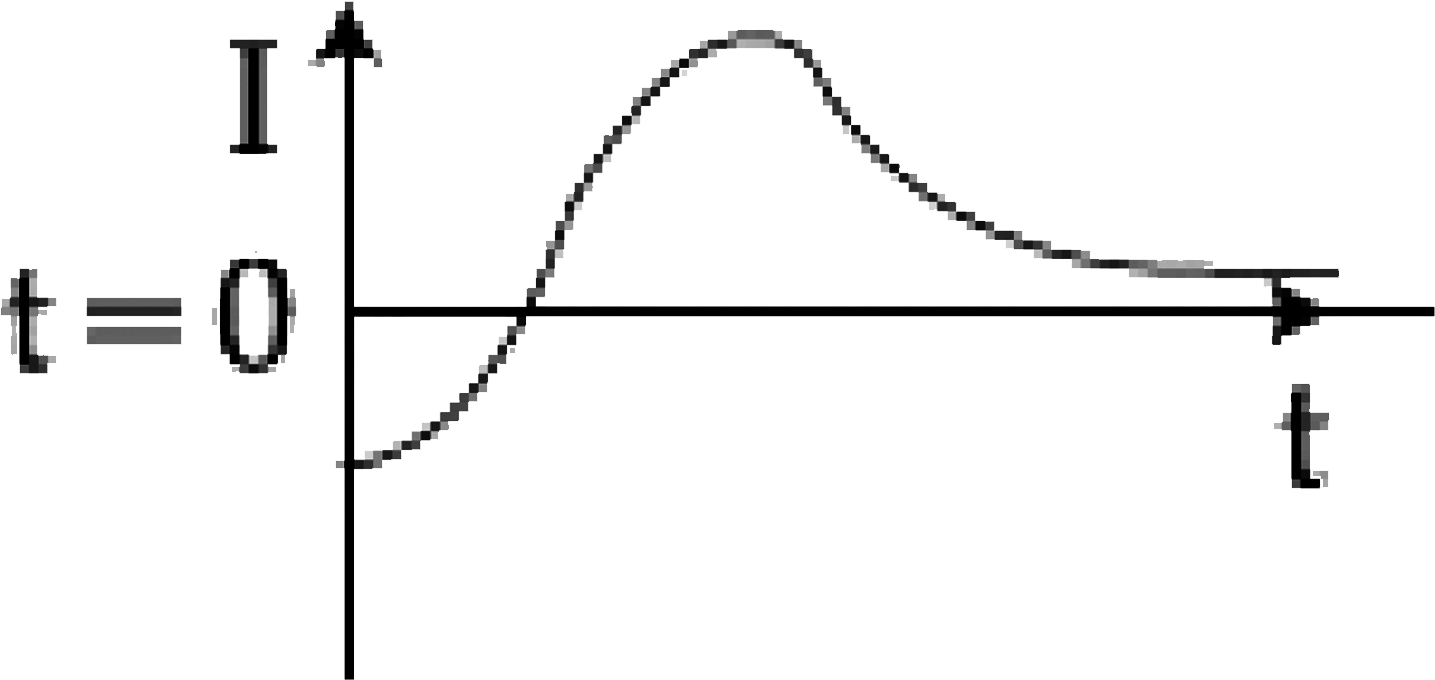
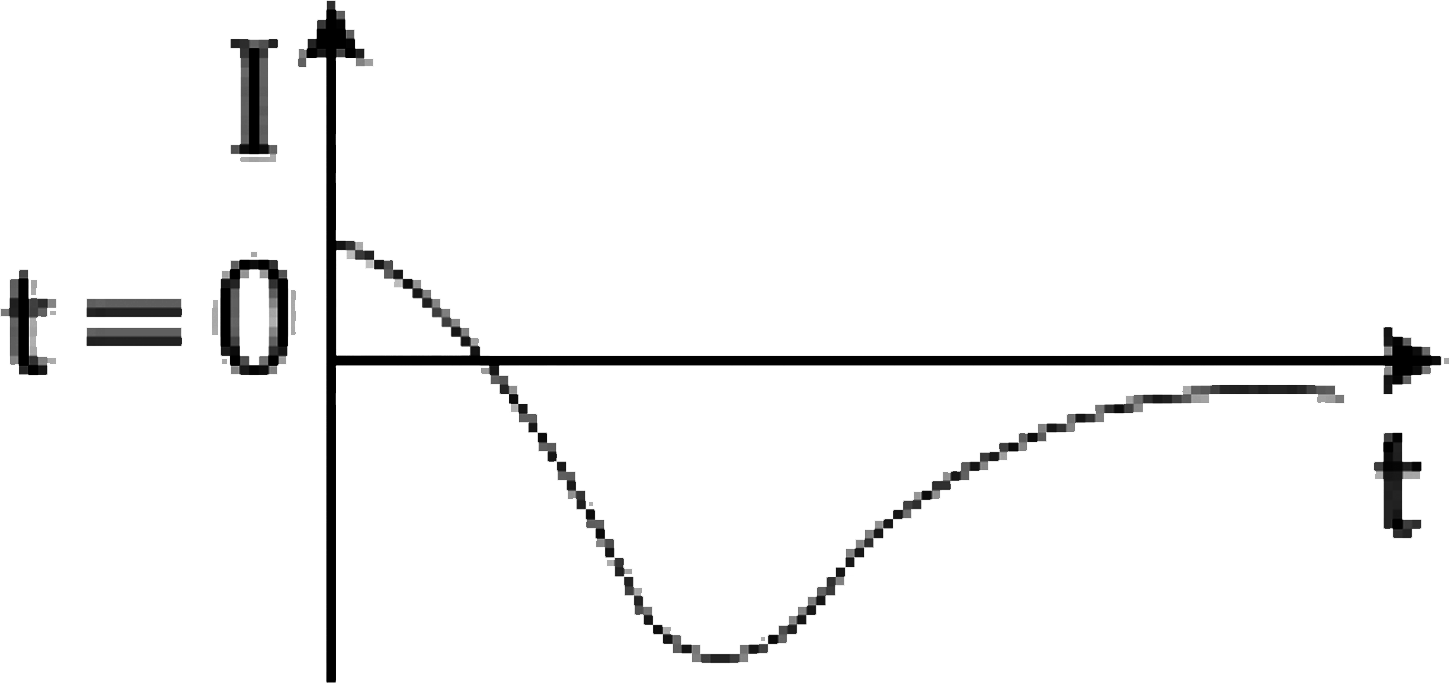
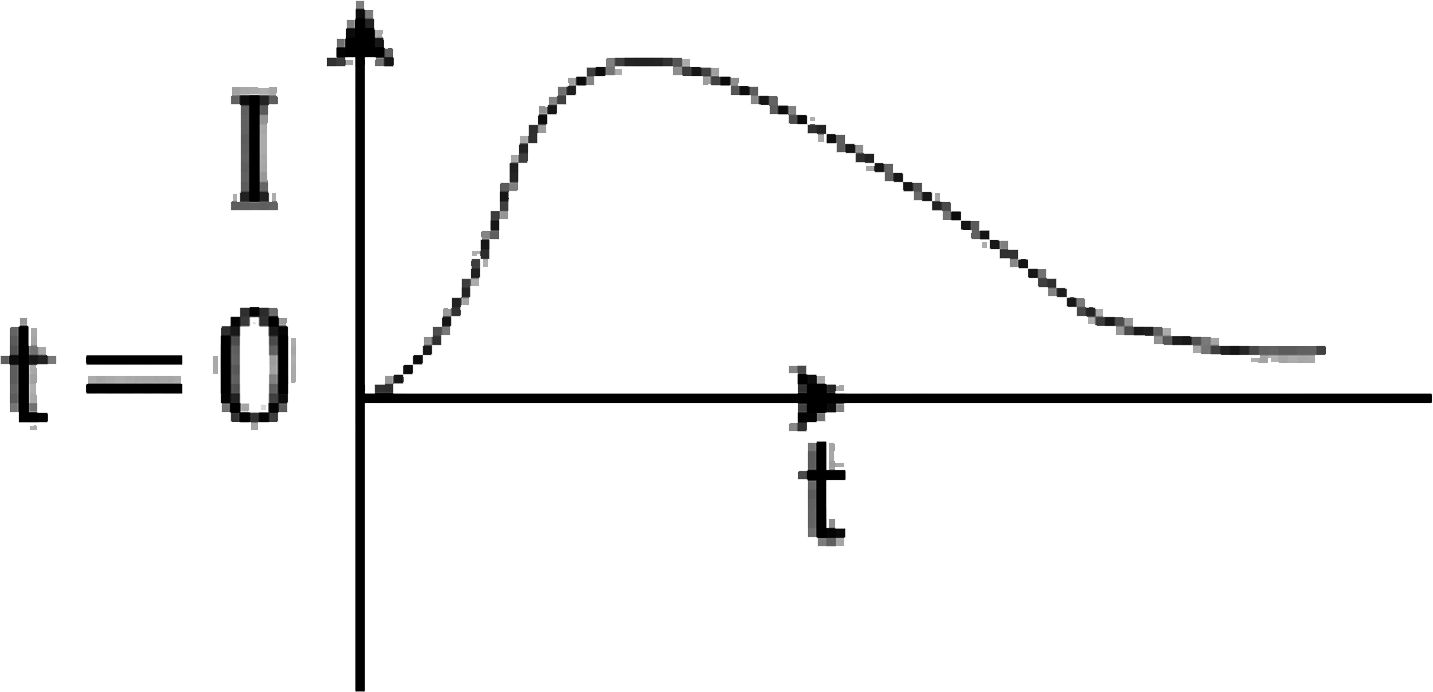
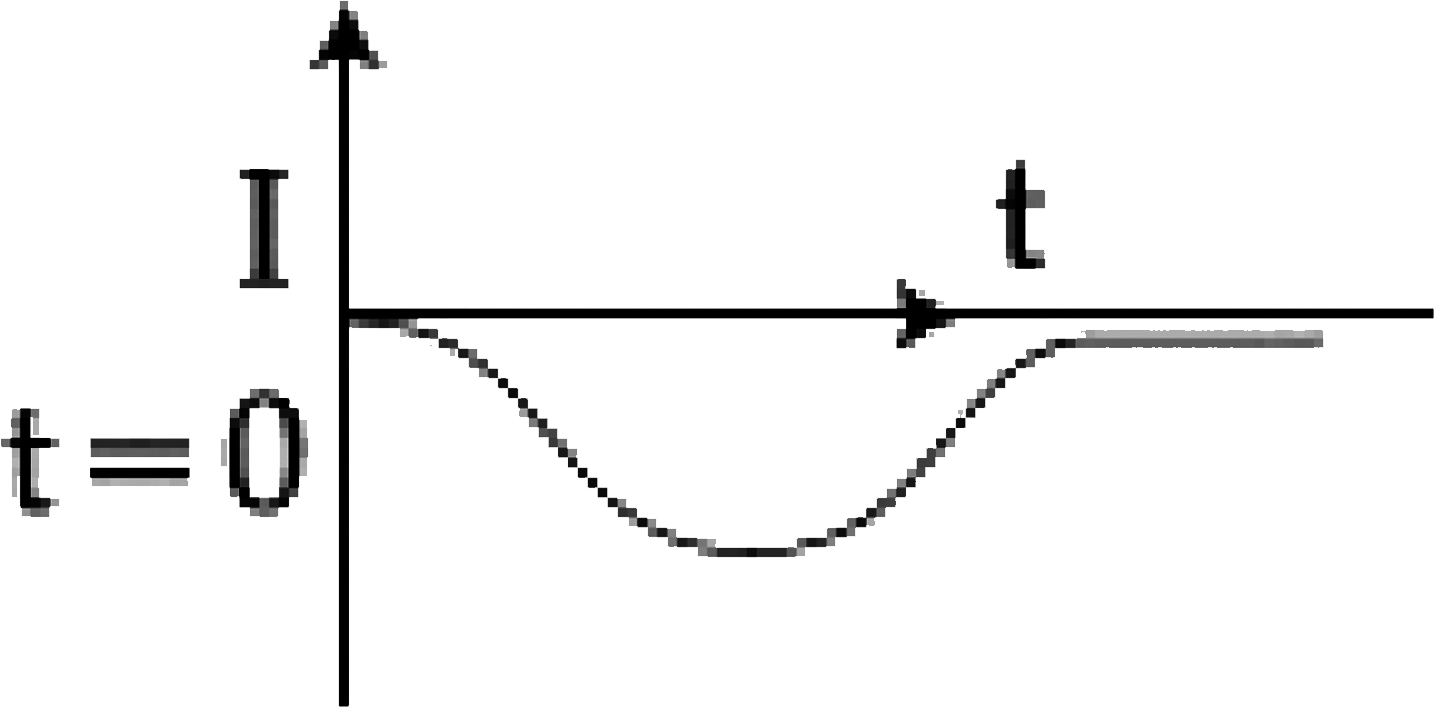
A very long solenoid of radius R is carrying current $I\left(t\right)=kt{e}^{-\alpha t}$ , as a function of time $(t\ge 0)$. Counter clockwise current is taken to be positive. A circular conducting coil of radius 2R is placed in the equatorial plane of the solenoid and concentric with the solenoid. the current induced inthe outer coil is correctly depicted, as function of time by :

Options

A.
B.
C.
D.

Answer

A

Explanation

To solve the problem, we need to analyze the situation involving a long solenoid carrying a time-dependent current and the induced current in a concentric conducting coil placed in its equatorial plane.

1. Identify the Current in the Solenoid:
The current in the solenoid is given by:
\( I(t) = k t e^{-\alpha t} \)
where \( k \) and \( \alpha \) are constants.

2. Determine the Magnetic Field Inside the Solenoid:
The magnetic field \( B \) inside a long solenoid carrying current \( I \) is given by:
\( B = \mu_0 n I \)
where \( \mu_0 \) is the permeability of free space and \( n \) is the number of turns per unit length. Since the solenoid is long and the current varies with time, we substitute \( I(t) \):
\( B(t) = \mu_0 n (k t e^{-\alpha t}) \)

3. Calculate the Magnetic Flux Through the Coil:
The area \( A \) of the circular coil of radius \( 2R \) is:
\( A = \pi (2R)^2 = 4\pi R^2 \)
The magnetic flux \( \Phi \) through the coil is given by:
\( \Phi(t) = B(t) \cdot A = \mu_0 n (k t e^{-\alpha t}) \cdot (4\pi R^2) \)
Therefore,
\( \Phi(t) = 4\pi \mu_0 n k t R^2 e^{-\alpha t} \)

4. Apply Faraday's Law of Induction:
According to Faraday's law, the induced emf \( \mathcal{E} \) in the coil is the negative rate of change of magnetic flux:
\( \mathcal{E} = -\frac{d\Phi}{dt} \)

5. Differentiate the Flux:
To find the induced emf, we differentiate \( \Phi(t) \):
\( \frac{d\Phi}{dt} = 4\pi \mu_0 n R^2 \left( k e^{-\alpha t} - k \alpha t e^{-\alpha t} \right) \)
Simplifying this gives:
\( \frac{d\Phi}{dt} = 4\pi \mu_0 n R^2 k e^{-\alpha t} (1 - \alpha t) \)
Thus, the induced emf is:
\( \mathcal{E} = -4\pi \mu_0 n R^2 k e^{-\alpha t} (1 - \alpha t) \)

6. Determine the Induced Current:
If the resistance of the coil is \( R_c \), the induced current \( I_{ind} \) can be expressed as:
\( I_{ind} = \frac{\mathcal{E}}{R_c} = -\frac{4\pi \mu_0 n R^2 k e^{-\alpha t} (1 - \alpha t)}{R_c} \)

7. Analyze the Behavior of the Induced Current:
- At \( t = 0 \):
\( I_{ind}(0) = -\frac{4\pi \mu_0 n R^2 k}{R_c} \)
- At \( t = 1/\alpha \) (where \( 1 - \alpha t = 0 \)):
\( I_{ind}(1/\alpha) = 0 \)
- As \( t \to \infty \):
\( I_{ind} \to 0 \)

Conclusion:
The induced current starts at a negative value, becomes zero at \( t = 1/\alpha \), and approaches zero as \( t \) increases. The correct depiction of the induced current as a function of time is represented by a curve that starts negative, crosses zero, and approaches zero again.