A wedge of mass M=4m lies on a frictionless plane. A particle of mass m approaches the wedge with speed v. there is no friction between the particle and the plane or between the particle and the wedge. The maximum height climbed by the particle on the wedge is given by
The correct Answer is:A
To solve the problem, we need to analyze the motion of the particle as it collides with the wedge and climbs up it. We will use the principles of conservation of momentum and conservation of energy.
1. Identify the System:
- We have a wedge of mass M=4m on a frictionless plane and a particle of mass m approaching the wedge with speed v.
2. Conservation of Momentum:
- Since there are no external forces acting on the system, the momentum before the collision must equal the momentum after the collision.
- Initial momentum pi=mv (only the particle is moving).
- Let v1 be the final velocity of both the wedge and the particle after the collision.
- The total mass after the collision is m+4m=5m.
- Thus, we have:
mv=(m+4m)v1⟹mv=5mv1⟹v1=v5
3. Conservation of Energy:
- Initially, the kinetic energy is only due to the particle:
KEi=12mv2
- After the collision, the system (particle + wedge) has kinetic energy and potential energy:
KEf=12(m+4m)v21+mgh
- Substituting v1=v5:
KEf=12(5m)(v5)2+mgh=12(5m)(v225)+mgh=mv210+mgh
4. Setting Initial Energy Equal to Final Energy:
- According to the conservation of energy:
KEi=KEf⟹12mv2=mv210+mgh
- Rearranging gives:
12mv2−mv210=mgh
- Finding a common denominator (which is 10):
5mv210−mv210=mgh⟹4mv210=mgh
5. Solving for Height h:
- Dividing both sides by mg:
h=4v210g=2v25g
Thus, the maximum height climbed by the particle on the wedge is:
h=2v25g
- We have a wedge of mass M=4m on a frictionless plane and a particle of mass m approaching the wedge with speed v.
- Since there are no external forces acting on the system, the momentum before the collision must equal the momentum after the collision.
- Initial momentum pi=mv (only the particle is moving).
- Let v1 be the final velocity of both the wedge and the particle after the collision.
- The total mass after the collision is m+4m=5m.
- Thus, we have:
mv=(m+4m)v1⟹mv=5mv1⟹v1=v5
- Initially, the kinetic energy is only due to the particle:
KEi=12mv2
- After the collision, the system (particle + wedge) has kinetic energy and potential energy:
KEf=12(m+4m)v21+mgh
- Substituting v1=v5:
KEf=12(5m)(v5)2+mgh=12(5m)(v225)+mgh=mv210+mgh
- According to the conservation of energy:
KEi=KEf⟹12mv2=mv210+mgh
- Rearranging gives:
12mv2−mv210=mgh
- Finding a common denominator (which is 10):
5mv210−mv210=mgh⟹4mv210=mgh
- Dividing both sides by mg:
h=4v210g=2v25g
h=2v25g
Topper's Solved these Questions
Similar Questions
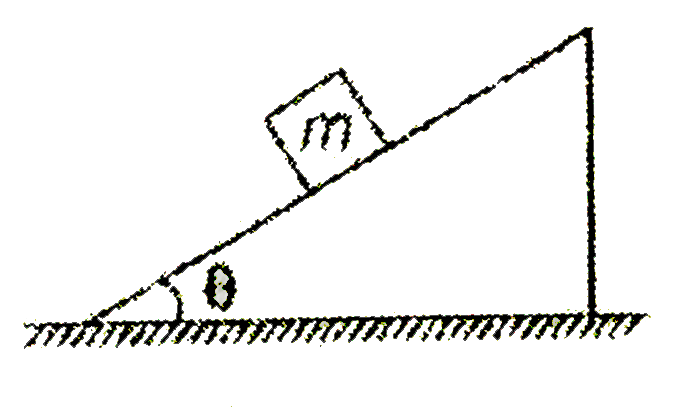
a Block of mass m is placed on a wedge of mass M there is no friction between the block and the wedge .The minmum coefficient of friction between the wedge and the gound so that wedge does not move is,
View SolutionA wedge of mass M is kept at rest on smooth surface a particle of mass m hits the wedge normally. Find the velocity of wedge and particle just after collision. Take coefficient of restitution as e.
View SolutionA wedge of mass M lies on a smooth fixed wedge of inclination θ. A particle of mass m lies on the wedge of mass M in the figure. All surfaces are frictionless and the distance of the block from the fixed wedge is l. Find.
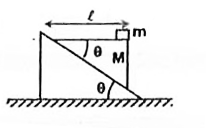
(a) Normal reaction between m and M
(b) the time after which the particle will hit the fixed plane.
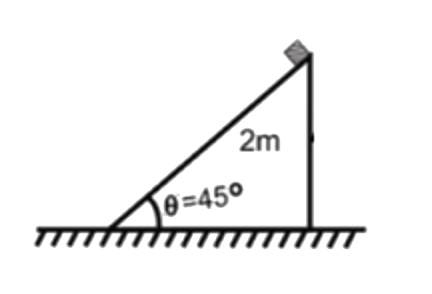
View SolutionA cube of mass m is placed on top of a wedge of mass 2m, as shown in figure. There is no friction between the cube and the wedge. The minimum co - efficient of friction between the wedge and the horizontal surface, so that the wedge does not move is
View Solution

In the situation shown in figure a wedge of mass m is placed on a rough surface, on which a block of equal mass is placed on the inclined plane of wedge.Friction coefficient between plane and the block and the ground and the wedge (μ). An external force F is applied horizontally on the wedge. Given the m does not slide on incline due to its weight. The value of F at which wedge will start slipping is:
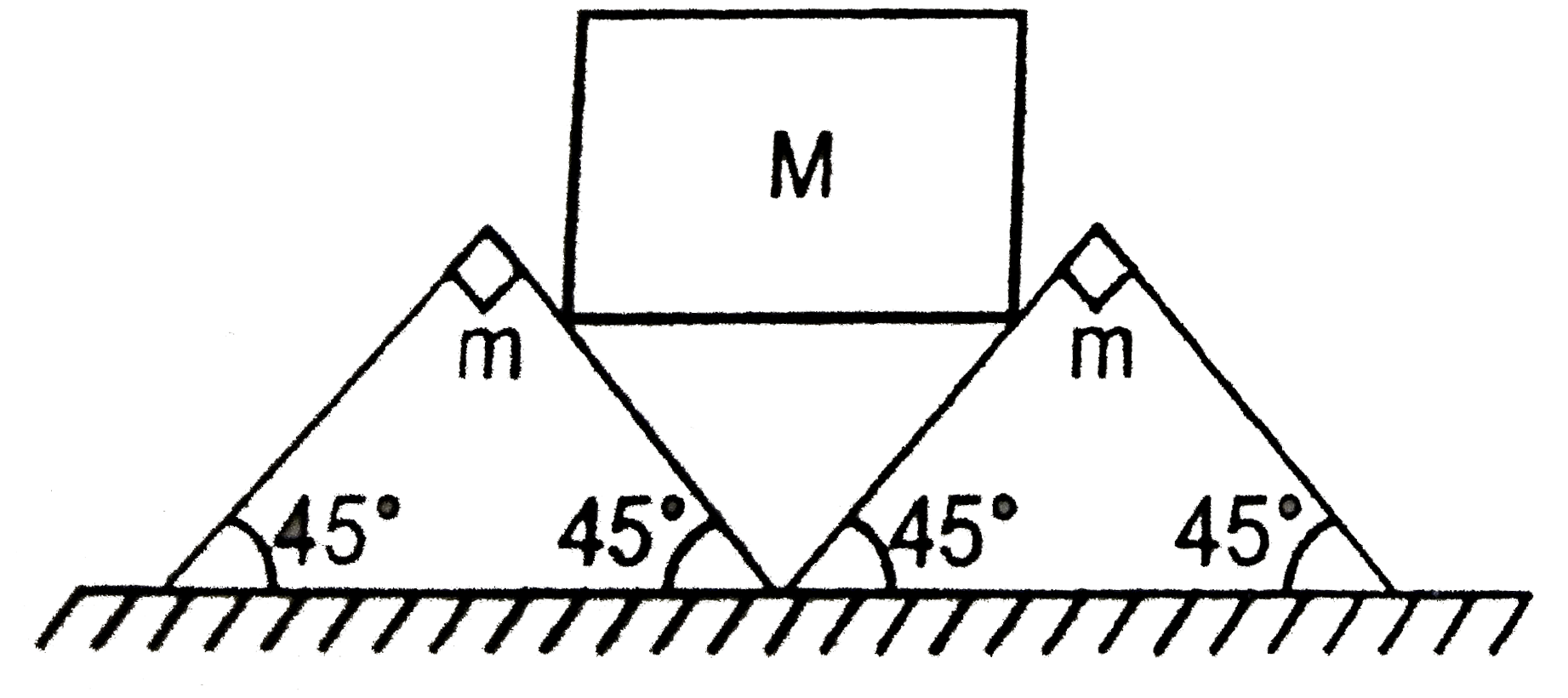
View SolutionTwo wedges, each of mass m, are placed next to each other on a flat horizontal floor. A cube of mass M is balanced on the wedges as shown in figure. Assume no friction between the cube and the wedges, but a coefficent of static friction μ<1 between the wedges and the floor. What is the largest M that can be balanced as shown without motion of the wedges ?
View SolutionIf the coefficient of friction between A and B is μ, the maximum acceleration of the wedge A for which B will remain at rest with respect to the wedge is

View SolutionIf the coefficient of friction between A and B is μ, the maximum acceleration of the wedge A for which B will remain at rest with respect to the wedge is

View SolutionA particle is projected from the ground and simultaneously a wedge starts moving towards the right, as shown in the figure. The maximum height of the wedge for which the particle will not hit the wedge is [g=10ms−2]
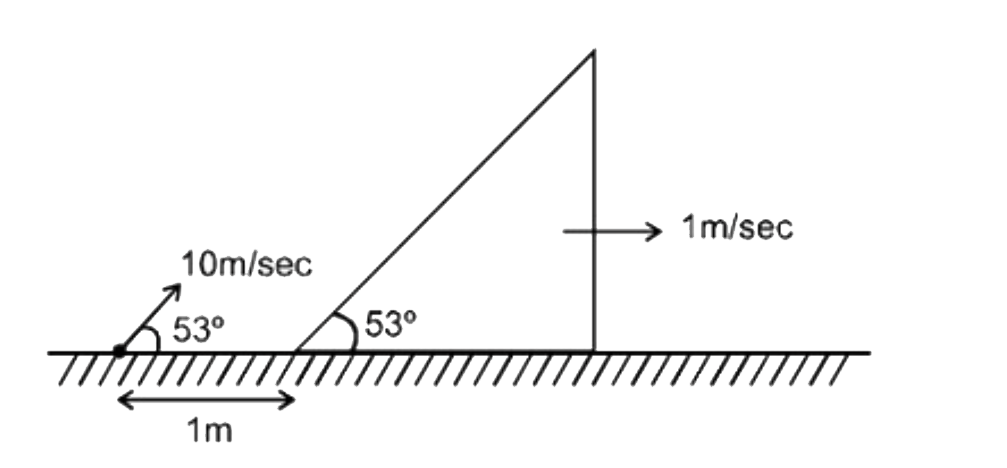
View SolutionA wedge of mass m2 is kept on a spring balance. A small block of mass m1 can move along the frictionless incline of the wedge. What is the reading of the balance while the block slides? Ignore the recoil of the wedge.

View Solution
