The ratio of surface tensions of mercury and water is given to be 7.5 while the ratio of their densities is 13.6.Their contact angles, with glass, are close to 135∘C and 0∘, respectively.It is observed that mercury gets depressed by an amount h in a capillary tube of radius r1, while water rises by the same amount h in a capillary tube of radius r2.The ratio (r1/r2), is then close to : a) 4/5 b) 2/5 c) 3/5 d) 2/3
4/5
2/5
3/5
2/3
The correct Answer is:A
To solve the problem, we need to analyze the behavior of mercury and water in capillary tubes and use the relevant formulas for capillary action.
1. Understanding Capillary Action:
- The height of liquid rise (or depression) in a capillary tube is given by the formula:
h=2γcosθρgr
where:
- h = height of rise or depression
- γ = surface tension of the liquid
- θ = contact angle with the glass
- ρ = density of the liquid
- g = acceleration due to gravity
- r = radius of the capillary tube
2. Given Data:
- Ratio of surface tensions: γHgγH2O=7.5
- Ratio of densities: ρHgρH2O=13.6
- Contact angle for mercury: θHg=135∘
- Contact angle for water: θH2O=0∘
3. Setting Up the Equations:
- For mercury (depression in capillary tube of radius r1):
h=2γHgcos135∘ρHggr1
- For water (rise in capillary tube of radius r2):
h=2γH2Ocos0∘ρH2Ogr2
4. Equating the Heights:
Since both mercury and water are observed to have the same height h:
2γHgcos135∘ρHggr1=2γH2Ocos0∘ρH2Ogr2
- Cancel g and 2 from both sides:
γHgcos135∘ρHgr1=γH2OρH2Or2
5. Substituting Values:
- cos135∘=−1√2
- Rearranging gives:
γHg(−1√2)ρHgr1=γH2OρH2Or2
6. Finding the Ratio r1r2:
Rearranging the equation:
r1r2=γHg(−1√2)ρH2OγH2OρHg
- Substituting the ratios:
r1r2=152(−1√2)⋅11⋅13.6
- Simplifying gives:
r1r2=152⋅13.6⋅√2
7. Calculating the Final Ratio:
- Evaluating the above expression numerically:
r1r2≈1527.2≈0.551
- This value is approximately 25.
Conclusion:
Thus, the ratio r1r2 is close to 25.
- The height of liquid rise (or depression) in a capillary tube is given by the formula:
h=2γcosθρgr
where:
- h = height of rise or depression
- γ = surface tension of the liquid
- θ = contact angle with the glass
- ρ = density of the liquid
- g = acceleration due to gravity
- r = radius of the capillary tube
- Ratio of surface tensions: γHgγH2O=7.5
- Ratio of densities: ρHgρH2O=13.6
- Contact angle for mercury: θHg=135∘
- Contact angle for water: θH2O=0∘
- For mercury (depression in capillary tube of radius r1):
h=2γHgcos135∘ρHggr1
- For water (rise in capillary tube of radius r2):
h=2γH2Ocos0∘ρH2Ogr2
Since both mercury and water are observed to have the same height h:
2γHgcos135∘ρHggr1=2γH2Ocos0∘ρH2Ogr2
- Cancel g and 2 from both sides:
γHgcos135∘ρHgr1=γH2OρH2Or2
- cos135∘=−1√2
- Rearranging gives:
γHg(−1√2)ρHgr1=γH2OρH2Or2
Rearranging the equation:
r1r2=γHg(−1√2)ρH2OγH2OρHg
- Substituting the ratios:
r1r2=152(−1√2)⋅11⋅13.6
- Simplifying gives:
r1r2=152⋅13.6⋅√2
- Evaluating the above expression numerically:
r1r2≈1527.2≈0.551
- This value is approximately 25.
Thus, the ratio r1r2 is close to 25.
Topper's Solved these Questions
Similar Questions
If 'M' is the mass of water that rises in a capillary tube of radius 'r', then mass of water which will ris in a capillary tube of radius '2r' is :
View SolutionMercury sticks to the walls of the capillary tube.
View Solution_2019_CBT3_E01_054_Q01.png)
mass m of a liquid rises inside a capillary of radius r. The mass of fluid that rises when a capillary of radius 2r is used is
View SolutionWater rises to a height h when a capillary of radius r is dipped in water. For another capillary of radius 2r dipped in water, it rises to the height
View SolutionWater rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
View SolutionWater rises to a height h in a capillary tube of cross-sectional area A. the height to which water will rise in a capillary tube of cross-sectional area 4A will be
View SolutionFigure shows a capillary tube of radius r dipped into water. If the atmosphere pressure is P0, the pressure at point A is
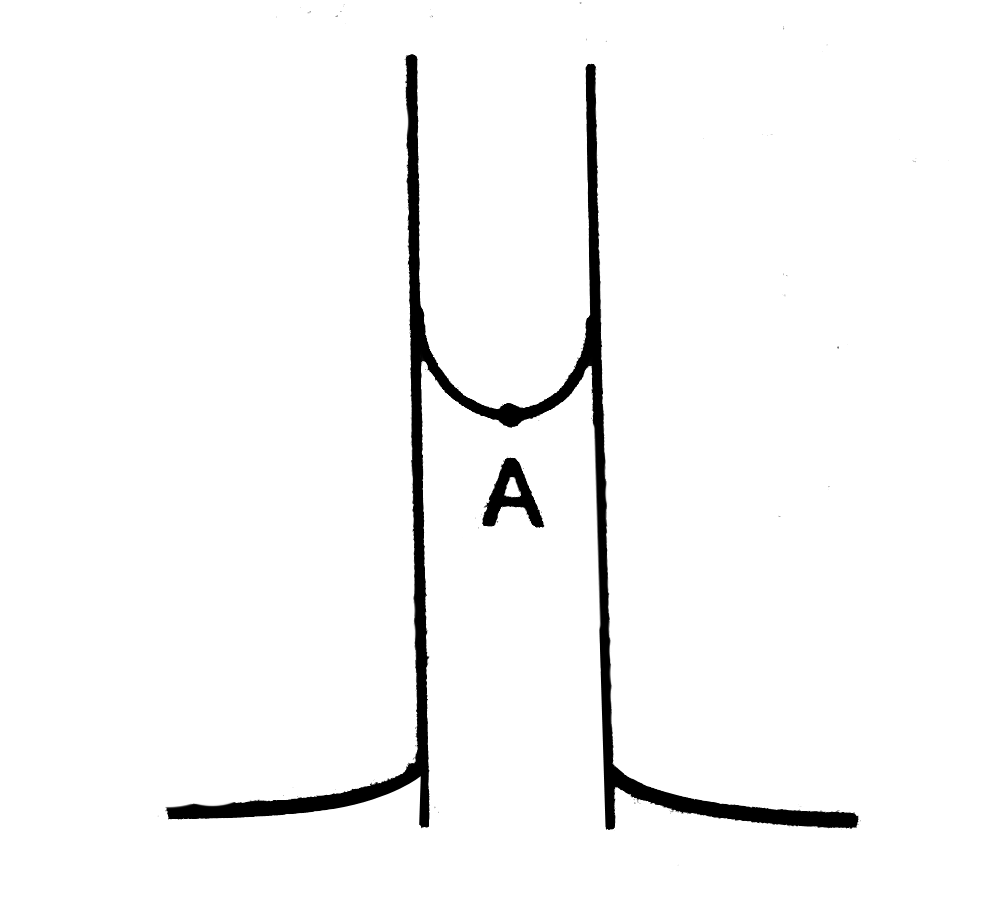
View Solution"The angle of contact of mercury with glass is obtus, while the water with glass is acute." Why?
View SolutionWater rises in a glass capillary tube, but descends if the bore of the capillary tube is coated with paraffin wax. Why ?
View SolutionThe liquid in the capillary tube will rise if the angle of contact is
View Solution
