A solid sphere of mass m& rdius R is divided in two parts of m mass 7m8&m8, and converted to a disc of radius 2R& solid sphere of radius 'r' respectively. Find I1I2 , If I1&I2 are moment of inertia of disc & solid sphere respectively
185
140
285
65
The correct Answer is:B
To solve the problem, we need to find the ratio of the moments of inertia I1 and I2 for a disc and a solid sphere, respectively. Let's break down the solution step by step.
Step 1: Calculate the Moment of Inertia of the Disc I1
The moment of inertia I of a disc about its central axis is given by the formula:
I=12mr2
In this case, the mass of the disc is 7m8 and the radius is 2R. Therefore, we substitute these values into the formula:
I1=12(7m8)(2R)2
Calculating (2R)2:
(2R)2=4R2
Now substituting back into the equation for I1:
I1=12(7m8)(4R2)=7m⋅4R216=28mR216=7mR24
Step 2: Calculate the Moment of Inertia of the Solid Sphere I2
The moment of inertia I of a solid sphere about its central axis is given by the formula:
I=25mr2
Here, the mass of the solid sphere is m8 and we need to find the radius r. We can find r by equating the densities of the original sphere and the new sphere.
The density ρ is given by:
ρ=mVolume=m43πR3
For the solid sphere of mass m8 and radius r:
ρ=m843πr3
Setting the two densities equal:
m43πR3=m843πr3
Cancelling 43π and m from both sides gives:
1R3=1/8r3
Cross-multiplying yields:
r3=R38⟹r=R2
Now substituting r=R2 into the formula for I2:
I2=25(m8)(R2)2
Calculating (R2)2:
(R2)2=R24
Substituting back into the equation for I2:
I2=25(m8)(R24)=2mR2160=mR280
Step 3: Calculate the Ratio I1I2
Now we can find the ratio of the moments of inertia:
I1I2=7mR24mR280
Cancelling mR2 from the numerator and denominator gives:
I1I2=74⋅80=5604=140
Final Answer
Thus, the ratio of the moments of inertia I1I2 is:
I1I2=140
Topper's Solved these Questions
Similar Questions
The magnitude of gravitational field at distances r1 and r2 from the centre of a uniform sphere of radius R and mass M, respectively. Find the ratio of (I1)/(I2)) if r1>R and r2>R.
View SolutionA solid sphere of mass M and radius R is pulled horizontally on a rough surface as shown in Fig. Choose the incorrect alternatives.

View SolutionThe moment of ineria (I) of a sphere of radius R and mass M is given by
View SolutionA uniform solid sphere of mass m and radius r is suspended symmetrically by a uniform thin spherical shell of radius 2r and mass m.
View Solution
A solid sphere of mass m and radius R is rolling without slipping as shown in figure. Find angular momentum of the sphere about z-axis.
View SolutionThree solid sphere of mass M and radius R are placed in contact as shown in figure. Find the potential energy of the system ?
View SolutionA solid sphere of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between sphere and plank to prevent slipping.
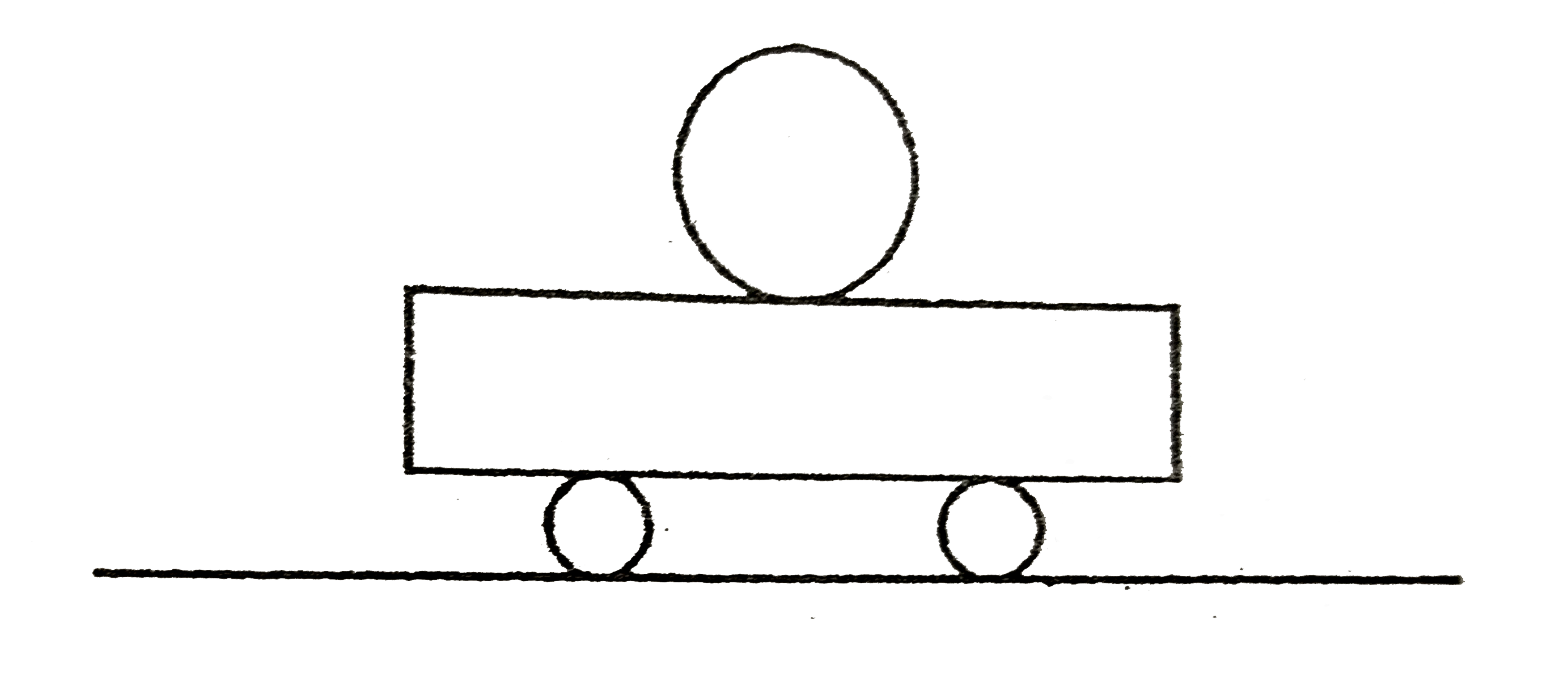
Acceleration of centre of mass of sphere is
View SolutionA solid sphere of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between sphere and plank to prevent slipping.
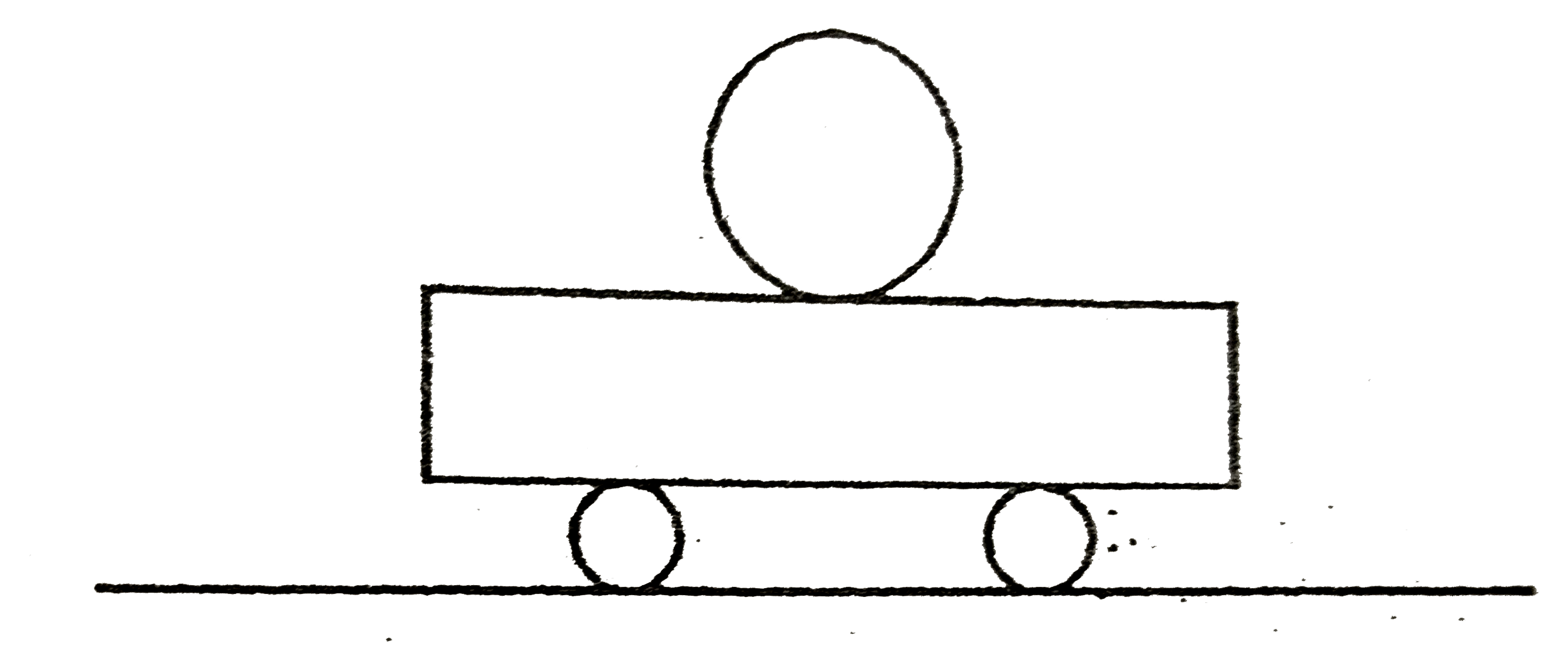
Angular acceleration of sphere is
View SolutionPercentage error in measuring the radius and mass of a solid sphere are 3 % & 1 % respectively. The error in measurement of moment of inertia about to its diameter is :-
View SolutionA solid sphere of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between sphere and plank to prevent slipping. Force of friction between sphere and plank is

View Solution
