A micture of light, consisting of wavelength 590nm and an unknown wavelength, illuminates Young's double slit and gives rise to two overlapping interference patterns on the scree. The central maximum of both lights coincide. Further, it is obseved that the third bright fringe of known light coincides with the 4th bright fringe of the unknown light. From this data, the wavelength of the unknown light is:
393.4 nm
885.0 nm
442.5 nm
776.8 nm
The correct Answer is:C
To solve the problem of finding the wavelength of the unknown light in a Young's double slit experiment, we can follow these steps:
Step 1: Understand the given information
We have two wavelengths:
- Known wavelength λ1=590nm
- Unknown wavelength λ2 (to be determined)
The central maximum of both lights coincides, and the third bright fringe of the known light coincides with the fourth bright fringe of the unknown light.
Step 2: Write the formula for the position of bright fringes
The position of the n-th bright fringe in a double-slit experiment is given by the formula:
yn=nλDd
where:
- yn is the position of the n-th bright fringe,
- n is the order of the fringe,
- λ is the wavelength of the light,
- D is the distance from the slits to the screen,
- d is the distance between the slits.
Step 3: Set up the equations for the coinciding fringes
For the known light (wavelength λ1):
- The position of the third bright fringe is:
y3=3λ1Dd
For the unknown light (wavelength λ2):
- The position of the fourth bright fringe is:
y4=4λ2Dd
Since these two positions coincide:
3λ1Dd=4λ2Dd
Step 4: Simplify the equation
We can cancel D and d from both sides of the equation:
3λ1=4λ2
Step 5: Solve for the unknown wavelength λ2
Rearranging the equation gives:
λ2=34λ1
Substituting the known wavelength λ1=590nm:
λ2=34×590nm
Step 6: Calculate λ2
Calculating this gives:
λ2=17704nm=442.5nm
Final Answer
Thus, the wavelength of the unknown light is:
λ2=442.5nm
---
We have two wavelengths:
- Known wavelength λ1=590nm
- Unknown wavelength λ2 (to be determined)
The position of the n-th bright fringe in a double-slit experiment is given by the formula:
yn=nλDd
where:
- yn is the position of the n-th bright fringe,
- n is the order of the fringe,
- λ is the wavelength of the light,
- D is the distance from the slits to the screen,
- d is the distance between the slits.
For the known light (wavelength λ1):
- The position of the third bright fringe is:
y3=3λ1Dd
- The position of the fourth bright fringe is:
y4=4λ2Dd
3λ1Dd=4λ2Dd
We can cancel D and d from both sides of the equation:
3λ1=4λ2
Rearranging the equation gives:
λ2=34λ1
λ2=34×590nm
Calculating this gives:
λ2=17704nm=442.5nm
Thus, the wavelength of the unknown light is:
λ2=442.5nm
Topper's Solved these Questions
Similar Questions
A beam of light consisting of two wavelength, 650 nm and 520 nm, is used to obtain interference fringes in a Young's double - slit experiment. Find the distance of the third bright fringe on the screen from the central maximum for wavelengths 650 nm.
View SolutionDescribe Young's double slit experiment to produce interference pattern due to a monochromatic source of light. Deduce the expression for the fringe width.
View Solution
Knowledge Check
Two sources of light of wavelengths 2500 Å and 3500 Å are used in Young’s double slit expt. simultaneously. Which orders of fringes of two wavelength patterns coincide?
A3rd order of 1st and 5th order of 2ndB7th order of 1st and 5th order of 2ndC5th order of 1st and 3rd order of 2ndD5th order of 1st and 7th order of 2ndTwo sources of light of wavelength 2500 Ã… and 3500 Ã… are used in Young's double slit experiment simultaneously. Which orders of fringes of two wavelength patterns coincide?
A3rd order of 1st source and 5th of the 2ndB7th order of 1st and 5th order of 2ndC5th order of 1st and 3rd order of 2ndD5th order of 1st and 7th order of 2ndA beam of light consisting of two wavelengths 650nm and 520nm is used to obtain interference fringes in a Young's double slit experiment.
(a) Find the distance of the third bright fringe on the screen from the central maximum for the wavelength 650nm.
(b) What is the least distance from the central maximum where the bright fringes due to both the wavelengths coincide? The distance between the slits is 2mm and the distance between the plane of the slits and screen is 120cm.A1.17 mmB2.52 mmC1.56 mmD3.14 mm
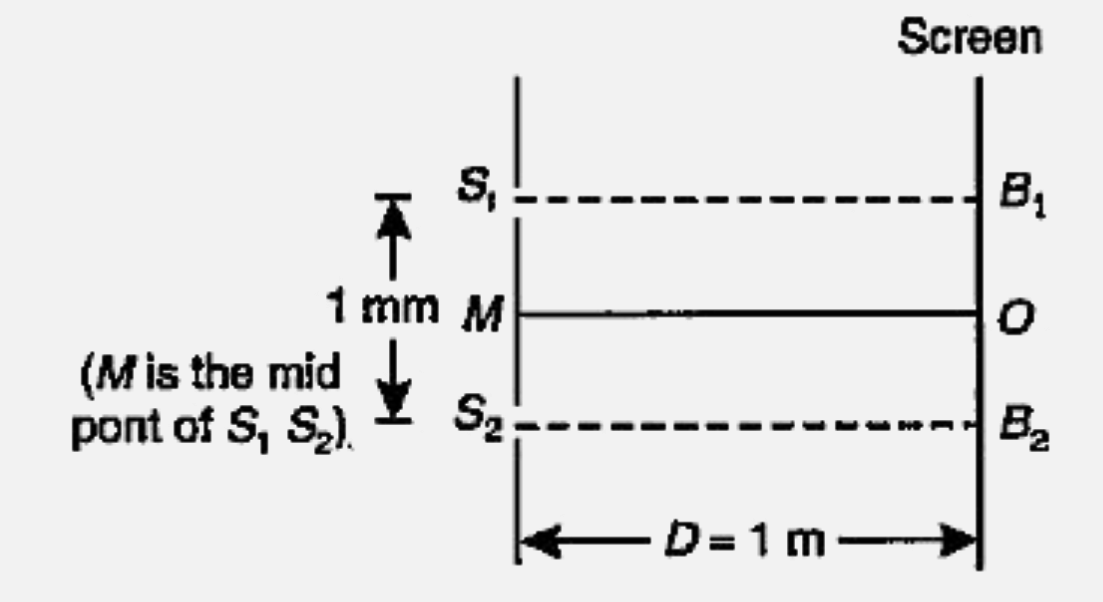
Following figure shows the positions of the first bright fringes B1andB2 on either side of the central bright fringe O in Young.s double slit experiment. Find the wavelength of monochromatic light used.
View SolutionA beam of light consisting of two wavelengths, 650 nm and 520 nm is used to obtain interference fringes in Young's double-slit experiment. What is the least distance (in m) from a central maximum where the bright fringes due to both the wavelengths coincide ? The distance between the slits is 3 mm and the distance between the plane of the slits and the screen is 150 cm.
View SolutionA beam of light consisting of two wavelengths 6500A0 and 5200A0 is used to obtain interference fringes in a Young's double slit experiment.
Find the distance of the third bright fringe on the screen from the central maximum for wavelength 6500A0. The distance between the slits is 2mm and the distance between the plane of the slits and the screen is 120 cm.
View SolutionIn Young's double-slit experiment, the slit are 0.5 mm apart and the interference is observed on a screen at a distance of 100 cm from the slits, It is found that the ninth bright fringe is at a distance of 7.5 mm from the second dark fringe from the center of the fringe pattern. The wavelength of the light used in nm is
View SolutionThe slits in Young's double slit experiment are illuminated by light of wavelength 6000 Ã…. If the path difference at the central bright fright fringe is zero, what is the path difference for light from the slits at the fourth bright frings?
View Solution
