Assume that a drop of liquid evaporates by decreases in its surface energy, so that its temperature remains unchanged. What should be the minimum radius of the drop for this to be possible? The surface tension is T, density of liquid is ρ and L is its latent heat of vaporization.
√T/ρL
T/ρL
2T/ρL
ρL/T
The correct Answer is:C
To find the minimum radius of a drop of liquid that can evaporate by decreasing its surface energy while maintaining a constant temperature, we can follow these steps:
1. Understanding the Problem:
We have a drop of liquid with radius r. When it evaporates, it loses some of its surface area, which leads to a decrease in surface energy. We need to find the minimum radius r such that this process can occur without changing the temperature of the liquid.
2. Surface Area Calculation:
The surface area A of a sphere (the drop) is given by:
A=4πr2
3. Change in Surface Area:
When the radius decreases by a small amount dr, the new radius becomes r−dr. The new surface area A′ is:
A′=4π(r−dr)2=4π(r2−2rdr+(dr)2)
The change in surface area dA is:
dA=A′−A=4π(r2−2rdr+(dr)2)−4πr2=−8πrdr+4π(dr)2
For small dr, we can neglect the (dr)2 term:
dA≈−8πrdr
4. Change in Surface Energy:
The change in surface energy dE due to the change in surface area is given by:
dE=T⋅dA=T⋅(−8πrdr)=−8πTrdr
5. Volume of Liquid Evaporated:
The volume dV of liquid that evaporates when the radius decreases from r to r−dr is:
dV=4πr2dr
The mass dm of the evaporated liquid is:
dm=ρ⋅dV=ρ⋅(4πr2dr)
6. Energy Required for Evaporation:
The energy required to evaporate this mass of liquid is given by:
dE=dm⋅L=ρ⋅(4πr2dr)⋅L
7. Equating Energy Changes:
Since the decrease in surface energy is used for the evaporation, we can set the two expressions for dE equal:
−8πTrdr=ρ⋅(4πr2dr)⋅L
Dividing both sides by dr (assuming dr≠0):
−8πTr=4πρr2L
8. Simplifying the Equation:
Cancel 4π from both sides:
−2T=ρrL
Rearranging gives:
r=2TρL
9. Conclusion:
The minimum radius r of the drop for it to evaporate by decreasing its surface energy while keeping the temperature constant is:
rmin=2TρL
We have a drop of liquid with radius r. When it evaporates, it loses some of its surface area, which leads to a decrease in surface energy. We need to find the minimum radius r such that this process can occur without changing the temperature of the liquid.
The surface area A of a sphere (the drop) is given by:
A=4πr2
When the radius decreases by a small amount dr, the new radius becomes r−dr. The new surface area A′ is:
A′=4π(r−dr)2=4π(r2−2rdr+(dr)2)
The change in surface area dA is:
dA=A′−A=4π(r2−2rdr+(dr)2)−4πr2=−8πrdr+4π(dr)2
For small dr, we can neglect the (dr)2 term:
dA≈−8πrdr
The change in surface energy dE due to the change in surface area is given by:
dE=T⋅dA=T⋅(−8πrdr)=−8πTrdr
The volume dV of liquid that evaporates when the radius decreases from r to r−dr is:
dV=4πr2dr
The mass dm of the evaporated liquid is:
dm=ρ⋅dV=ρ⋅(4πr2dr)
The energy required to evaporate this mass of liquid is given by:
dE=dm⋅L=ρ⋅(4πr2dr)⋅L
Since the decrease in surface energy is used for the evaporation, we can set the two expressions for dE equal:
−8πTrdr=ρ⋅(4πr2dr)⋅L
Dividing both sides by dr (assuming dr≠0):
−8πTr=4πρr2L
Cancel 4π from both sides:
−2T=ρrL
Rearranging gives:
r=2TρL
The minimum radius r of the drop for it to evaporate by decreasing its surface energy while keeping the temperature constant is:
rmin=2TρL
Topper's Solved these Questions
Similar Questions
If the temperature of a liquid is increased, then its surface tension
View SolutionAssume that a rain drop evaporates at a rate proportional to its surface area. Form a differential equation involving the rate of change of the radius of the rain drop.
View SolutionWhat should be the radius of a water drop so that the excess pressure inside it is 0.2 atm? Surface tension of water is 72×10−3N/m?
View SolutionOn heating a liquid, its surface tension
View SolutionIf a spherical rain drop evaporates at a rate proportional to its surface area. Form a differential equation indicating the rate of change of the radius of the rain drop.
View SolutionA spherical rain drop evaporates at a rate proportional to its surface area at any instant t. The differential equation giving the rate of change of the radius of the rain drop is _____
View SolutionA spherical rain drop evaporates at a rate proportional to its surface area at any instant t. The differential equation giving the rate of change of the radius of the rain drop is _____
View SolutionSpherical rain drop evaporates at a rate proportional to its surface area. The differential equation corresponding to the rate of change of the radius of the rain drop if the constant of proportionality is K>0 is
View SolutionA liquid of diameter D breaks up into 64 tiny drops. If the surface tension of the liquid is σ what is the change in energy?
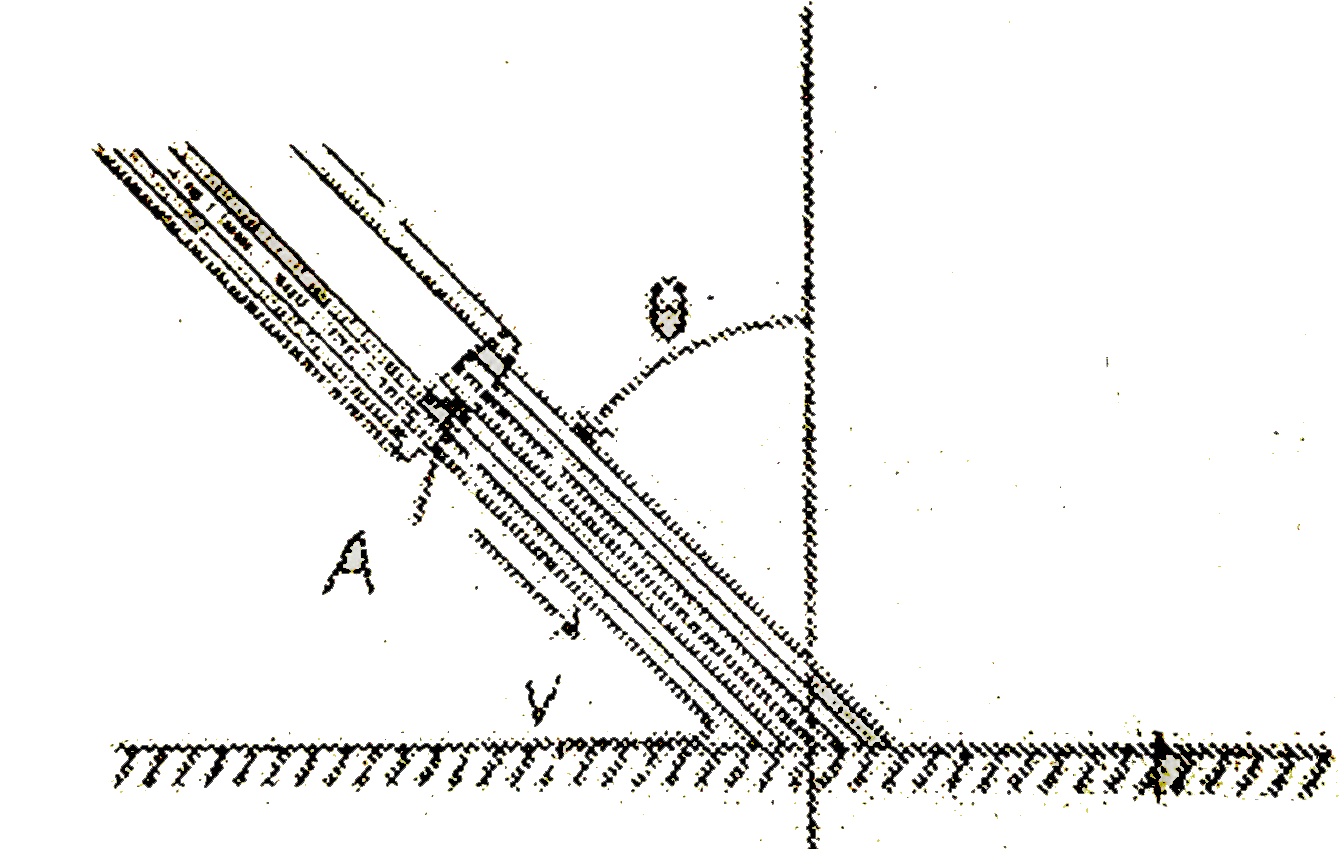
View SolutionA stream of liquid, set at an angle θ, is directed against a plane surface (figure). The liquid, after hitting the surface, spreads over it. Find the pressure on the surface. The density of the liquid is ρ and its velocity is v.
View Solution
